INTRODUCTION TO EUCLID'S GEOMETRY for Class 9 MATHS
Introduction
Toh bachoo chapter shuru karne se phle kuch baten jante hai related to the history of mathematics and the ancient world. The word ‘geometric’ , ka matlab hai earth-measure in Greek . It originated from the need for measuring land for problems faced by every ancient civilization . For example , when the river Nile overflowed , it wiped out the boundaries between the adjoining fields of different landowners . And fir uske baad boundaries ko fir se bnana padta tha . Is type ke constructions and area as well as volumes ke calculation ke liye wahan ke log bahut sare geometric techniques ka use karte the . They also calculate the volume of a truncated pyramid (solid pyramid) with base of triangle or square or some polygon and its side faces are triangles converging to a point at the top.
Euclid collected all the known work and arranged it in his famous treatise , called ‘Elements’ . He divided the ‘Elements’ into thirteen chapters , each called a book . Jisne puri duniya ki help ki or geometry ki samajh ko aasan bnaya . Now we will learn Euclid’s approach to geometry and relate them with present geometry .
Euclid’s Definitions, Axioms and Postulates
Doston kuch important facts aapko jan na bahut zaroori hai like , a solid has shape , size , position , and can be moved from one place to another . It's boundaries are called surfaces . They separate one part of the space from another and are said to have no thickness . The boundaries of the surfaces can be curved or straight lines . A solid has three dimensions , a surface has two , a line has one and a point has none .
Euclid writes 23 definitions in Book 1 of the ‘Elements’ and few of them are :
1. A point is that which has no part .
2. A line is breadth less length .
3. The ends of a line are points .
4. A straight line is a line which lies evenly with the points on itself .
5. A surface is that which has length and breadth only .
6. The edges of a surface are lines .
7. A plane surface is a surface which lies evenly with the straight lines on itself .
Euclid assumed certain properties , jinhe hume prove karne ki zarurat nahi hai . These assumptions are actually ‘obvious universal truths’ . He divided them into two types : axioms and postulates .
Postulate : Assumptions used specific to geometry .
Axioms : Assumptions used throughout mathematics and not specifically linked to geometry .
While there are statements which can be proved , using definitions , axioms , previously proved statements and deductive reasoning are called Theorems .
Some of Euclid’s axioms are :
(1) Things which are equal to the same thing are equal to one another .
(2) If equals are added to equals, the whole is equal.
Or, if A=B and B=C then A=C .
(3) If equals are subtracted from equals, the remainders are equal .
(4) Things which coincide with one another are equal to one another .
(5) The whole is greater than the part .
For example , if a quantity B is a part of another quantity A , then A can be written as the sum of B and some third quantity C . Symbolically , A > B means that there is some C such that A = B + C .
(6) Things which are double of the same things are equal to one another .
For example , if 2A=2B then we can say A=B .
(7) Things which are halves of the same things are equal to one another .
For example , if A2=B2 then we can say A=B .
Euclid’s five postulates :
Postulate 1 : A straight line may be drawn from any one point to any other point .
At least one straight line passes through two distinct points , but it does not say that there cannot be more than one such line .
Axiom 5.1 : Given two distinct points , there is a unique line that passes through them .
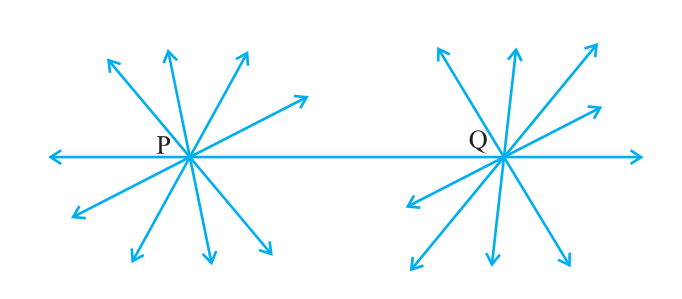
There is only one line which passes through both P and Q which is PQ .
Postulate 2 : A terminated line can be produced indefinitely .
A line segment can be extended on either side to form a line .

Postulate 3 : A circle can be drawn with any centre and any radius .
Postulate 4 : All right angles are equal to one another .
Postulate 5 : If a straight line falling on two straight lines makes the interior angles on the same side of it taken together less than two right angles , then the two straight lines , if produced indefinitely , meet on that side on which the sum of angles is less than two right angles .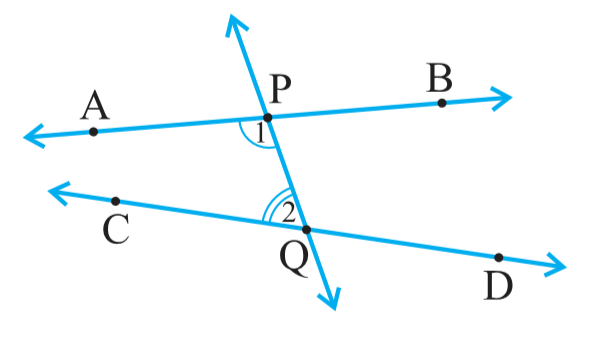
For example, maan lo ek line hai PQ jo two lines AB and CD ko kuch aise intersect karti hai jisme sum of the interior angles 1 and 2 is less than 180∘ on the left side of PQ , jaisa ki aap upar figure me dekh sakte hai . Therefore, the lines AB and CD will intersect on the left side of PQ agar hum dono lines ko aage badhate jate hai .
Note : A system of axioms is called consistent , if it is impossible to deduce from these axioms a statement that contradicts any axiom or previously proved statement .
Euclid stated his postulates and axioms , he used them to prove other statement or result by applying deductive reasoning . The statements that were proved are called propositions or theorems .
Euclid deduced 465 propositions in a logical chain using his axioms , postulates , definitions and theorems proved earlier in the chain .
Theorem 5.1
Two distinct lines cannot have more than one point in common .
Assume we have two lines l and m . We need to prove that they have only one point in common .
Man lo , two lines intersect in two distinct points , say P and Q . So , you have two lines passing through two distinct points P and Q . Lakin this assumption clashes with the axiom that only one line can pass through two distinct points . So , the assumption that we started with , that two lines can pass through two distinct points is wrong . From this we are forced to conclude that two distinct lines cannot have more than one point in common .
Example 1
In the given figure we have ∠1=∠3,∠2=∠4and∠3=∠4 prove that ∠1=∠2.
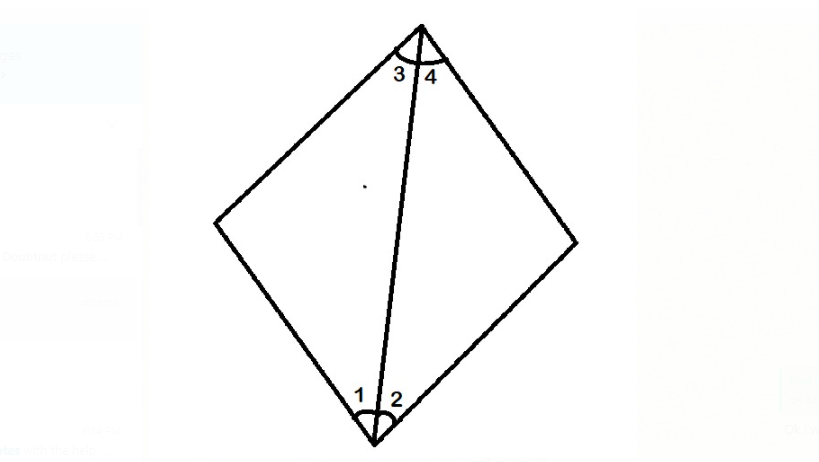
Euclid 's Axiom 1 says the things which are equal to the same things are equal to one another
∴∠1=∠3and∠3=∠4 [given]
⇒∠1=∠4 [by Euclid 's Axiom 1]
Now ∠1=∠4 [proved] and ∠2=∠4 [given]
⇒∠1=∠2 [by Euclid 's Axiom 1]
Hence ∠1=∠2
Example 2
Solve the equation x−5=15
By Euclid 's Axiom 2 we know that if equals be added to equals then the whole is equal . ∴x−5=15
we can add 5 in L.H.S and R.H.S.
⇒x−5+5=15+5
⇒x=20
Hence x=20
Example 3
In the adjoining figure , we have∠ABC=∠ACB and ∠3=∠4 . Show that BD=DC.
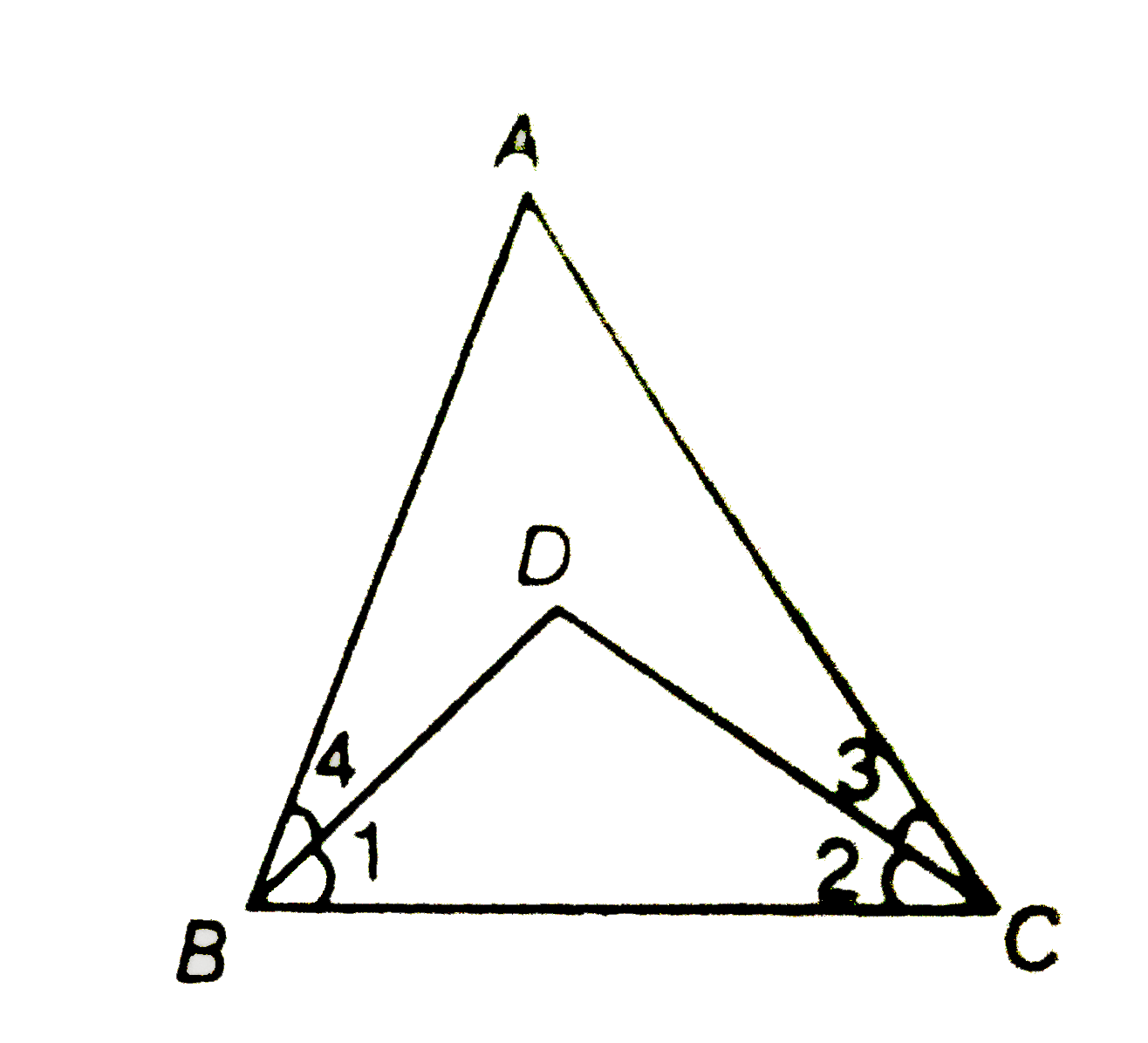
Given, ∠ABC=∠ACB ... (i)
and ∠4=∠3 ...(ii)
According to Euclid's axiom , if equals are subtracted from equals , then remainders are also equal . On subtracting Eq. (ii) From Eq.(i) we get
∠ABC−∠4=∠ACB−∠3
⇒∠1=∠2
Now , in ΔBDC,∠1=∠2
⇒ DC =BD [sides opposite to equal angle are equal]
∴ BD=DC
Equivalent Versions of Euclid’s Fifth Postulate
(i) For every line l and for every point P not lying on l , there exists a unique line m passing through P and parallel to l .
(ii)Two distinct intersecting lines cannot be parallel to the same line .
There are several equivalent versions of Euclid’s Fifth Postulate . An equivalent version of Euclid's fifth postulate and it is known as Playfair 's axiom .
(i) For every line l and for every point P not lying on l , there exists a unique line m passing through P and parallel to l .
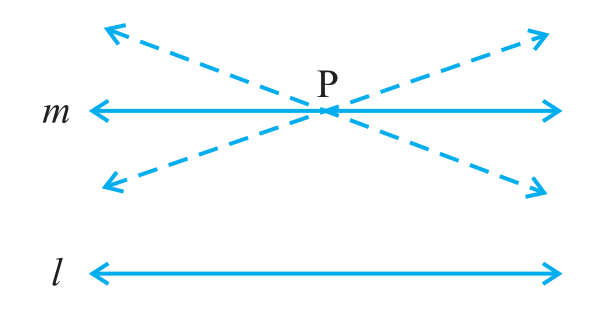
All the lines passing through the point P, only line m is parallel to line l .
(ii) Two distinct intersecting lines cannot be parallel to the same line .
Many mathematicians , including him , were convinced that the fifth postulate is actually a theorem that can be proved using just the first four postulates and other axioms. However , all attempts to prove the fifth postulate as a theorem have failed . But these efforts have led to a great achievement - the creation of several other geometries . These geometries are quite different from Euclidean geometry . They are called non-Euclidean geometries .
Summary
1. Axioms or postulates are the assumptions which are obvious universal truths . They are not proved . Theorems are statements which are proved , using definitions , axioms , previously proved statements and deductive reasoning .
2. Some of Euclid’s axioms were :
(i) Things which are equal to the same thing are equal to one another .
(ii) If equals are added to equals , the whole is equal .
(iii) If equals are subtracted from equals , the remainders are equal .
(iv) Things which coincide with one another are equal to one another .
(v) The whole is greater than the part .
(vi) Things which are double of the same things are equal to one another .
(vii) Things which are halves of the same things are equal to one another .
3. Euclid’s postulates were :
Postulate 1 : A straight line may be drawn from any one point to any other point .
Postulate 2 : A terminated line can be produced indefinitely .
Postulate 3 : A circle can be drawn with any centre and any radius .
Postulate 4 : All right angles are equal to one another .
Postulate 5 : If a straight line falling on two straight lines makes the interior angles on the same side of it taken together less than two right angles, then the two straight lines , if produced indefinitely , meet on that side on which the sum of angles is less than two right angles .
4. Two equivalent versions of Euclid’s fifth postulate are :
(i) For every line l and for every point P not lying on l , there exists a unique line m passing through P and parallel to l .
(ii) Two distinct intersecting lines cannot be parallel to the same line .