If two distinct chords drawn from the point p q on the circle x2 + y2 = px + qy where pq ≠ 0 are bisected by the x-axis thena p2 = q2b p2 = 8q2 c p2 8q2
Correct option: d p2 > 8q2Explanation:In solving a line and a circle there often generate a quadratic equation and further we have to apply condition of Discriminant so quation convert from coordinate to quadratic equation.From equation of circle it is clear that circle passes through origin. Let AB is chord of the circle.A ≡ p q. C is mid point and coordinate of C is h 0.Then coordinate of B are -p + 2h -q and B lies on the circlex2 + y2 = px + qy we haveThere are given two distinct chords which are bisected at x-axis then there will be two distinct values of h satisfying Eq. i.So discriminant of this quadratic equation must be > 0.
Related Solutions
If two distinct chords drawn from the point (p,q) on the circle x^(2)+y^(2)=px+qy (where pqne0 ) are bisected by the X- axis , then
View SolutionIf two distinct chords drawn from the point (p,q) on the circle (where are bisected by X -axis ,then
View SolutionIf two distinct chords, drawn from the point (p,q) on the circle where are bisected by x-axis then show that .
View SolutionIf two distinct chords, drawn from the point (p,q) on the circle where are bisected by x-axis then show that .
View SolutionIf two distinct chords, drawn from the point (p,q) on the circle x^(2)+y^(2)-px-qy=0 ("where pq" ne 0) are bisected by the x-axis then
View SolutionIf two distinct chords, drawn from the point (p, q) on the circle x^(2)+y^(2) =px+qy (where pq ne ~ 0) are bisected by the x-axis, then
View SolutionIf two different chords that circle from the point (p, q) x^(2) + y^(2) = px + qy = 0 (Where pq ne 0) bisects the x-axis, then
View SolutionIf two distinct chords,drawn from the point (p, q) on the circle x^(2)+y^(2)=px+qy (where pq!=q) are bisected by the x -axis,then p^(2)=q^(2)(b)p^(2)=8q^(2)p^(2) 8q^(2)
View SolutionIf two distinct chords, drawn from the point (p,q) on the circle x^(2)+y^(2)=px+qy (where pq ne 0) are bisected by the x-axis then show that, p^(2) gt 8 q^(2)
View Solution= 113400 இங்கு p1, p2, p3, p4 என்பன ஏறு வரிசையில் அமைந்த பகா எண்கள் மற்றும் x1, x2, x3, x4 என்பன முழுக்கள் எனில் p1, p2, p3, p4 மற்றும் x1, x2, x3, x4 ஆகியவற்றின் மதிப்புகளைக் காண்க.
View Solutionfind the true set of values of p for which the equation :
View SolutionIf cosec theta + cot theta = p prove that cos theta = p2-1 / p2 + 1
View Solution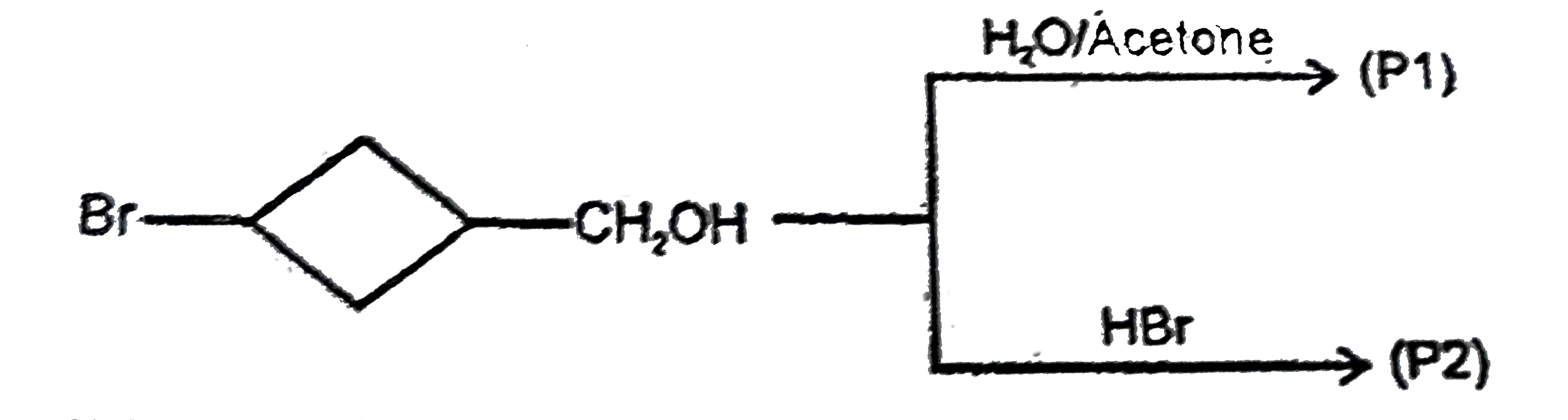
P1 and P2 are respectively
View SolutionP1 and P2 are respectively :
View Solutionacid radicals (p2)
View SolutionProperties of ITF#!#P1 & P2
View Solutionयदि दो विभिन्न जीवाएं जो बिंदु (p, q) से वृत्त (जहाँ pq , x-अक्ष द्वारा समद्विभाजित करती है, तब
View SolutionFrom power prove that for lens. P=P1+P2+P3+.........Pn
View SolutionIf two distinct chords, drawn from the point (p, q) on the circle x^2+y^2=p x+q y (where p q!=q) are bisected by the x-axis, then (a) p^2=q^2 (b) p^2=8q^2 (c) p^2 8q^2
View SolutionIf two distinct chords, drawn from the point (p, q) on the circle x^2+y^2=p x+q y (where p q!=q) are bisected by the x-axis, then p^2=q^2 (b) p^2=8q^2 p^2 8q^2
View Solution