Points 3 0 and -1 0 are invarient points under reflection in the line L1 ; point 0 -3 and 0 1 are invarient points on reflection in line L2.i Write the equation of the line L1 and L2.ii Write down the images of points P3 4 and Q-5 -2 reflection in L1. Name the image as P and Q respectively.iii Write down the images of P and Q on reflection in L2. Name the image as P and Q respectively.
i 3 0 and -1 0 lies on X-axis so these are invarient under reflection on the X-axis. Hence L1 lies on X-axies. So equation of line L1 is y =0.0 -3 and 0 1 lies on Y-axis so these are invarient under reflection on the Y-axis. So equation of line L2 is y = 0.
Related Solutions
Points (3, 0) and (-1,0) are invariant points under reflection in the line points (0, -3) and (0, 1) are invariant points on reflection in line
Write down the images of points P (3, 4) and Q (-5, -2) on reflection in Name the images as P' and Q' respectively.
View SolutionPoints (3, 0) and (-1,0) are invariant points under reflection in the line points (0, -3) and (0, 1) are invariant points on reflection in line
Write down the images of P (3,4) and Q (-5,-2) on reflection in Name the images as P" and Q" respectively.
View SolutionPoint (-5,0) and (4,0) are invariant point under reflection in the line point (0,-6) and (0,5) are invariant on reflection in the line .
Write down the image of P(2,6) and Q (-8,-3) on reflection in . Name the images as P' and Q' respectively.
View SolutionPoints (3, 0) and (-1,0) are invariant points under reflection in the line points (0, -3) and (0, 1) are invariant points on reflection in line
Name or write equations for the lines and
View SolutionPoint (-5,0) and (4,0) are invariant point under reflection in the line point (0,-6) and (0,5) are invariant on reflection in the line .
Write down the image of P and Q on reflection in . Name the images as P'' and Q'' respectively.
View SolutionPoints (3, 0) and (-1,0) are invariant points under reflection in the line points (0, -3) and (0, 1) are invariant points on reflection in line
State or describe a single transformation that maps P' onto P".
View SolutionPoints (4,0) and (-3,0) are invarient points under reflection in line point (0,5) and (0,-2) are invarient under reflection in line
Name and write the equation of lines .
View SolutionPoint (-5,0) and (4,0) are invariant point under reflection in the line point (0,-6) and (0,5) are invariant on reflection in the line .
Name or write equation for the line .
View SolutionPoints (4,0) and (-3,0) are invarient points under reflection in line point (0,5) and (0,-2) are invarient under reflection in line
Write P' . The reflection of the image of .
View Solutionif line 2x+7y-1=0 intersect the lines L1:3x+4y+1=0 and L2:6x+8y-3=0 in A and B respectively,then equation of line parallal to L1 and L2 and passes through a point P such that AP:PB=2:1 (internally) is (P is on the line 2x + 7y-1=0)
View SolutionPoint (-5,0) and (4,0) are invariant point under reflection in the line point (0,-6) and (0,5) are invariant on reflection in the line .
State or describe a single transformation that maps Q' onto Q'' .
View SolutionThe point P (2, -4) is reflected about the line x = 0 to get the image Q. The point Q is reflected about the line y = 0 to get the image R.
Find the area of figure PQR.
View SolutionPoint P (0, k) on reflection in the origin is invariant. Write the value of k.
View SolutionThe point P (2,-4) is reflected about the line x = 0 to get the image Q. Find the co-ordinates of Q.
View SolutionConsider two points A and B on L1 and a line L2 as shown in figure. Find the foot of the perpendicular drawn from (2,3,4) on L1 to the line L2.
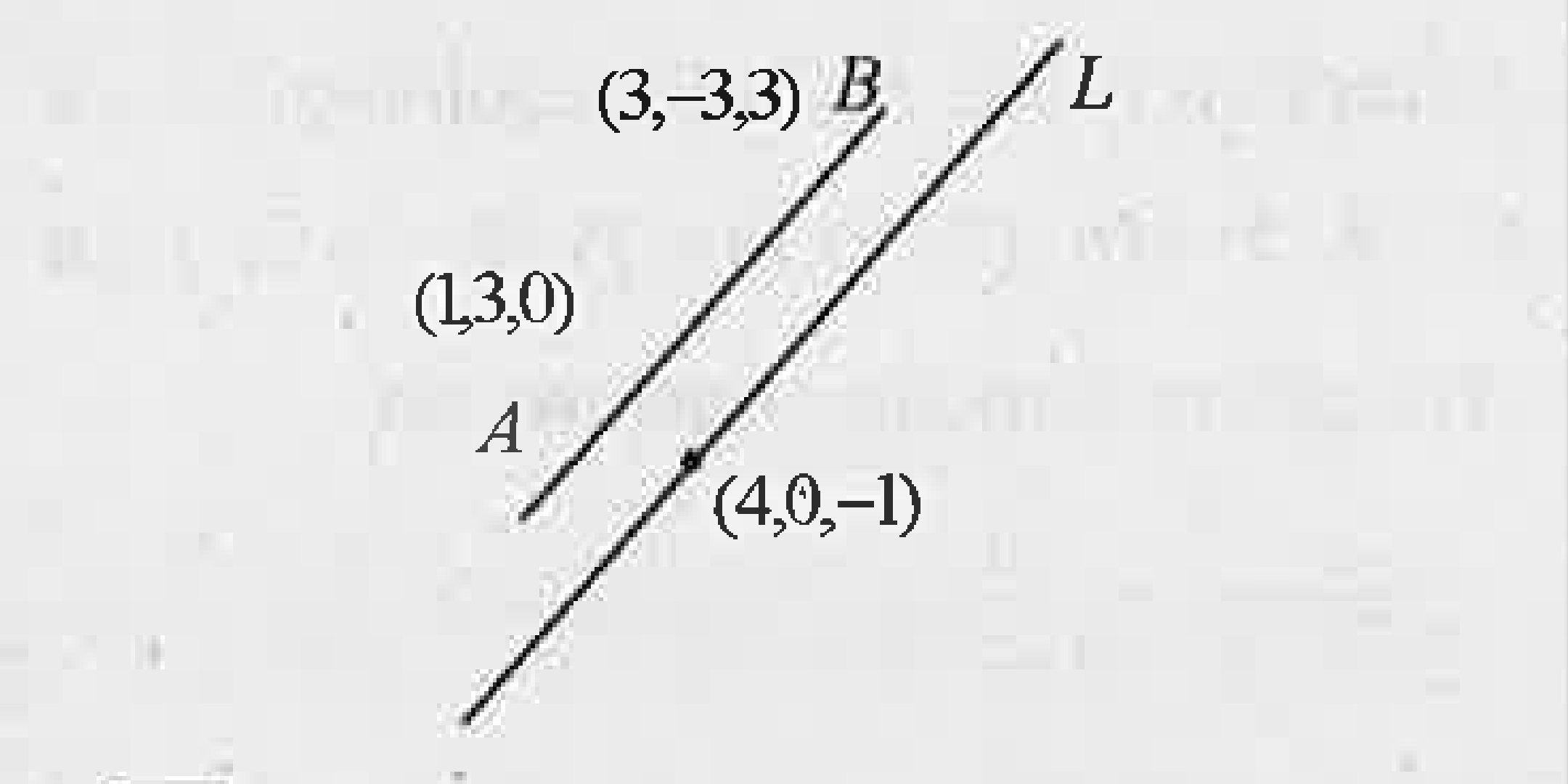
View SolutionThe points P (4, 1) and Q (-2, 4) are reflected in line y = 3. Find the co-ordinates of P', the image of P and Q', the image of Q.
View SolutionThe point P(3,6) is first reflected on the line y=x and then the image point Q is again reflected on the line y=-x to get the image point Q'. Then the circumcentre of the is
View SolutionThe point (-5,0) on reflection in a line is mappped as (5,0) and the point (-2,-6) on reflection in the same line is mapped as (2,-6)
(a) Name the line of reflection. (b) Write the co-ordinates of the image of (5,-8) in the line obtained in (a).
View SolutionThe point (-2, 0) on reflection in a line is mapped to (2, 0) and the point (5, -6) on reflection in the same line is mapped to (-5, -6).
State the name of the mirror line and write its equation.
View SolutionThe point (-2, 0) on reflection in a line is mapped to (2, 0) and the point (5, -6) on reflection in the same line is mapped to (-5, -6).
State the co-ordinates of the image of (-8,-5) in the mirror line.
View Solution