Question

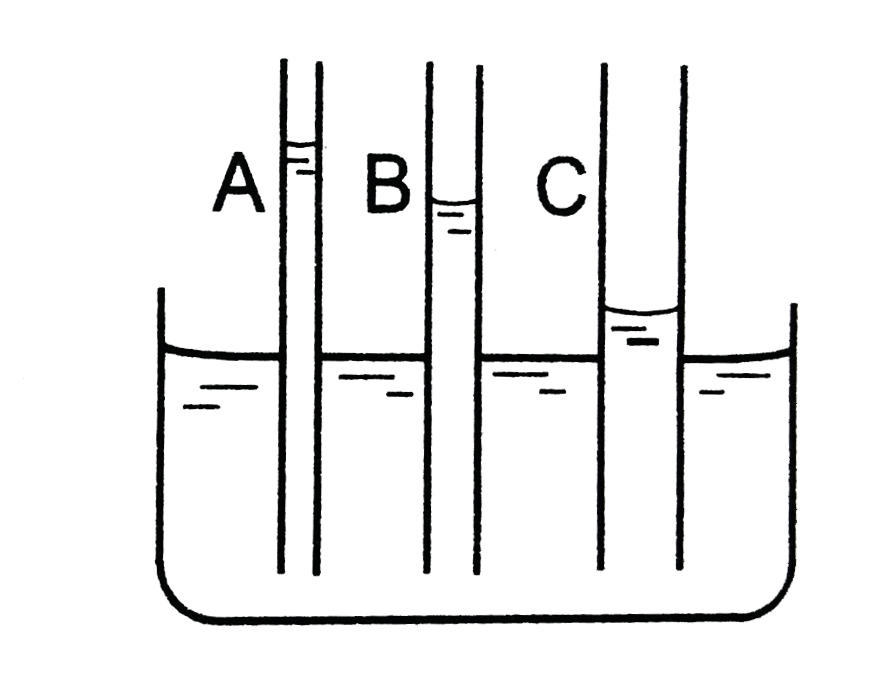
The capillaries shown in figure 14-E4 have inner radii 0.5 mm 1.0 mm and 1.5 mm respectively. The liquid in the beaker is water. Find the heights of water level in the capillaries. The surface tension of water is 7.5 x 10 -2 N/m.

Answer

The rise in a capillary = 2S.cosθ/rρg Here S = 7.5x10⁻² N/m ρ = 1000 kg/m² Let g = 10 m/s²  For water θ = 0°.  Hence for A r = 0.5 mm =0.0005 m Rise = 2* 7.5x10⁻²*cos0°/0.0005*1000*10 =15/500 m =3/100 m =3 cm  For B r = 1.0 mm =0.001 m Rise = 2* 7.5x10⁻²*cos0°/0.001*1000*10 =15/1000 m =15*100/1000 cm =1.5 cm  For C r = 1.5 mm =0.0015 m Rise = 2* 7.5x10⁻²*cos0°/0.0015*1000*10 =15/1500 m =1/100 m =1.0 cm