The charge acquired by C1 as shown in the figure is Q. The charge acquired by C2 is C1 = C2 = C3a Qb Q/2c Q/4d 2Q
Correct option: b Q/2Explanation:
Related Solutions
0.जब C1 और C2 धरिता के संधारित समंतर क्रम में संयोगजीत किए जाते हैंपरिनामी धरिता है (A) C1+C2 (B) C1C2/(C1+C2) (C) (C1+C2)/C1C2 (D)C1/C2
View Solution- View Solution
C1 and C2 are two concentric circles, the radius of C2 being twice that of C1. From a point P on C2, tangents PA and PB are drawn to C1. Then the centroid of the triangle PAB (a) lies on C1 (b) lies outside C1 (c) lies inside C1 (d) may lie inside or outside C1 but never on C1
View SolutionC1 and C2 are two concentric circles, the radius of C2 being twice that of C1. From a point P on C2, tangents PA and PB are drawn to C1. Then the centroid of the triangle PAB (a) lies on C1 (b) lies outside C1 (c) lies inside C1 (d) may lie inside or outside C1 but never on C1
View SolutionC1 and C2 are two concentric circles, the radius of C2 being twice that of C1. From a point P on C2, tangents PA and PB are drawn to C1. Then the centroid of the triangle PAB (a) lies on C1 (b) lies outside C1 (c) lies inside C1 (d) may lie inside or outside C1 but never on C1
View SolutionDelta[[ Prove that ,, 11,1,1nC1,(n+1)C1,(n+2)C1(n+1)C2,(n+2)C2,(n+3)C2]]=1
View SolutionLet conditions C1 and C2 be defined as follows: C1: b^2- 4ac > 0, C2: a, -b, c are of same sign. The roots of ax^2+ bx + c = 0 are real and positive, if (a) both C1 and C2 are satisfied (b) only C2 is satisfied (c) only C1 is satisfied (d) None of these
View SolutionAmong four cars, weight of C1 is twice the weight of C2. Weight of C3 is half of the weight of C2. C4 is 40 kg heavier than C2, but 40 kg lighter than C1. What is the weight (in kg) of C4?
View SolutionConsider two curves ; . Then, a. C1 and C2 touch each other at one point b. C1 and C2 touch each other exactly at two point c. C1 and C2 intersect(but do not touch) at exactly two point d. C1 and C2 neither intersect nor touch each other
View SolutionConsider two curves C1:y^2=4x; C2=x^2+y^2-6x+1=0. Then, a. C1 and C2 touch each other at one point b. C1 and C2 touch each other exactly at two point c. C1 and C2 intersect(but do not touch) at exactly two point d. C1 and C2 neither intersect nor touch each other
View SolutionConsider two curves C1:y^2=4x ; C2=x^2+y^2-6x+1=0 . Then, (a) C1 and C2 touch each other at one point (b) C1 and C2 touch each other exactly at two point (c) C1 and C2 intersect(but do not touch) at exactly two point (d) C1 and C2 neither intersect nor touch each other
View SolutionConsider two curves C1:y^2=4x ; C2=x^2+y^2-6x+1=0 . Then, a. C1 and C2 touch each other at one point b. C1 and C2 touch each other exactly at two point c. C1 and C2 intersect(but do not touch) at exactly two point d. C1 and C2 neither intersect nor touch each other
View SolutionFive chairs C1, C2, C3, C4 and C5 are placed one above the other. If C1 is above C2, C3 is above C4 but below C5 and C4 is above C1, which chair is in the middle?
View SolutionThe centres of two circles C1 and C2 each of unit radius are at a distance of 6 units from each other. Let P be the mid point of the line segment joining the centres of C1 and C2 and C be a circle touching circles C1 and C2 externally. If a common tangent to C1 and C passing through P is also a common tangent to C2 and C, then the radius of the circle C is
View SolutionThree charges +q, +q, +2q are arranged as shown in figure. What is the field at point P (center of side AC)
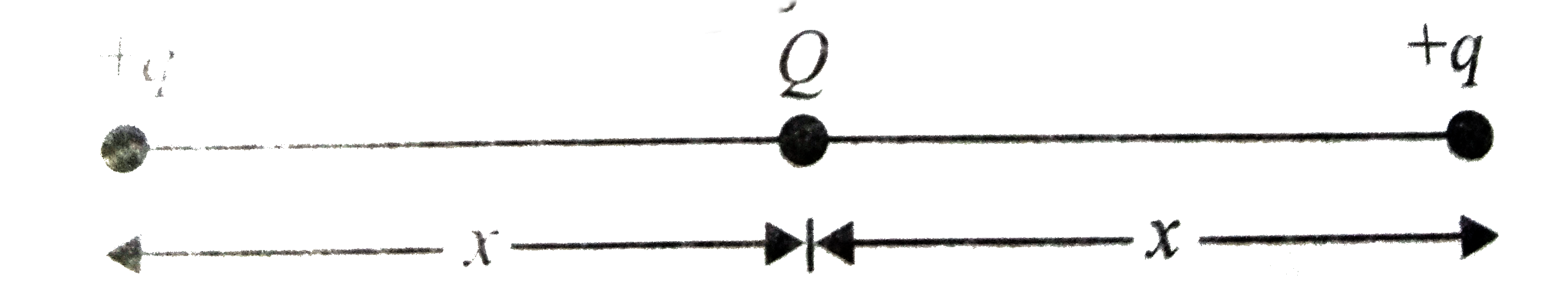
View SolutionA charge Q is placed at the centre of the line joining two point charges +q and +q as shown in figure. The ratio of charges Q and q is
 View Solution
View SolutionA charge Q is placed at the centre of the line joining two point charges +q and +q as shown in figure. The ratio of charges Q and q is
View SolutionLet and be two circles whose equations are and and is a variable point . List 1 a) P lies inside C1 but outside C2 2)P lies inside C2 but outside C1 c)P lies outside C1 but outside C2 d)P does not lie inside C2 List 2 p)λ ϵ (−∞,−1)∪(0,∞) q)λ ϵ (−∞,−1)∪(1,∞) r)λ ϵ (−1,0) s)λ ϵ (0,1)
View SolutionC0-(C1)/(2)+(C2)/(3)-............+(-1)^(n)(Cn)/(n+1)=(1)/(n+1)
View SolutionThe sum of series ^(20)C_0-^(20)C1+^(20)C2-^(20)C3++^(20)C 10 is
View Solution
