Let ABC be an isosceles triangle with AB = BC. If base BC is parallel to x-axis and m1 and m2 be the slopes of medians drawn through the angular points B and C then:a m1m2 = –1b m1 + m2 = 0c m1m2 = 2d m1 + 2m2 = 0
Correct option b m1 + m2 = 0Explanation:Let B ≡ b b1 C ≡ 3b b1 A ≡2b aand B1 and C2 be the midpoints of AC and AB respectively.
Related Solutions
A smooth ring of mass connected with a hanging block of mass by a long inextensible string, is released from rest. The ring moves vertically along a fixed rigid rod as shown in figure. Describe the motion of the ring if
(A)
(B)
(C )
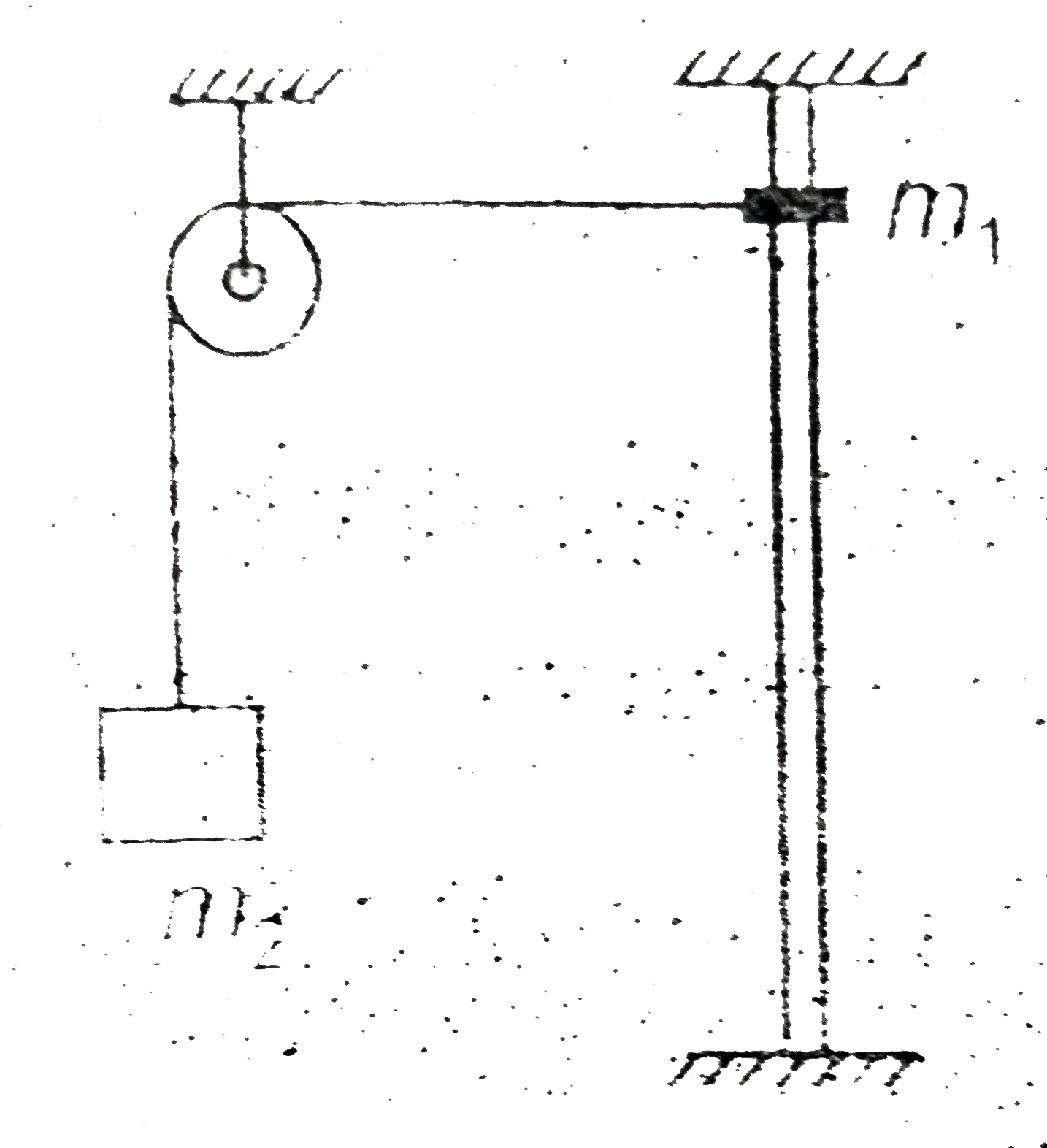 View Solution
View SolutionLet ABC be an isosceles triangle with AB=BC .If base BC is parallel to x-axis and m_(1) and m_(2) are the slopes of medians drawn through the angular points B and C, then
View SolutionLet be an isosceles triangle with If base is parallel to x-axis and are the slopes of medians drawn through the angular points then
View SolutionDiscuss different cases, to find final velocities for two bodies in the below expression for v_(1f)=((m1-m2)v1i)/(m1+m2) and v_(2f)=(2m1v1i)/(m1+m2)
View Solutionf(x)=|x-1|+|x-2|,g(x)=x+(1)/(x),x>0* if m 1=min(f(x)) and m2=min(g(x)) then (m2+m1)/(m2-m1) is equal to
View Solutiontwo tangents of a hyperbola having m1m2 cuts the axes at 4 concyclic points .find value of m1 m2
View SolutionTwo masses m1 and m2 are suspended together by a massless spring of constant k. When the masses are in equilibrium, m1 is removed without disturbing the system. The amplitude of oscillations is
 View Solution
View SolutionTwo masses m1 and m2 are suspended together by a massless spring of constant k. When the masses are in equilibrium, m1 is removed without disturbing the system. The amplitude of oscillations is
 View Solution
View SolutionTwo masses m1 and m2 are connected by light string, which passes over the top of a smooth plane inclined at 30º to the horizontal, so that one mass rests on the plane and the other hangs vertically as shown in fig. It is found that m1, hanging vertically can draw m2 up the full length of the plane in half the time in which m2 hanging vertically draws m1 up. Find m1/m2 . Assume pulley to be smooth-
 View Solution
View SolutionA partical of mass m1 is travelling with avelocity u1 strikes head-on against partical of mass m2 at rest.After collision the particales move with a velocity v1 and v2 respectively .show that the ratio of decrese in K.E of the partical of mass m1 to its original K.E is given By (4m1m2)/(m1+m2)^2
View SolutionIn the figure shown, when the persons A and B exchange their positions, then (m2>m1) the plank moves towards_________
View SolutionTwo plane mirrors M1 and M2 , placed at right angles, form two sides of a container. Mirror M1 is inclined at an angle theta_(0) to the horizontal. A light ray AB is incident normally on M1 . Now the container is filled with a liquid of refractive index mu so that the ray is first refracted, then reflected at M1 . The ray is next reflected at M2 and then comes out of the liquid surface making an angle theta with the normal to the liquid surface. Find theta .
View Solutionকোনোবস্তুর ঘনত্বের তরলে আপাত ওজন হয় , এবং , ঘনত্বের। তরলে আপাত ওজন হয় ।ওই বস্তুটির ঘনত্ব হবে।
View SolutionWhat is the maximum value of force, so that both the blocks will move together ? m1=m2=2kg ,μ=1/3
 View Solution
View SolutionIf the temperatures of samples of the same material with masses of m1 and m2 are T1 and T2, respectively, then the resulting temperature of the mixture
View SolutionDefine gravitational potential energy and derive an expression for it associated with two particles of masses m1 and m2.
View SolutionA stationary particle explodes into two particle of masses m1 and m2 and move in opposite directions with velocities v1 and v2.The ratio of their K.E is
View Solutionएक समद्विबाहु त्रिभुज ABC इस प्रकार है कि AB = AC तथा AD आधार BC की माध्यिका है तब =
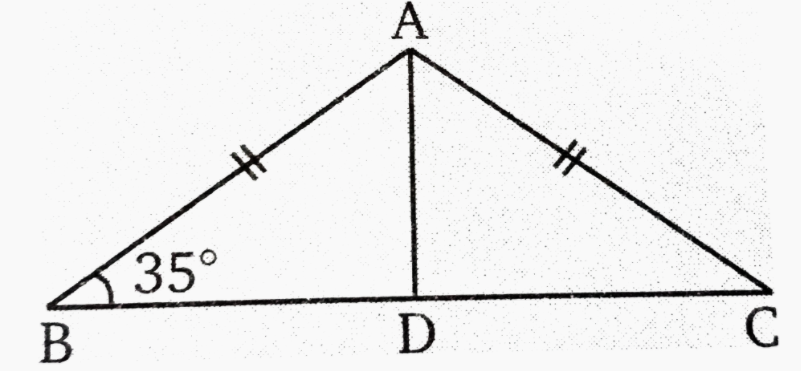 View Solution
View SolutionIn a triangle ABC, AB is parallel to y-axis, BC is parallel to x-axis, centroid is at (2, 1), If median through C is , then the slope of median through A is
View SolutionIn a triangle ABC, AB is parallel to y-axis, BC is parallel to x-axis, centroid is at (2, 1), If median through C is x-y=1, then the slope of median through A is
View Solution
