Derive an expression of Discharging of a Capacitor using RC circuits.
Again we consider the discharging of capacitor with resistance. Suppose a capacitor has a charge q0. The positive plate has a charge +q0 and negative plate –q0 . When the switch is closed the extra electrons on negative plate immediately comes to the positive plate and net charge on both plates become zero. So we can say that discharging takes place immediately.In case of C – R circuit discharging also takes time. The q – t equation in this case is Thus q decreases exponentially from q0 to zero as shown in FigIn case of discharging definition of τ is charged.At time t = τ q = q0 e–1 = 0.368 q0 Hence in this case τ can be defined as the time when charge reduces to 36.8% of its maximum value q0. During discharging current flows in the circuit till‘q’ becomes zero.This current can be found by differentiating ‘q’with respect to ‘t’ but with negative sign because charge is decreasing with time. Hence discharging current is This is an exponentially decreasing equation. Thus i – t graph decreases exponentially with time from i 0 to 0. The i – t graph is as shown in Fig.
Related Solutions
Discharging of Capacitor in a RC Circuit
View SolutionCharging and Discharging OF Capacitors||RC- Circuits||Examples
View SolutionDischarging of RC Circuits and its characteristics
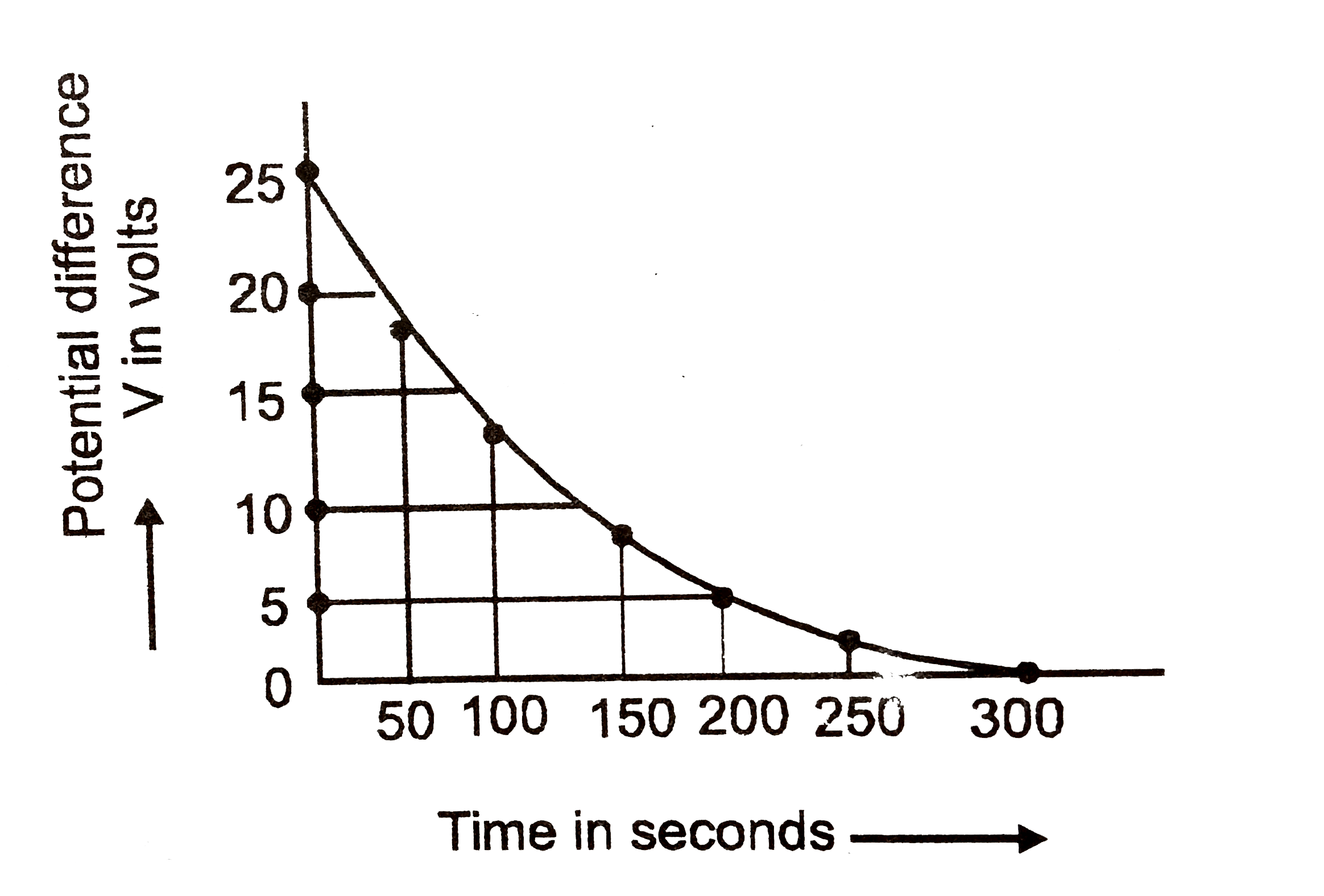
View SolutionThe figure shows an experimental plot discharging of a capacitor in an RC circuit. The time constant of this circuit lies between:
View SolutionThe figure shows an experimental plot discharging of a capacitor in an circuit. The time constant of this circuit lies between:
 View Solution
View SolutionRC Circuit #!#Charging of Capacitor
View SolutionFind the current as a function of time in case of (a) charging and (b) discharging of a capacitor in a simple RC circuit
View SolutionFind the current as a function of time in case of (a) charging and (b) discharging of a capacitor in a simple RC circuit
View SolutionHow many time constants will elapse before the charge on a capacitor falls to of its maximum value in a discharging RC circuit.
View SolutionHow many time constants will elapse before the charge on a capacitor falls to of its maximum value in a discharging RC circuit.
View SolutionHow many time constants will elapse before the charge on a capacitor falls to of its maximum value in a discharging RC circuit.
View SolutionDielectric inside capacitor and Introduction to RC Circuit
View SolutionPower supplied by battery during charging of capacitor in an RC Circuit #!#Examples based on RC Circuit
View Solutioncharging and discharging of capacitors in complex circuits
View Solutioncharging and discharging of capacitors in complex circuits
View SolutionDerive the expression for the capactive reactance and for the phase shift in an a.c. circuit containing a capacitor.
View SolutionDerive an expression for resonant frequency of series circuit containing inductor, capacitor and resistor.
View SolutionDerive the expression for the impedance of an a.c. circuit with a capacitor and a resistor in sereis.
View SolutionRC Circuit#!#Solution for RC Circuit
View SolutionR-C Circuit- Important Questions|Discharging Of A Capacitor
View Solution
