Find the point to which origin is to be shifted so as to remove the first degree terms of the equation 2x2 + 4xy - 5y2 + 20x - 22y - 14 = 0
In the given equation a = 2 h = 2 b = -5 g = 10 f = -11 c = -14 Thereforeab – h2 = -10 – 4 = - 14hf – bg = 2 -11 – -510 =- 22 + 50 = 28gh – af = 102 – 2 -11 = 42 ThereforeNew origin
Related Solutions
In order to eliminate the first degrees terms from the equation , the point to which origin is to be shifted is
View SolutionIn order to eliminate the first degree terms from the equation
The point to which origin is to be shifted is
View SolutionIn order to eliminate the first degree terms from the equation , the point to which origin is to be shifted, is
View SolutionThe point to which the origin is to be shifted to remove the first degree terms from the equation is
View SolutionFind the point to which the origin has to be shifted to eliminate x and y terms in the equation
View SolutionFind the point to which the origin has to be shifted to eliminate x and y terms in the equation
View SolutionIf the origin is shifted to (2,3) and the axes are rotated through an angle about that point, then the transformed equation of is
View SolutionFind the point to which the axes are to be translated to eliminate x and y terms (remove first degree terms) in the equation
View SolutionFind the point to which the axes are to be translated to eliminate x and y terms (remove first degree terms) in the equation 2x^(2)+4xy+5y^(2)-4x-22y+7 = 0.
View SolutionFind the point to which the axes are to be translated to eliminate x and y terms (remove first degree terms) in the equation
View SolutionIf the axes are shifted to (-2,-3) and rotated through (pi)/(4) then transformed equation of 2x^(2)+4xy-5y^(2)+20x-22y-14=0 is
View SolutionWhen the origin is shifted to (-2,-3) and the axes are rotated through an angle , find the transformed equation of .
View SolutionThe point to which the orgine is to be shifted to remove the first degree terms from the equation is
View SolutionIn order to eliminate the first degree terms from the equation then point to which origin is to be shifted is
View SolutionTo remove the first degree terms in the following equation origin should be shifted to the another point then calculate the new origins is
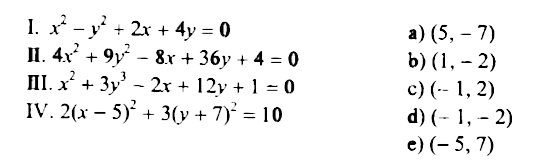
View SolutionFind the point to which the origin is to be shifted by the translation of axes so as to remove the first degree terms from the equation
where
View Solutionयदि मूलबिंदु को बिंदु पर स्थानांतरित किया जाये , तो वक्र का परिवर्तित समीकरण ज्ञात कीजिए ।
View SolutionTo remove the first degree terms from the equation where the origin shifted to the point
View SolutionThe point to which the origin should be shifted in order to eliminate x and y terms in the equation is
View SolutionTo remove the first degree terms of the equation 2xy+ 4y- 2y + 7 = 0 the shifted origin is
View Solution