A square coil of edge I having n turns carries a current i. It is kept on a smooth horizontal plate. A uniform magnetic field B exists in a direction parallel to an edge-The total mass of the coil is M. What should be the minimum value of B for which the coil will start tipping over?
Edge = l Current = i Turns= n mass = MMagnetic filed = Bτ = μBSin90° = μBMin Torque produced must be able to balance the torque produced due to weight Now τB = τ Weight
Related Solutions
A square coil of edge I having n turns carries a current i. it is kept on a smooth horizontal plate. A uniform magnetic field B exists in a direction parallel to an edge the total mass of the coil is M. What should be the minimum value of B for which the coil will start tipping over?
View SolutionA square coil of edge I having n turns carries a curent i. it is kept on a smooth horizontal plate. A uniform magnetic field B exists in a direction parallel to an edge the total mass of the coil is M. What should be the minimum value of B for which the coil will start tipping over?
View SolutionA square coil of edge I having n turns carries a curent i. it is kept on a smooth horizontal plate. A uniform magnetic field B exists in a direction parallel to an edge the total mass of the coil is M. What should be the minimum value of B for which the coil will start tipping over?
View SolutionA square coil of edge I having n turns carries a curent i. it is kept on a smooth horizontal plate. A uniform magnetic field B exists in a direction parallel to an edge the total mass of the coil is M. What should be the minimum value of B for which the coil will start tipping over?
View SolutionA square coil of edge l having turns carries a current It is kept on a smooth horizontal plate. uniform magnetic field exists in a direction parallel to an edge.The total mass of the coil is . What should be the minimum value of for which the coil will start tipping over? Given that (All physical quantities are in SI unit) ?
View SolutionA square coil with I core has n turns which give current. carries. It is kept on a smooth horizontal plane. A uniform magnetic field B is present in the direction parallel to the core. The total _mass of the coil is m. The minimum value of B for which the horoscope starts tipping is:
View SolutionA rectangular coil carrying current I is placed in a uniform magnetic field B such that the direction of B is perpendicular to the plane of the coil. Calculate the torque experienced by the coil.
View SolutionA flat coil of n turns, area A and carrying a current I is placed in a uniform magnetic field of magnitude B . The plane of the coil makes an angle theta with the direction of the field. The torque acting on the coil is
View SolutionA rectangular coil of areaand number of turns n is placed in a uniform magnetic field vecB. If the coil carries a current I, then the torque acting on the coil is given by
View SolutionWhen a current carrying coil is placed in a uniform magnetic field of induction B , then a torque tau acts on it. If I is the current, n is the number of turns and A is the face area of the coil and the normal to the coil makes an angle theta with B , Then
View SolutionA moving coil galvanometer has N number of turns in a coil of effective area. A it carries a current I. The magnetic field B is radial. The torque acting on the coil is
View SolutionA moving coil galvanometer has N numbr of turns in a coil of effective area A , it carries a current I . The magnetic field B is radial. The torque acting on the coil is
View SolutionA moving coil galvanometer has N numbr of turns in a coil of effective area A , it carries a current I . The magnetic field B is radial. The torque acting on the coil is
View SolutionA wire is parallel to a square coil . The coil and wire carry currents in the directions Shown . Then, at any point A within the coil the magnetic field will be -
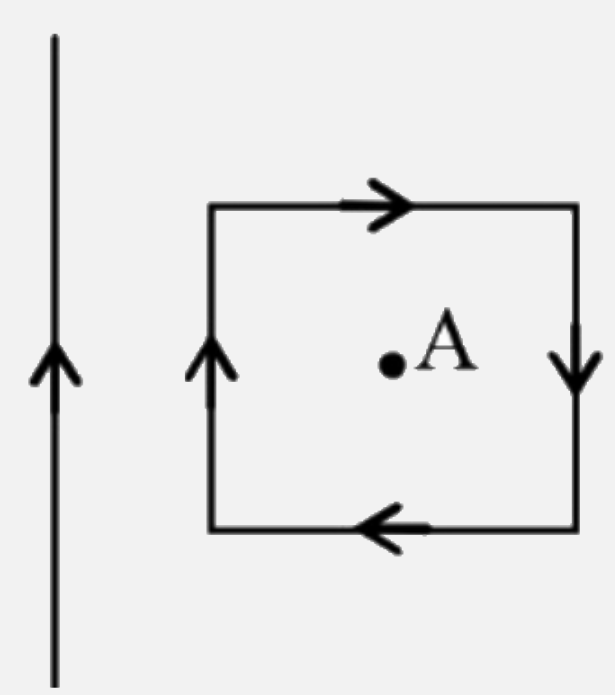
View SolutionA wire is parallel to a square coil . The coil and wire carry currents in the directions Shown . Then, at any point A within the coil the magnetic field will be -
View SolutionThe magnetic field at the centre of a circular coil carrying current I ampere is B. It the coil is bent into smaller circular coil of n turns then its magnetic field at the centre is B, the ratio between B' and B is
View SolutionThe magnetic field at the centre of a circular coil carrying current I-ampere is B. If the coil bent into smaller circular coil of n turns, its magnetic field at the centre is B. The ratio between B’ and B is:
View SolutionThe magnetic field at the centre of a circular coil carrying current I ampere is B. It the coil is bent into smaller circular coil of n turns then its magnetic field at the centre is B, the ratio between B' and B is
View SolutionA rectangular coil has turns and carries a current of . It is placed in a uniform magnetic field with the direction of magnetic field parallel to the plane of the coil. The magnitude of the torque required to hold this coil in this position is
View SolutionA rectangular coil 20cmxx20cm has 100 turns and carries a current of 1 A . It is placed in a uniform magnetic field B=0.5 T with the direction of magnetic field parallel to the plane of the coil. The magnitude of the torque required to hold this coil in this position is
View Solution