In a collinear collision a particle with an initial speed v0 strikes a stationary particle of the same mass. If the final total kinetic energy is 50% greater than the original kinetic energy the magnitude of the relative velocity between the two particles after collision is :1 √2v0 2 v0/2 3 v0/√2 4 v0/4
Ans. 1initial1/2 mv12+1/2 mv22= 3/2 1/2 mv02...1from momentum conservationmv0 = mv1 + v2 ...2v1 + v22 = v02
Related Solutions
In a collinear collision, a particle with an initial speed v0 strikes a stationary particle of the same mass. If the final total kinetic energy is 50% greater than the original kinetic energy, the magnitude of the relative velocity between the two particles, after collision, is :
View SolutionIn a collinear collision, a particle with an initial speed v0 strikes a stationary particle of the same mass. If the final total kinetic energy is 50% greater than the original kinetic energy, the magnitude of the relative velocity between the two particles, after collision, is :
View SolutionIn a collinear collision, a particle with an initial speed v0 strikes a stationary particle of the same mass. If the final total kinetic energy is 50% greater than the original kinetic energy, the magnitude of the relative velocity between the two particles, after collision, is :
View SolutionIn a collinear collision, a particle with an initial speed strikes a stationary particle of the same mass. If the final total kinetic energy is 50% greater than the original kinetic energy, the magnitude of the relative velocity between the two particles, after collision, is:
View SolutionIn a collinear collision, a particle with an initial speed strikes a stationary particle of the same mass. If the final total kinetic energy is greater than the original kinetic energy, the magnitude of the relative velocity between the two particles, after collision, is
View SolutionIn a collinear collision a particle with an intial speed v_(0) strike a stationary particle of the same mass. If the final total kinetic energy is 50 % greater than the original kinetic energy the magnitude of the relative velocity between the two particles after collision is .
View SolutionIn a collinear collision, the initial move v_(0) One particle of it collides with another paused particle of the same mass. If the total final kinetic energy is 50% higher than the initial kinetic energy, then the relative motion of the two particles after the collision will result.
View Solutionएक एकरेखीय संघट्ट (collinear collision ) में, आरम्भिक चाल का एक कण समान द्रव्यमान के एक दूसरे रुके हुए कण से टकराता है। यदि कुल अंतिम गतिज ऊर्जा , आरम्भिक गतिज ऊर्जा ज्यादा हो तो टक्कर के बाद दोनों कणों के सापेक्ष गति का प्रणाम होगा :
View SolutionTwo spheres of radii R and 2R are charged to potential V0 and 2 V0 and are connected by a battery of emf V0 as shown in figure. When switch 'S' is closed then
View SolutionA particle 1 moving with velocity experienced a head on collision with a stationary particle 2 of the same mass. As a result of collision, the kinetic energy of the system decreased by Find the magnitude and direction of the velocity of the particle 1 after collision.
View SolutionA sphere of mass M,, moving with a velocity V0 collides head on with a stationary sphere of mass M. The collision is elastic. V1 and V2 are respectively their velocities immediately after collision.
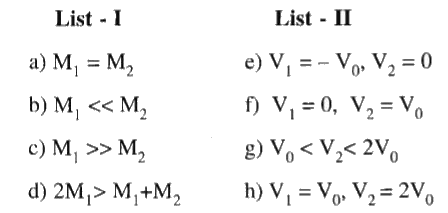
View SolutionParticle 1 moving with velocity experienced a head-on collision with a stationary particle of the same mass. As a result of the collision, the kinetic energy of the system decreased by . Find the magnitude and direction of the velocity of particle 1 after the collision.
View Solutionयदि एक कण समान द्रव्यमान से स्थिर कण को सीधी प्रत्यास्थ टक्कर करता है तो टक्कर के पश्चात् उनके वेग ज्ञात करो ?
View SolutionA particles of rest mass with kinetic energy T strikes a stationary particle of the same rest mass. Find the rest mass and the velocity of the compound particle formed as a result of the collision.
View Solutionm_P The proton of mass is the initial kinetic energy after a fully elastic collision with a heavy particle. 4/9 And if we return with the part, the mass of the heavy particle will be:
View SolutionSTATEMENT-1 : In elastic, collisions total kinetic energy is lost.
and
STATEMENT-2 : In inelastic, collisions kinetic energies of both individual particles is lost.
View SolutionSTATEMENT-1 : In elastic, collisions total kinetic energy is lost. and STATEMENT-2 : In inelastic, collisions kinetic energies of both individual particles is lost.
View SolutionIn a one-dimensional collision between two particles, their relative velocity is vecv_(1) before the collision and vecv_(2) after the collision
View SolutionA proton in motion makes head on collision with an unknown particle at rest. If the collision is perfectly elastic and proton rebounds back with 4/9 of its initial kinetic energy after collision, the mass of unknown particle is
View SolutionA particle of mass m moving with velocity v is collide with a stationary particle of mass 2 m . The speed of the system , after collision will be ……….
View Solution