In a chain of identical atoms the vibration frequency ω depends on wave number k as ω= ωmax sin ka/2 where ωmax is the maximum vibration frequency k = 2π/λ is the wave number corresponding to frequency ω a is the distance between neighbouring atoms. Making use of this dispersion relation find the dependence of the number of longitudinal vibrations per unit frequency interval on ω i.e. dN/dω if the length of the chain is l. Having obtained dN/dω find the total number N of possible longitudinal vibrations of the chain.
If the chain has N atoms we can assume atom number 0 and N +1 held ficed. Then the displacement of the nth atom has the form
Related Solutions
In a chain of identical atoms the vibration frequency omega depends on wave number k as omega= omega_(max) sin (ka//2) , where omega_(max) is the maximum vibration frequency omega , a is the distance between neighbouring atoms. Making use of this dispersion relation, find the dependence of the number of longitudinal vibrations per unit. frequency interval on omega i.e., dN//omega , if the length of the chain is l . Having obtained dN//omega , find the total number N of possible longitudinal vibrations of the chain.
View SolutionThe value of (1−ω+ω^2 )^ 5 +(1+ω−ω^ 2 )^ 5 where ω and ω^ 2 are the complex cube roots of unity, is
View SolutionIn the following circuit the equivalent resistance between A and B is
10 Ω
20 Ω
30 Ω
40 Ω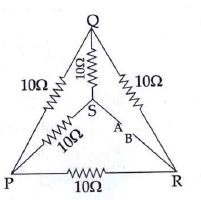
View SolutionIf are connected in parallel, what will be the current through the resistor?
View Solutioni) (1 + ω) (1 + ω ^ 4) (1 + ω ^ 8) = -ω ^ 2
View Solutionइकाई के घन मूल #!# &
View SolutionAssuming that the vibration frequency of atoms in a crystal depends on the atomic mass m, the atomic spacing a and compressibility beta , find an expression for frequency.
View SolutionIn an experiment it is observed that the frequency of a vibrating string depends on length the tension and the mass per unit length . Find out a relation for the frequency from dimensional analysis.
View SolutionWhat is the effective resistance (in Ω) of two resistors 21 Ω and 28 Ω connected in parallel?
View SolutionThe frequency of a sound depends on the .................. of the vibrating object.
View SolutionThe dimensional formula for ω in the relation y = A Sin ωt is
View SolutionThe frequency of vibration of a string depends on, (i) tension in the string (ii) mass per unit length of string, (iii) vibrating length of the string. Establish dimension the relation for frequency.
View SolutionThe frequency of vibration of a string depends of on, (i) tension in the string (ii) mass per unit length of string, (iii) vibrating length of the string. Establish dimensionally the relation for frequency.
View SolutionAssuming that the vibration frequency v of atoms in a crystal depends on the atomic mass m , the atomic spacing alpha and compressibilty beta , find an expression for frequency.
View SolutionThree resistors of are given in the class.a) What is the highest resistance that you can get using all of them? b) What is the least resistance that you can get using all of them? c) Can you make a resistance using these three? Draw the circuit.
View SolutionA girl has many resistors of 2 Ω 9 Ω resistance. For this draw a cicuit with the minimum number of resistors.
View SolutionEstimation of frequency of a wave forming a standing wave represented by can be done if the speed and wavelength are known using . Speed of motion depends on the medium properties namely tension in string and mass per unit length of string . A string may vibrate with different frequencies . The corresponding wavelength should be related to the length of the string by a whole number for a string fixed at both ends . Answer the following questions:
Speed of a wave in a string forming a stationary wave does not depend on
View SolutionEstimation of frequency of a wave forming a standing wave represented by y = A sin kx cos t can be done if the speed and wavelength are known using speed = "Frequency" xx "wavelength" . Speed of motion depends on the medium properties namely tension in string and mass per unit length of string . A string may vibrate with different frequencies . The corresponding wavelength should be related to the length of the string by a whole number for a string fixed at both ends . Answer the following questions: Speed of a wave in a string forming a stationary wave does not depend on
View SolutionIf the time interval of the vibrating object is 0.04 seconds, then the frequency of the wave will be _____.
View SolutionHigh frequency sound waves vibrate basilar membrane
View Solution