यदि सारणिक ∣∣ ∣ ∣∣1+sin2xcos2xsin2xsin2x1+cos2xsin2xsin2xcos2x1+sin2x∣∣ ∣ ∣∣ का अधिकतम तथा न्यूनतम मान क्रमशः α तथा β है तब
α+β99=4
α3−β17=26
n∈N के लिए (α2n−β2n) हमेशा एक सम पूर्णाक है
एक त्रिभुज बनाया जा सकता है जिसकी भुजाएँ α−β,α+β तथा α+3β है
The correct Answer is:A, B, C
Step by step video & text solution for यदि सारणिक |(1+sin^(2)x,cos^(2)x,sin2x),(sin^(2)x,1+cos^(2)x,sin2x),(sin^(2)x,cos^(2)x,1+sin2x)| का अधिकतम तथा न्यूनतम मान क्रमशः alpha तथा beta है तब by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- सारणिक तथा आव्यूह BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - JEE ADVANCED MORE THAN ONE CORRECT ANSWERS (Comprehension type questions) (Passage-I)
6 Videos
- सारणिक तथा आव्यूह BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - JEE ADVANCED MORE THAN ONE CORRECT ANSWERS (Comprehension type questions) (Passage-III)
6 Videos
- सारणिक तथा आव्यूह BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - EXERCISE
262 Videos
- सरल रेखा BOOK - ERRORLESS HINDICHAPTER - सरल रेखा EXERCISE - JEE ADVANCE (Matrix Match type questions)
4 Videos
Similar Questions
माना कि p एक विषम अभाज्य संख्या है तथा Tp,2×2 आव्यूहों का निम्न समुच्चय है
Tp={A=[abca]:a,b,c∈{0,1,2,.............p−1}}
Tp में ऐसे A की संख्या जो सममित या विषम सममित अथवा दोनों प्रकार के हो एवं जिनके लिए det(A),p से विभाज्य है, निम्न है
View Solutionमाना A=⎡⎢⎣100210321⎤⎥⎦ तथा U1,U2 व U3,3×3 आव्यूह U के कॉलम है। यदि कॉलम आव्यूह U1,U2 तथा U3,AU1=⎡⎢⎣100⎤⎥⎦,AU2=⎡⎢⎣230⎤⎥⎦,AU3=⎡⎢⎣231⎤⎥⎦ को संतुष्ट करते है, तब निम्न प्रश्नो के उत्तर दीजिए
|U| का मान है
View Solution∣∣ ∣ ∣∣111cos(nx)cos(n+1)xcos(n+2)xsin(nx)sin(n+1)xsin(n+2)x∣∣ ∣ ∣∣ निर्भर नहीं करता है
View Solution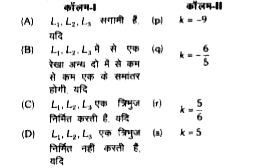
View Solution∫2sinx(3+sin2x)dxisequa<o 12ln∣∣∣2+sinx−cosx2−sinx+cosx∣∣∣−1√2tan−1(sinx+cosx√2)+c 12ln∣∣∣2+sinx−cosx2−sinx+cosx∣∣∣−12√2tan−1(sinx+c0sx√2)+c 14ln∣∣∣2+sinx−cosx2−sinx+cosx∣∣∣−1√2tan−1(sinx+c0sx√2)+c noneofthese
View SolutionLet f(x)=∣∣cos(x+x2)sin(x+x2)−cos(x+x2)sin(x−x2)cos(x−x2)sin(x−x2)sin2x0sin(2x2)∣∣ . Find the value of f′(0).
View Solutionint(cos x-sin x)/(sqrt(8-sin2x))dx=sin^(-1)((sin x+cos x)/(a))+c
View SolutionIf f(x)=|[cos(x +x^2), sin(x +x^2),-cos(x+x^2)],[ sin(x-x^2), cos(x -x^2), sin(x-x^2)],[sin2x,0,sin2x^2]| then find f'(x)
View Solutionint(sin2x)/((sin x+cos x)^(2))dx
View SolutionIf ∫sin8x−cos8x1−2sin2xcos2xdx=asin2x+C then a=
View SolutionThe value of (2(sin2x+2cos^(2)x-1))/(cos x sin x cos3x+sin3x) is
View SolutionThe integral int((sin2x+cos^(2)x)dx)/(1+sin^(2)x(sin2x-cos^(2)x)) is equal to
View Solution(1+sin2xx+sin2x)dx
View SolutionIf αandβ respectively the minmum and minimum values of the fuction f(x) given by
f(x)=∣∣ ∣ ∣∣1+sin2 xcos2x sin2xsin2x 1+cos2x sin2xsin2x cos2x 1+sin2x∣∣ ∣ ∣∣ then which one of
the following options is not true ?
View SolutionIf the maximum and minimum values of the determinant
∣∣ ∣ ∣∣1+sin2xcos2xsin2xsin2x1+cos2xsin2xsin2xcos2x1+sin2x∣∣ ∣ ∣∣ are α and β respectively, then α+2β is equal to
View Solution
