माना A एक 2×2 आव्यूह है।
कथन-1 : adj(adjA)=A कथन-2 : ∣(adjA|=|A∣
कथन-1 सही है, कथन-2 सही है, कथन-1 के लिए कथन-2 का स्पष्टीकरण सही है।
कथन-1 सही है, कथन-2 सही है, कथन-1 के लिए कथन-2 का स्पष्टीकरण नहीं सही है।
कथन-1 सही है, कथन-2 गलत है।
कथन-1 गलत है, कथन-2 सही है।
The correct Answer is:B
Topper's Solved these Questions
- सारणिक तथा आव्यूह BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - JEE ADVANCED MORE THAN ONE CORRECT ANSWERS (Comprehension type questions) (Passage-I)
6 Videos
- सारणिक तथा आव्यूह BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - JEE ADVANCED MORE THAN ONE CORRECT ANSWERS (Comprehension type questions) (Passage-III)
6 Videos
- सारणिक तथा आव्यूह BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - EXERCISE
262 Videos
- सरल रेखा BOOK - ERRORLESS HINDICHAPTER - सरल रेखा EXERCISE - JEE ADVANCE (Matrix Match type questions)
4 Videos
Similar Questions
माना कि p एक विषम अभाज्य संख्या है तथा Tp,2×2 आव्यूहों का निम्न समुच्चय है
Tp={A=[abca]:a,b,c∈{0,1,2,.............p−1}}
Tp में ऐसे A की संख्या जो सममित या विषम सममित अथवा दोनों प्रकार के हो एवं जिनके लिए det(A),p से विभाज्य है, निम्न है
View Solutionमाना A=⎡⎢⎣100210321⎤⎥⎦ तथा U1,U2 व U3,3×3 आव्यूह U के कॉलम है। यदि कॉलम आव्यूह U1,U2 तथा U3,AU1=⎡⎢⎣100⎤⎥⎦,AU2=⎡⎢⎣230⎤⎥⎦,AU3=⎡⎢⎣231⎤⎥⎦ को संतुष्ट करते है, तब निम्न प्रश्नो के उत्तर दीजिए
|U| का मान है
View Solution∣∣ ∣ ∣∣111cos(nx)cos(n+1)xcos(n+2)xsin(nx)sin(n+1)xsin(n+2)x∣∣ ∣ ∣∣ निर्भर नहीं करता है
View Solution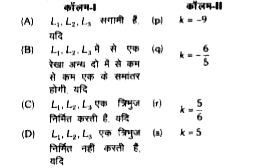
View SolutionIf A is non-singular matrix of order n xx n then find (adj(A))^(-1),adj(A^(-1)),adj(kA),adj(adj(A))
View SolutionIf A = =⎡⎢⎣−1111−1111−1⎤⎥⎦ find the values of
(i) |A||adj A| (ii) |adj (adj (adjA))|
(iii) |adj(3A)| (iv) adj adj A
View SolutionadjAB−(adjB)(adjA)=
View Solution(i) Prove that (adj adj A) =|A|n–2A
(ii) Find the value of |adj adj adj A| in terms of |A|
View SolutionIf adj A=[2341] and adj B=[1−2−31] then adj (AB) is :
View Solutionadj (AB) - (adj B)(adj A) का मान है
View SolutionIf A is a 2×2 non singular matrix, then adj(adj A) is equal to :
View SolutionIf A is a 3×3 matrix and |A|=2, then |Adj(AdjA)|Adj(AdjA) =
View SolutionIf A is a 3×3 matrix and |A|=2, then |Adj(Adj(Adj(Adj(A)|=
View Solutionवक्तव्य I यदि A , 2×2 कोटि का आव्यूह है तब |adj A| = |A|
, वक्तव्य II |A|=∣∣AT∣∣
View SolutionLet A, 2xx2 Class is a matrix Statement I adj (adj A) = A Statement II | adj A | = A
View Solution
