यदि z=tan−1(xy), तो zx:zy=
y:x
x:y
−y:x
−x:y
The correct Answer is:C
Step by step video, text & image solution for यदि z=tan^(-1)((x)/(y)), तो z_(x):z_(y)= by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- अवकलन तथा अवकलज के अनुप्रयोगBook:ERRORLESS HINDIChapter:अवकलन तथा अवकलज के अनुप्रयोगExercise:MULTIPLE CHOICE QUESTIONS (एक बिन्दु पर अवकलज,मानक अवकलज)
Explore 186 Videos
- अवकलन तथा अवकलज के अनुप्रयोगBook:ERRORLESS HINDIChapter:अवकलन तथा अवकलज के अनुप्रयोगExercise:MULTIPLE CHOICE QUESTIONS (अस्पष्ट फलनों का अवकलन,प्रचलिक व संयुक्त फलनों का अवकलन,लघुगणकीय अवकलन, अनन्त श्रेणियों के अवकलन )
Explore 64 Videos
- अवकल समीकरण Book:ERRORLESS HINDIChapter:अवकल समीकरण Exercise:JEE Advanced ( Matrix Match type questions )
Explore 3 Videos
- केन्द्रीय प्रवृत्ति के मापBook:ERRORLESS HINDIChapter:केन्द्रीय प्रवृत्ति के मापExercise:Jee Advanced (Reasoning )
Explore 2 Videos
Similar Questions
2f(x)=f'(x) एवं f(0)=3, तब f(2)=
View Solutionमाना y,x2x−2xxcoty−1=0 से परिभाषित x का एक अस्पष्ट फलन है। तब y'(1) बराबर है
View Solution
View Solutionकथन - 1 : प्रथम n सम धन पूर्णांकों का प्रसरण n2−14 है।
कथन - 2 : प्रथम n धन पूर्णाको का योगफल n(n+1)2 और प्रथम n धन पूर्णांको के वर्गों का योगफल n(n+1)(2n+1)6 है।
View SolutionFor any complex number z=x+ iy,define (z)=|x|+|y|. If z_(1) and z_(2) are any two complex numbers,then (1) (z_(1)+z_(2))lt=(z_(1))+(z_(2)) (2) (z_(1)+z_(2))=(z_(1))+(z_(2)) (3) (z_(1)+z_(2))gt=(z_(1))+(z_(2)) (4) (z_(2)-z_(1))=(z_(2))-(z_(1))
View SolutionIn the diagram as shown, find parameters representing x and y axes and also parameter Z, if Z1=X2,Z3=Z4 and Z2>Z3:
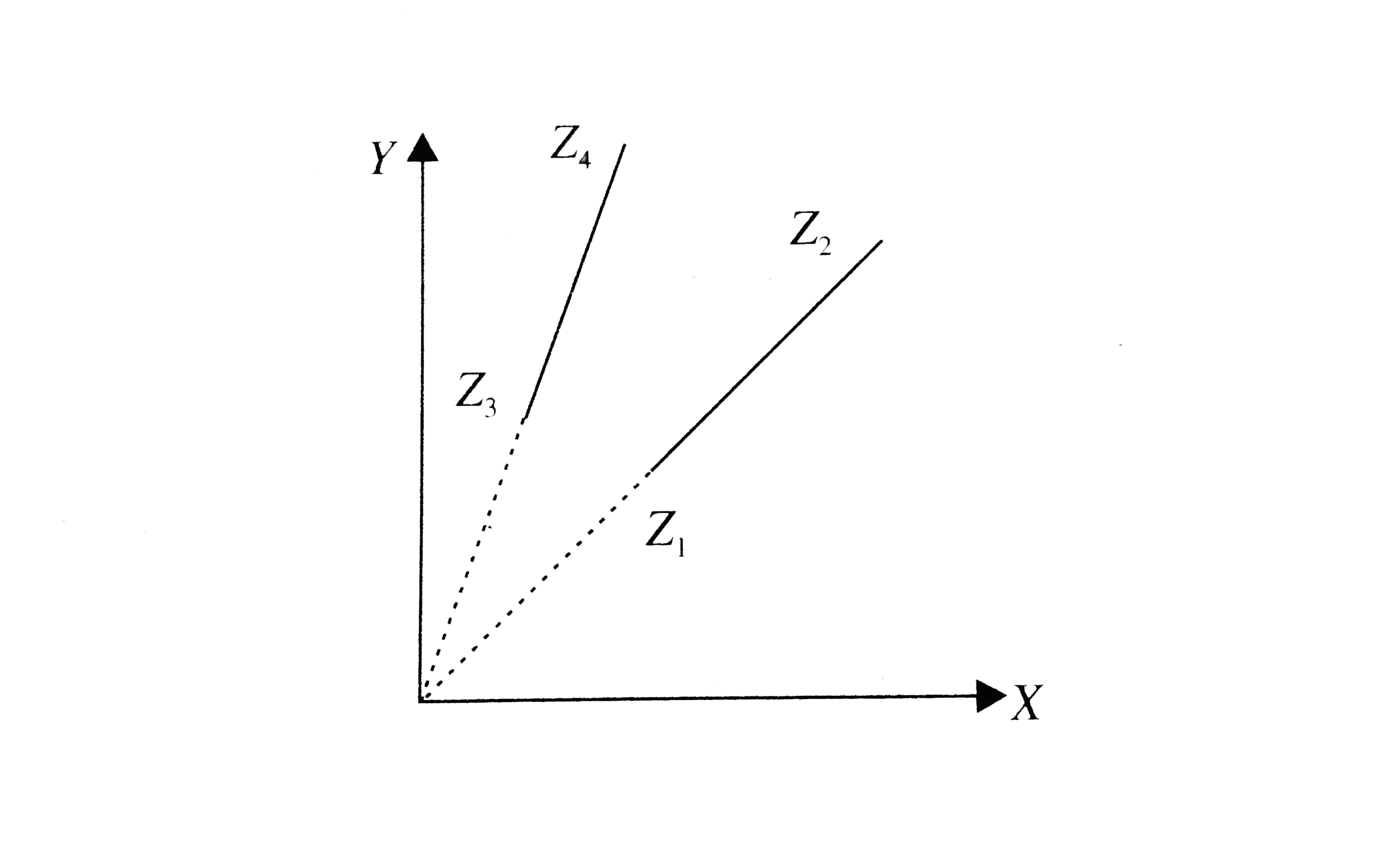
View SolutionConsider the set of equation x+y+xy=35,y+z+yz=8,z+x+zx=3. If z1,z2 are possible values of z, then |z1−z2| is equal to
View Solutionरेखा x−x1l−y−y1m=z−z1n समतल a(x−x1)+b(y−y1)+c(z−z1)=0 पर स्थित होगी यदि
View SolutionA function f(z1,z2,z3,.....zn)=f(z1,z2)+f(z2,z3,....,zn−1)+(z1+z2+....zn), for n>0f(y,z)=f(z,0)+f(0,y)
f(y,0)=y+f(y−1,0)
f(0,y)=y−f(0,y−1)
f(0,0)=1
Find the value of f(1,2,3,4,........n), where n is a perfect cut less than 50 and n is greater than 25:
View SolutionA function f(z1,z2,z3,.....zn)=f(z1,z2)+f(z2,z3,....,zn−1)+(z1+z2+....zn), for n>0f(y,z)=f(z,0)+f(0,y)
f(y,0)=y+f(y−1,0)
f(0,y)=y−f(0,y−1)
f(0,0)=1
Find the value of f(0,1,0,1):
View SolutionA function f(z1,z2,z3,.....zn)=f(z1,z2)+f(z2,z3,....,zn−1)+(z1+z2+....zn), for n>0f(y,z)=f(z,0)+f(0,y)
f(y,0)=y+f(y−1,0)
f(0,y)=y−f(0,y−1)
f(0,0)=1
Find the value of f(8,8,8,2,2,2):
View SolutionA function f(z1,z2,z3,.....zn)=f(z1,z2)+f(z2,z3,....,zn−1)+(z1+z2+....zn), for n>0f(y,z)=f(z,0)+f(0,y)
f(y,0)=y+f(y−1,0)
f(0,y)=y−f(0,y−1)
f(0,0)=1
Find the value of f(1,1,3,1,1,3):
View SolutionA function f(z1,z2,z3,.....zn)=f(z1,z2)+f(z2,z3,....,zn−1)+(z1+z2+....zn), for n>0f(y,z)=f(z,0)+f(0,y)
f(y,0)=y+f(y−1,0)
f(0,y)=y−f(0,y−1)
f(0,0)=1
f(9,2,k,0,9,4)=124, then the value of k is :
View SolutionIn the diagram as shown, find parameters representing x and y axes and also parameter Z, if Z1=Z2,Z3=Z4 and Z2>Z3:

View SolutionIf Z_("eff") of F (Z=9) is X and Z_("eff") of Li (Z = 3) is Y then find the value of |X-Y|
View Solution