माना कि दीर्घ वृत की नाभियाँ और हैं, जहाँ और हैं। माना कि एवं दो परवलय हैं जिनकी नाभियाँ क्रमशः एवं हैं तथा दोनों के शीर्ष (vertex) (0,0) है। माना कि की स्पर्श रेखा बिन्दु से, एवं की स्पर्श रेखा बिन्दु से गुजरती हैं। यदि की प्रवणता हो और की प्रवणता हो, तब का मान ज्ञात करो ।
The correct Answer is:4
Step by step video, text & image solution for माना कि दीर्घ वृत x^2/9 +y^2/5=1 की नाभियाँ (f_(1), 0) और (f_(2),0) हैं, जहाँ f_1 gt 0 और f_(2) lt 0 हैं। माना कि P_1 एवं P_2 दो परवलय हैं जिनकी नाभियाँ क्रमशः (f_(1),0) एवं (2f_(2),0) हैं तथा दोनों के शीर्ष (vertex) (0,0) है। माना कि P_1 की स्पर्श रेखा T_1 बिन्दु (2f_2,0) से, एवं P_2 की स्पर्श रेखा T_2 बिन्दु (f_(1),0) से गुजरती हैं। यदि T_1 की प्रवणता m_1 हो और T_2 की प्रवणता m_2 हो, तब (1/(m_1^2)+m_2^2) का मान ज्ञात करो । by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ERRORLESS HINDICHAPTER - शांकव परिच्छेद EXERCISE - JEE Advanced (Matrix Match type questions)7videos
शांकव परिच्छेद
- BOOK - ERRORLESS HINDICHAPTER - शांकव परिच्छेद EXERCISE - JEE Advanced (Comprehension type questions) Passage-XII3videos
शांकव परिच्छेद
- BOOK - ERRORLESS HINDICHAPTER - वृत्त तथा वृत्तों का निकाय EXERCISE - JEE Advanced (Integer type questions)20videos
वृत्त तथा वृत्तों का निकाय
- BOOK - ERRORLESS HINDICHAPTER - श्रेणियाँ EXERCISE - JEE ADVANCED (MATRIX MATCH TYPE QUESTIONS)1videos
श्रेणियाँ
Similar Questions
 View Solution
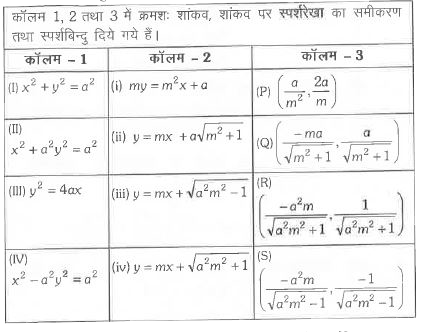
View Solutionनिचे दी गयी टेबल के तीन कॉलमों में उपलब्ध सूचना का उपयुक्त ढंग से सुमेल कर प्रश्नों के उत्तर दीजिये |
a=√2 के लिए उपयुक्त शांकव (कॉलम 1) के स्पर्शबिन्दु (−1,1) पर एक स्पर्शरेखा खींची जाती है तब निम्न में से केवल कौन सा विकल्प इस स्पर्शरेखा का समीकरण प्राप्त करने का सही संयोजन है02:22View Solutionरेखा 2x−3y=1 वृत्तीय क्षेत्र x2+y2≤6 को दो भागों में विभाजित करती है। यदि
S={(2,34),(52,34),(14,−14),(18,14)}, तो S में स्थित उन बिन्दुओं की संख्या जो लघुत्तर भाग में अन्दर है, निम्न है05:25View Solutionनिम्नलिखित कॉलम I को कॉलम II से मिलाये
 02:59View Solution
02:59View SolutionSuppose that the foci of the ellipse x29+y25=1 are (f1,0)and(f2,0) where f1>0andf2<0. Let P1andP2 be two parabolas with a common vertex at (0,0) and with foci at (f1,0)and(2,f20), respectively. Let T1 be a tanent to P1 which passes through (2f2,0) and T2 be a tangent to P2 which passes through (f1,0). If m1 is the slope of T1andm2 is the slope of T2, then the value of (1m21+m22) is __________
05:04View SolutionLet f(1):R→R,f2:[0,∞)→R,f3:R→Randf4:R→[0,∞) be a defined by
f1(x)={|x|if x<0exif x>0:f2(x)=x2,f3(x)={sinxif x<0xif x≥0 and f4(x)={f2(f1(x))if x<0f2(f1(f1(x)))−1if x≥0 Then, f4 is05:09View SolutionSuppose that the foci of the ellipse x29+y25=1 are (f1,0)and(f2,0) where f1>0andf2<0 Let P1andP2 be two parabola with a c ommon vertex at (0, 0) and with foci at (f1,0)and(2fz,0) and T2 be a tangent to P2 which passes through (f1,0). If m1 is the slope of T1andm2 is the slope of T2, then the value of (1m21+m22), is
01:59View Solutionमाना कि X={1,2,3,4,5,6,7,8,9}, माना कि X में सम्बन्ध R1 तथा R2 इस प्रकार हैं कि R1={(x,y):x−y,3 से विभाज्य हैं,x≠y} तथा R2={(x,y):{x,y}⊂{1,4,7} या {x,y}⊂{2,5,8} या {x,y}⊂{3,6,9} जहाँ ⊂ का अर्थ उपसमुच्चय से हैं| दिखाएं कि R1=R2
01:02View SolutionLet f1:R→R,f2:[0,∞)→R,f3:R→R be three function defined as
f1(x)={|x|x<0exx≥0,f2(x)=x2,f3(x)={f2(f1(x))x<0f2(f1(x))−1x≥0 then f3(x) is:05:01View Solution''स्तम्भ'अ'''''स्तम्भ'ब'''बिन्दु(−4,5)एवं(4,−5)के मध्य-बिन्दु के निर्देशांकy(0,3)एवं(4,0)के बीच की दुरी (x)मूल बिन्दु से(x,y)के बीच की दुरी0,0(0,y) बिन्दु की कोटि 5(x,0) बिन्दु की भुज√x2+y2
03:23View Solutionl+(f1−f02f1−f0−f2)×hसूत्र है:
02:46View Solutionबहुलक =l+(f1−f02f1−f0−f2)×h में h होता है ।
View SolutionSuppose that the foci of the ellipse x29+y25=−1 are (f1,0) and (f2,0) where f1>0 and f2<0. Let P1 and P2 be two parabolas with a common vertex at (0, 0) and with foci at (f1,0) and (2f2,0) respectively. Let T1 be a tangent to P1 which passes through (2f2,0) and T2 be a tangent to P2 which passes Through (f1,0). If m1 is the slope of T1 and m2 is the slope of T2, then the value of (1m21+m22) is -
06:14View Solutionमाना दीर्घवृत्त x29+y25=1 की नाभियाँ (foci) (f1,0) और (f2,0) हैं, जहाँ f1>0 और f2<0 हैं। माना P एवं 2 दो परवलय (parabola) हैं। जिनकी नाभियाँ क्रमश: (f1,0) एवं (2f2,0) हैं तथा दोनों के शीर्ष (vertex) (0, 0) है। माना P की स्पर्श रेखा 7, बिन्दु (2f2,0) से एवं P2 की स्पर्श रेखा T2 बिन्दु (f1,0) से गुजरती हैं। यदि T1 की प्रवणता (slope) m1 हो और T2 की प्रवणता m2 हो, तब (1m21+m22) का मान है।
07:47View Solutionपरवलय y2=2x की स्पर्श रेखा 18x-6y+1= 0 का स्पर्श बिन्दु है
05:28View Solution(A) (B)1. समचतुर्भुज के विकर्ण होते हैं(a) अद्वितीय हल2. त्रिभुज की दो भुजाएँ बराबर होतीए हैं(b) लंबवत3. बहुलक(c) समद्विबाहु त्रिभुज4. प्रायिकता(d) l1+(f1−f0)2f1−f0−f2×(l2−l1)5. रेखाएँ प्रतिच्छेदी हो(e) n(A)n(S)
View Solution
