माना दीर्घवृत्त $\frac{x^{2}}{9} + \frac{y^{2}}{5} = 1$ की नाभियाँ (foci) $(f_{1}, 0) और (f_{2}, 0)$ हैं, जहाँ $f_{1} > 0 और f_{2} < 0$ हैं। माना P एवं 2 दो परवलय (parabola) हैं। जिनकी नाभियाँ क्रमश: $(f_{1}, 0) एवं (2 f_{2}, 0)$ हैं तथा दोनों के शीर्ष (vertex) (0, 0) है। माना P की स्पर्श रेखा 7, बिन्दु $(2 f_{2}, 0)$ से एवं $P_{2}$ की स्पर्श रेखा $T_{2}$ बिन्दु $(f_{1}, 0)$ से गुजरती हैं। यदि $T_{1}$ की प्रवणता (slope) $m_{1}$ हो और $T_{2}$ की प्रवणता $m_{2}$ हो, तब $(\frac{1}{m_{1}^{2}} + m_{2}^{2})$ का मान है।

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $\frac{1}{m_{1}^{2}} + m_{2}^{2} = 4$

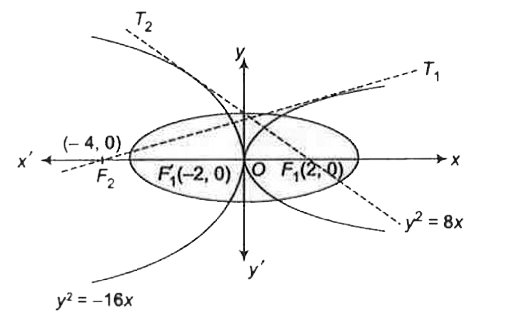
$y^{2} = - 16 x$
$P_{1}$ के सापेक्ष स्पर्शी रेखा जो $(2 f_{2}, 0) अर्थार्त (- 4, 0)$ से होकर गुजरती है
$∴ T_{1} : y = m_{1} x + \frac{2}{m_{1}}$
$\Rightarrow 0 = - 4 m_{1} + \frac{2}{m_{1}}$
$\Rightarrow m_{1}^{2} = 1 / 2 ... ... . (i)$
साथ ही $P_{2}$ के सापेक्ष स्पर्शी रेखा जो $(f_{1}, 0)$ अर्थार्त (2,0) से होकर गुजरती है
$\Rightarrow T_{2} : y = m_{2} x + \frac{(- 4)}{m_{2}} \Rightarrow 0 = 2 m_{2} - \frac{4}{m_{2}}$
$\Rightarrow m_{2}^{2} = 2 ... ... (i i)$
$∴ \frac{1}{m_{1}^{2}} + m_{2}^{2} = 2 + 2 = 4$

Updated on:21/07/2023

|

Class 12 MATHS परवलय

Topper's Solved these Questions

Similar Questions

JEE Main & Advanced (Hindi Medium)-परवलय-एकल पूर्णांक प्रश्न

Home
Ask Doubt
Profile