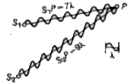
माना S1 और S2 दो स्रोत हैं और S1 से आने वाली तरंग बिन्दु P पर तरंगदैर्ध्य (λ) के 7 गुना दूरी तय करके पहुँचती है, तथा S2 से आने वाली तरंग, तरंगदैर्ध्य (λ) के 9 गुना दूरी तय करके बिन्दु P पर पहुंचती है।

निम्न में से सत्य कथन है
I. S2P−S1P=2λ
II. S1 से आने वाली तरंगें S2 से दो चक्र पहले पहुँचेगी,
III. S1 और S2 बिन्दु P पर कला में हैं।
IV. S1 और S2 बिन्दु P पर कला में नहीं हैं।
I, II, और III
I, III और IV
II, III और IV
I, II और IV
The correct Answer is:A
दिया गया है,
S1P=7λ और S2P=9λ अतः S2P−S1P=9λ−7λ
⇒S2P−S1P=2λ
S1 स्रोत से उत्पन्न तरंग, S2 स्रोत की तुलना में दो चक्र पहले पहुँचेगी तथा पुनः समान कला में होगी।
Similar Questions
Microwave ocillators S1andS2 are used to show interference of electromagnetic waves on a screen which in parallel to S1S2 and 2 m away from S1S2 . If S1S2=20 cm and λ=4cm, then the distance between the successive maxima and minima will be
View SolutionIf P is a point on the hyperbola 16x2−9y2=144 whose foci are S1 and S2 then : |S1P−S2P|=
View SolutionIf 1,2,3….are first terms, 1,3,5….are common differences and S1,S2,S3…….are sums of n terms of given p AP's, then S1+S2+S3+…….+Sp is equal to
View Solutionमाना Sk=1+2+3+…+kk है। यदि S21+S22+….S210=512A है, तो A बराबर है
View SolutionIf s1,s2,s3…..sp are the sums of n terms of 'p' A.P's whose first terms are 1,2,3…p and common differences are 1,3,5… (2p−1) respectively then s1+s2+s3+…+sp=
View SolutionIf S1 and S2 are the foci of x2a2+y2b2=1 and P is any point on it, then the area PS1S2 will be maximum, whenP=
View SolutionIf P is any point on the hyperbola (x−1)29−(y+1)216=1 and S1 and S2 are its foci, then |S1P−S2P|=
View SolutionA monochromatic light source of wavelength lamda is placed at S. three slits S_(1),S_(2) and S_(3) are equidistant from the source S and the point P on the screen S_(1)P-S_(2)P=lamda//6 and S_(1)P-S_(3)P=2lamda//3 . if I be the intensity at P when only one slit is open the intensity at P when all the three slits are open is-
View Solution
