A wooden log of mass M and length L is hinged by a frictionless nail at O.A bullet of mass m strikes with velocity v and sticks to it. Find angular velocity of the system immediately after the collision aboutO.

Video Solution

Text Solution

Verified by Experts

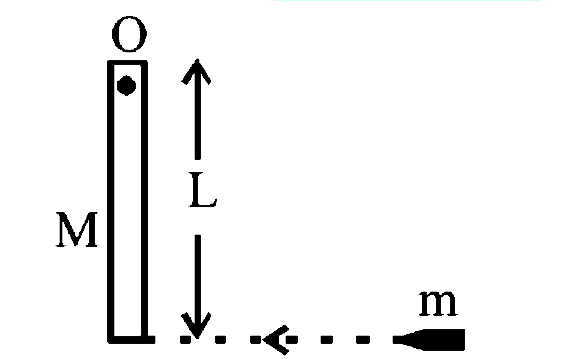
The correct Answer is:A, C

We know that $τ = \frac{d v a c L}{d t}$
$τ \times d t = d \to L$
when angular impulse $(τ \times d \to t)$ is zero, the anglar
momentum is constant. In this case for the wooden
log-bullet system the angular impulse about O is constant .
therefore.
${[a n g a - - r m o m e n t u m o f t h e s y s t e m]}_{\in i t i a l}$
$= {[a n g a - - r m o m e n t u m o f t h e s y s t e m]}_{\in i t a l}$
$\Rightarrow m v \times L = I_{0} \times ω ... . . (i)$
where $I_{0}$ is the moment of inertia of the wooden log - bullet
system after collision aobut O
$I_{0} = I_{w \infty d e n log} + I_{b \leq - - - t}$
$= \frac{1}{3} M L^{2} + M L^{2} ... . (i i)$
From (i) and (ii)
$ω = \frac{m v \times L}{[\frac{1}{3} M L^{2} + m L^{2}]}$
$\Rightarrow o e m g a = \frac{m v}{\frac{M L}{3} + m L} ⎤ ⎦$ = (3mv)/((M+3m))L)

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A bullet of mass A and velocity B is fried into a block of mass C and sticks to it. The final velocity of the system equals
A $\frac{A + B}{C} A$
B $\frac{A}{A + C} B$
C $\frac{A + C}{B}$
D $\frac{A}{A + B} B$
Question 2 - Select One
A bullet of mass m is fired with velocity v in a large bulk of mass M. The final velocity v' will be
A $\frac{M v}{m + M}$
B $\frac{m v}{m + M}$
C $\frac{(m + M) v}{m}$
D $(m + M) v$
Question 3 - Select One
A bullet of mass m is fired with velocity v in a large bulk of mass M. The final velocity v' will be
A $\frac{M v}{m + M}$
B $\frac{m v}{m + M}$
C $\frac{(m + M) m}{m}$
D $(m + M) v$

SUNIL BATRA (41 YEARS IITJEE PHYSICS)-ROTATIONAL MOTION-MCQs with one correct answer

Home
Ask Doubt
Profile