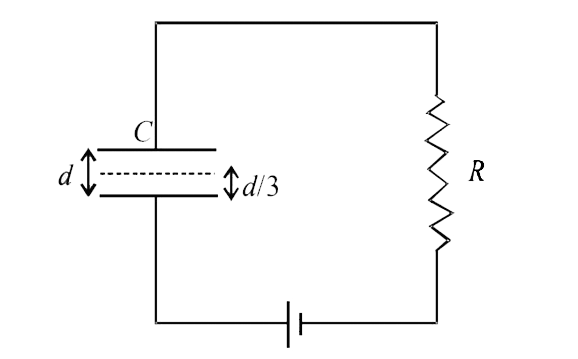
A parallel plate capacitor C with plates of unit area and separation d is filled with a liquid of dielectric constant K=2. The level of liquid is d/3 initially. Suppose the liquid level decreases at a constant speed v, the time constant as a function of time t is-
(a) 6eπslon0R5d+3vt
(b) (15d+9vt)eπslon0R2d2−3dvt−9v2t2
(c) 6eπslon0R5d−3vt
(d) (15d−9vt)eπslon0R2d2−3dvt−9v2t2
The correct Answer is:A
Let the level of liquid at an instant of time 't' be x. Then
V=−dxdt⇒dx=−vdt
⇒∫xd/3dx=−v∫t0dt
⇒x−d3=vt
⇒x=d3−vt ltbrrgt 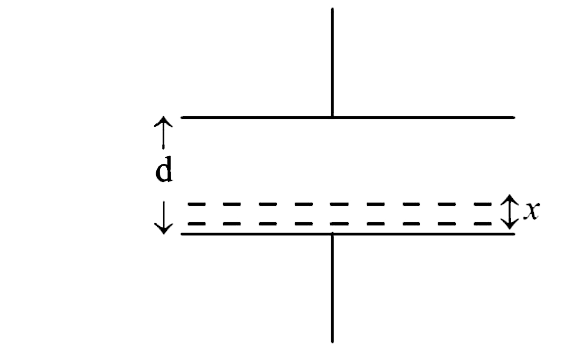
Also the capacitance can be conisdered as an equivalent of two capacitances in series such that
1Ceq=1C1+1C2
⇒1Ceq=1∈0Ad−x+1∈0AKx=d−x∈0A+x∈0AK
∴ Ceq=∈0AKKd+x(1−K)
But A=1, K=2 and x=d3−vt
∴ Ceq=∈0×1×22d+[d3−vt](1−2)=6∈05d+3vt
∴ Time constant τ=RCeq=6R∈05d+3vt
Topper's Solved these Questions
- ELECTROSTATICSBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - ELECTROSTATICSEXERCISE - Subjective Problems
2 Videos
- ELECTROSTATICSBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - ELECTROSTATICSEXERCISE - Comprehension Based Questions
2 Videos
- ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTEXERCISE - JEE Main And Advanced
107 Videos
- MODERN PHYSICSBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MODERN PHYSICSEXERCISE - MCQ (One Correct Answer
1 Videos
Similar Questions
A parallel plate capacitor with plate area A & plate separation d is filled with a dielectric material of dielectric constant given byK=K0(1+αx).. Calculate capacitance of system: (given αd<<1).

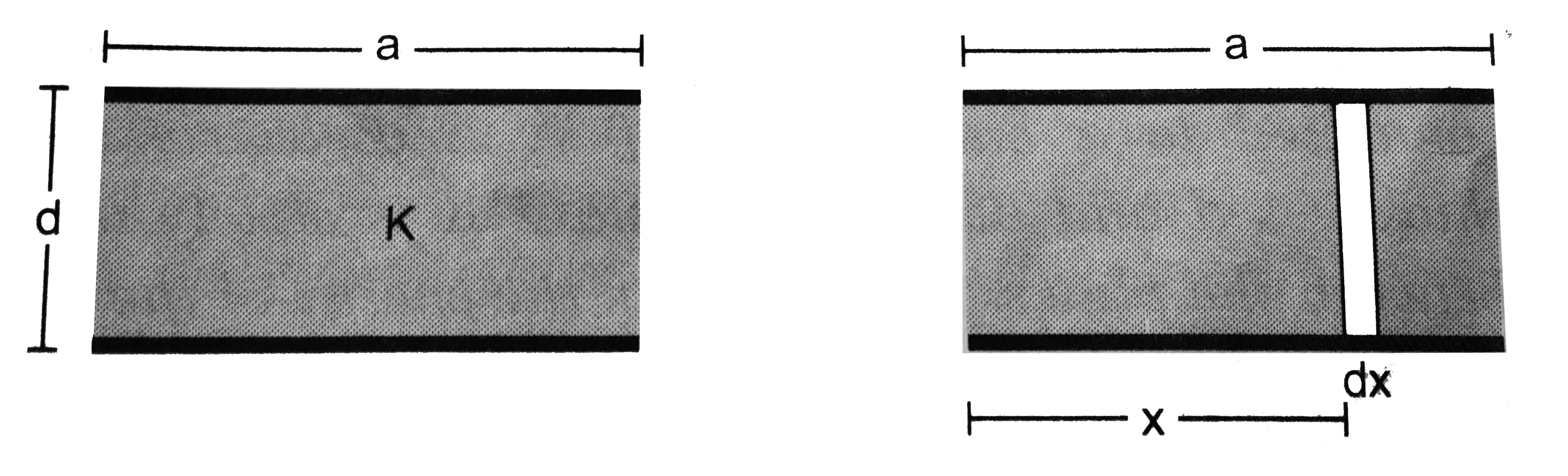
View SolutionFigure shown a parallel-plate capacitor having square plates of edge a and plate-separation d. The gap between the plates is filled with a dielectric of dielectric constant K which varies parallel to an edge as K=K0+αx, where K and α are constants and x is the distance from the left end. Calculate the capacitance.
View Solution
Knowledge Check
A parallel plate capacitor C with plates of unit area and separation d is filled with a liquid of dielectric constant K=2. The level of liquid is d/3 initially. Suppose the liquid level decreases at a constant speed v, the time constant as a function of time t is-
 A6ε0R5d+3/tB(15d+9vt)ε0R2d3−3dvt−gv2t2C6ε0R5d−3vtD(15d−9vt)ε0R2d3+3dvt−9v2t2
A6ε0R5d+3/tB(15d+9vt)ε0R2d3−3dvt−gv2t2C6ε0R5d−3vtD(15d−9vt)ε0R2d3+3dvt−9v2t2A capacitor of plate area A and separation d is filled with two dielectrics of dielectric constant K1=6andK2=4. New capacitance will be
 A4Aε0dB4.8Aε0dC5Aε0dD2.4Aε0d
A4Aε0dB4.8Aε0dC5Aε0dD2.4Aε0dA parallel plate capacitor of area ‘A’ plate separation ‘d’ is filled with two dielectrics as shown. What is the capacitance of the arrangement ?
 A3Kε0A4dB4Kε0A3dC(K+1)ε0A2dDK(K+3)ε0A2(K+1)d
A3Kε0A4dB4Kε0A3dC(K+1)ε0A2dDK(K+3)ε0A2(K+1)d
A parallel plate capacitor having plate area A and separation between the plates d is charged to potential V.The energy stored in capacitor is ....???
View SolutionTwo conducting plates each having area A are kept at a separation d parallel to each other. The two plates are con- nected to a battery of emf V. The space between the plates is filled with a liquid of dielectric constant K. The height (x) of the liquid between the plates increases at a uniform rate from zero to d in a time interval t0.
(a) Write the capacitance of the system as a function of x as the liquid beings to fill the space between the plates.
(b) write the current through the cell at time t
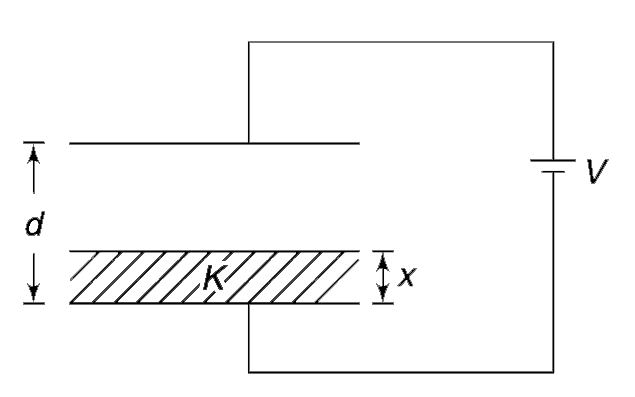
View SolutionIf the gap between the plates of a parallel plate capacitor is filled with medium of dielectric constant k=2 , then the field between them
View SolutionA parallel plate capacitor of plate area A and separation d is filled with two materials each of thickness d2 and dielectric constants ∈1 and ∈2 respectively. The equivalent capacitance will be

View SolutionA parallel plate capacitor carries a harge Q. If a dielectric slab with dielectric constant K=2 is dipped between the plates, then
View Solution
