Two charges −2Q abd Q are located at the points with coordinates (−3a,0) and (+3a,0) respectively in the x-y plane. (i) Show that all points in the x-y plane where the electric potential due to the charges is zero, on a circle. Find its radius and the location of its centre (ii) Give the expression V(x) at a general point on the x-axis and sketch the function V(x) on the whole x-axis. (iii) If a particle of charge +q starts from rest at the centre of the circle, shown by a short quantitative argument that the particle eventually crosses the circule. Find its speed when it does so.
The correct Answer is:A, B, C, D
Let P be a point in the X-Y plane with coordinates (x,y) at which the potential due to charges −2Q and +Q placed at A and B respectively be zero.
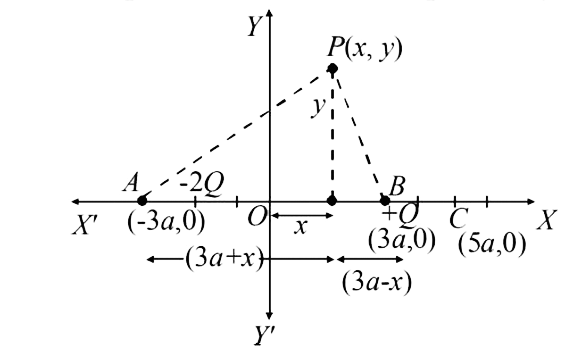
∴ K(2Q)√(3a+x)2+y2=K(+Q)√(3a−x)2+y2
⇒2√(3a−x)2+y2=√(3a+x)2+y2
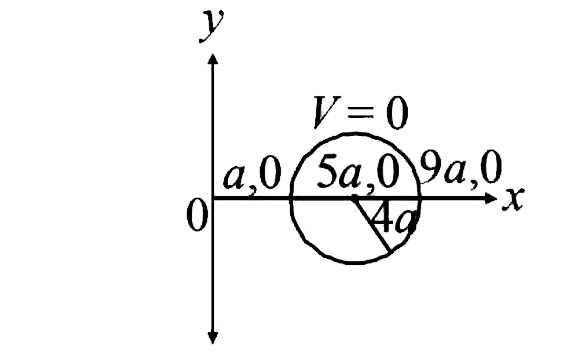
⇒(x−5a)2+(y−0)2=(4a)2
This is the equation of a circle with centre at (5a,0) and radius 4a. Thus C (5a,0) is the centre of the circle.
(b) For xgt3a
To find V(x) at any point on X-axis, let us consider a point (arbitrary) M at a distance x from the origin.
The potential at M will be
V(x)=K(−2Q)x+3a+K(+Q)(x−3a) where k=14πε0
∴ V(x)=KQ[1x−3a−2x+3a] for |x|>3a
Similarly, for 0<|x|<3a
V(x)=KQ[13a−x−23a+x]
Since circle of zero potential cuts the x-axis at (a,0) and (9a,0)
Hence, V(x)=0 at x=a, at x=9a
* From the above expressions
V(x)→∞ at x→3a and V(x)→−∞ at x→−3a
* V(x)→0 as x→±∞
* V(x) varies 1/x in general.
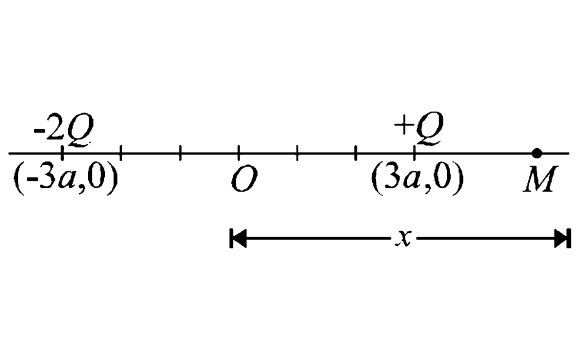
(c) Applying Energy Conservation
(K.E.+P.E.)centre=(K.E.+P.E.)circumference
0+K[Qq2a−2Qq8a]=12mv2+K[Qq6a−2Qq12a]
12mv2=KQq4a, v=√KQq2ma=√14πε0(Qq2ma)
(##JMA_El_C12_087_S04.png" width="80%">
Topper's Solved these Questions
- ELECTROSTATICSBook:SUNIL BATRA (41 YEARS IITJEE PHYSICS)Chapter:ELECTROSTATICSExercise:Subjective Problems
Explore 2 Videos
- ELECTROSTATICSBook:SUNIL BATRA (41 YEARS IITJEE PHYSICS)Chapter:ELECTROSTATICSExercise:Comprehension Based Questions
Explore 2 Videos
- ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTBook:SUNIL BATRA (41 YEARS IITJEE PHYSICS)Chapter:ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTExercise:JEE Main And Advanced
Explore 107 Videos
- MODERN PHYSICSBook:SUNIL BATRA (41 YEARS IITJEE PHYSICS)Chapter:MODERN PHYSICSExercise:MCQ (One Correct Answer
Explore 1 Video
Similar Questions
Two fixed charges -2Q and Q are located at the point with coordinates (-3a,0) and (+3a,0) respectively in the x- y plane .
Show that all points in the x-y plane where the electric potential due to the two charge is zero , lie on a circle. If its radius is r = xa and the location of its centre is (ya,0) find x and y .
View SolutionTwo fixed charges -2Q and Q are located at the point with coordinates (-3a,0) and (+3a,0) respectively in the x- y plane .
If a particle of charge +q starts from rest at the centre of the circle , show by a short quantitavtive argument that the particle eventually crosses the circle . If its speed V=√Qqzπε0ma , then find z.
View Solution
Knowledge Check
Two fixed charges −2Q and Q are locatted at the points with coordinates ( -3 a, 0) and (+3 a, 0) prespectively in the X−Y plane .
All the points in the X−Y plane where the electric potential due to the two charges is zero , line on a .Astraight lineBcircleCparabolaDellipseTwo fixed charges −2Q and Q are locatted at the points with coordinates ( -3 a, 0) and (+3 a, 0) prespectively in the X−Y plane .
The expression for the potential V(x) at a general point on the x-axis is given by .AV(x)=14πɛ0[Q3a−x−2Q(3a+x)]for0<x<3aBV(x)=14πɛ0[2Q3a−x−2Q(3a+x)]for0<x<3aCV(x)=14πɛ0[Q3a−x−2Q(3a+x)]for0<x<3aDV(x)=14πɛ0[Q3a−x−2Q(3a+x)]for0<x<3aTwo fixed charges −2Q and +Q are located at points (−3a,0) and (+3a,0) respectively. Then which of the following statement is correct?
APoints where the electric potential due to the two charges is zero in x-y plane, lie on a circle radius 4a and centre (5a,0)Bpotential is zero at x=a and x=9aCboth a and b are wrongDboth a and b are correct
Two charges - q and +q are located at points (0, 0, - a) and (0,0, a), respectively.
What is the electrostatic potential at the points (0, 0, z) and (x, y,0) ?
View SolutionTwo charges +q and -q are kept at (−x1,0)and(x1,0) respectively in the x-y plane. Find the magnitude and direction of the net electric field at the origin (0,0)
View Solutiontwo point charges + 16 q and -4q located at x=0 and x=L respectively. the location of a point on the x-axis from x=0, at which the net electric field due to these two charges is zero is
View SolutionTwo point charges +8q and -2q located at x =0 and x=L respectively . The location of a point on the x-axis at which the net electric field due to these two point charges is zero is.
View SolutionTwo point charges +8q and −2q are located at x=0 and x=L respectively. The location of a point on the x axis at which the net electric field due to these two point charges is zero is
View Solution