A parallel plate capacitor is made by stacking n equally spaced plates connected alternatively. If the capacitance between any two adjacent plates is 'C' then the resultant capacitance is
(a) (n+1)C
(b) (n−1)C
(c) nC
(d) C
The correct Answer is:B
To solve the problem of finding the resultant capacitance of a parallel plate capacitor made by stacking n equally spaced plates connected alternatively, we can follow these steps:
1. Understanding the Configuration:
- We have n plates stacked on top of each other.
- The plates are connected alternatively, meaning that every two adjacent plates form a capacitor.
2. Identifying the Number of Capacitors:
- The number of pairs of adjacent plates (which form capacitors) can be calculated as n−1. This is because for n plates, there are n−1 gaps between them.
3. Capacitance of Each Pair:
- Each pair of adjacent plates has a capacitance of C.
4. Connecting Capacitors:
- Since the plates are connected alternatively, the capacitors formed between the plates are in parallel.
5. Calculating the Total Capacitance:
- For capacitors in parallel, the total capacitance Ceq is the sum of the individual capacitances:
Ceq=(n−1)×C
Final Result
Thus, the resultant capacitance between any two adjacent plates in this configuration is:
Ceq=(n−1)C
- We have n plates stacked on top of each other.
- The plates are connected alternatively, meaning that every two adjacent plates form a capacitor.
- The number of pairs of adjacent plates (which form capacitors) can be calculated as n−1. This is because for n plates, there are n−1 gaps between them.
- Each pair of adjacent plates has a capacitance of C.
- Since the plates are connected alternatively, the capacitors formed between the plates are in parallel.
- For capacitors in parallel, the total capacitance Ceq is the sum of the individual capacitances:
Ceq=(n−1)×C
Thus, the resultant capacitance between any two adjacent plates in this configuration is:
Ceq=(n−1)C
Topper's Solved these Questions
- ELECTROSTATICSBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - ELECTROSTATICSEXERCISE - Subjective Problems
2 Videos
- ELECTROSTATICSBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - ELECTROSTATICSEXERCISE - Comprehension Based Questions
2 Videos
- ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTEXERCISE - JEE Main And Advanced
107 Videos
- MODERN PHYSICSBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MODERN PHYSICSEXERCISE - MCQ (One Correct Answer
1 Videos
Similar Questions
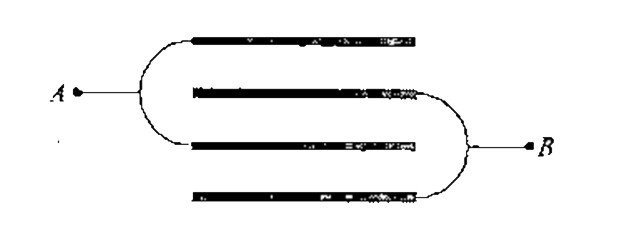
Figure shows four parallel plates kept at equal separation forming three parallel plate capacitors. The capacitance between two adjoining plates is C. Find out equivalent capacitance between A and B.
View SolutionIn a parallel plate capacitor with air between the plates, each plate has an area of 6×10−3m2 and distance between the plates is 3mm. Calculate the capacitance. If this capacitance is connected to a 100V supply, what is the charge on each plate of the capacitor ?
View Solution
Knowledge Check
n equally spaced plates are stacked to make a parallel plate capacitor. If-C is the POLES, capacitance between two adjacent plates, the resultant capacitance is :
AnCBn2CC(n−1)CDCnA parallel plate capacitor has 91 plates, all are identical and arranged with same spacing between them. If the capacitance between adjacent plates is 3 pF. What will be the resultant capacitance?
A273 pFB30 pFC94 pFD270 pFA parallel plate capacitor is filled with dielectrics. What is capacitance?
 A2K1K2K1+K2BK1K2K1+K2C2ε0Ad(K1K2K1+K2)D2ε0Ad(2K1+K22K1K2)
A2K1K2K1+K2BK1K2K1+K2C2ε0Ad(K1K2K1+K2)D2ε0Ad(2K1+K22K1K2)
A variable capacitor has n plates and the distance between two successive plates is d. determine its capacitance.
View SolutionA parallel plate capacitor with air between the plates has a capacitance of 10 pF. The capacitance, if the distance bgetween the plates is reduced by half and the space between tehm is filled with a substance of dielectric constant 4 is
View SolutionA parallel plate capacitor of capacitance C is charged to a potential V and then disconnected with the battery. If separation between the plates is decreased by 50% and the space between the plates is filled with a dielectric slab of dielectric constant K=10. then
View SolutionA parallel plate capacitor with air between the plates has a capacitance C. If the distance between the plates is doubled and the space between the plates is filled with a dielectric of dielectric constant 6, then the capacitance will become.
View SolutionIn a parallel plate capacitor, the capacitance
View Solution
