The length of a wire of a potentiometer is 100 cm, and the e.m.f. of its standard cell is E volt. It is employed to measure the e.m.f. of a battery whose internal resistance is 0.5Ω. If the balance point is obtained at I = 30 cm from the positive end, the e.m.f. of the battery is .
where i is the current in the potentiometer wire.
30E100.5
30E100−0.5
30(E−0.5i)100
30E100
The correct Answer is:D
To solve the problem, we need to find the e.m.f. of the battery using the information provided about the potentiometer and the balance point. Here’s a step-by-step solution:
Step 1: Understand the Setup
We have a potentiometer wire of length L=100cm and a standard cell with e.m.f. Evolts. The battery we want to measure has an internal resistance of r=0.5Ω, and the balance point is at l=30cm.
Step 2: Determine the Potential Drop Across the Potentiometer Wire
The potential drop across the entire potentiometer wire is proportional to its length. The potential drop across the 30 cm length of the potentiometer wire can be calculated as follows:
V=lL×E=30cm100cm×E=0.3E
Step 3: Relate the Potential Drop to the Battery's E.M.F.
At the balance point, the potential drop across the potentiometer wire (which is 0.3E) is equal to the e.m.f. of the battery plus the potential drop across its internal resistance. The current i flowing through the potentiometer wire can be expressed as:
V=Ebattery−i⋅r
Where Ebattery is the e.m.f. of the battery we want to find.
Step 4: Calculate the Current i
The current i can be determined using the relationship of the potential drop across the potentiometer wire. Since V=0.3E and the internal resistance r=0.5Ω, we can express the current as:
i=Vr=0.3E0.5=0.6E
Step 5: Substitute i Back into the Equation
Now we can substitute i back into the equation relating the e.m.f. of the battery:
0.3E=Ebattery−(0.6E⋅0.5)
0.3E=Ebattery−0.3E
Ebattery=0.3E+0.3E=0.6E
Final Answer
The e.m.f. of the battery is:
Ebattery=0.6Evolts
We have a potentiometer wire of length L=100cm and a standard cell with e.m.f. Evolts. The battery we want to measure has an internal resistance of r=0.5Ω, and the balance point is at l=30cm.
The potential drop across the entire potentiometer wire is proportional to its length. The potential drop across the 30 cm length of the potentiometer wire can be calculated as follows:
V=lL×E=30cm100cm×E=0.3E
At the balance point, the potential drop across the potentiometer wire (which is 0.3E) is equal to the e.m.f. of the battery plus the potential drop across its internal resistance. The current i flowing through the potentiometer wire can be expressed as:
V=Ebattery−i⋅r
Where Ebattery is the e.m.f. of the battery we want to find.
The current i can be determined using the relationship of the potential drop across the potentiometer wire. Since V=0.3E and the internal resistance r=0.5Ω, we can express the current as:
i=Vr=0.3E0.5=0.6E
Now we can substitute i back into the equation relating the e.m.f. of the battery:
0.3E=Ebattery−(0.6E⋅0.5)
0.3E=Ebattery−0.3E
Ebattery=0.3E+0.3E=0.6E
The e.m.f. of the battery is:
Ebattery=0.6Evolts
Topper's Solved these Questions
Similar Questions
A potentiometer wire has resistance of per unit length of 0.1Ω/m. A cell of e.m.f. 1.5V balances against 300cm length of the wire. Find the current in the potentiometer wire.
View SolutionA potentiometer wire has resistance of per unit length of 0.1Ω/m. A cell of e.m.f. 1.5V balances against 300cm length of the wire. Find the current in the potentiometer wire.
View Solution
Knowledge Check
The length of a wire of a potentiometer is 100 cm, and the emf of its stand and cell is E volt. It is employed to measure the emf of a battery whose internal resistance is 0.5Ω. If the balance point is obtained at l= 30 cm from the positive end, the emf of the battery is
A30E100.5B30E100−0.5C30(E−0.5i)100 wher I is the current in the potentiometer wireD30E100The length of a potentiometer wire is 200 cm and the e.m.f. of the battery connected to it is E. It is used to measure the e.m.f. of a cell whose internal resistance is 0.5Ω . If the balance point is obtained at l=40 cm from the positive end, then the e.m.f. of the cell is
A40E200B40E200+0.5C40(E−0.5)200D40E200−0.5AB is a potentiometer wire of length 100 cm and its resistance is 10 Ω. It is connected in series with a battery of emf 2V and resistance 40 Ω. If a source of unknown emf E is balanced by 40 cm length of the potentiometer wire, the value of E is :-
A0.8 VB1.6 VC0.08 VD0.16 V
In figure AB is a potentiometer wire of length 10 m and resistance 1Ω. The balancing length for 2V potential drop is 18 m. The e.m.f. of the battery E is
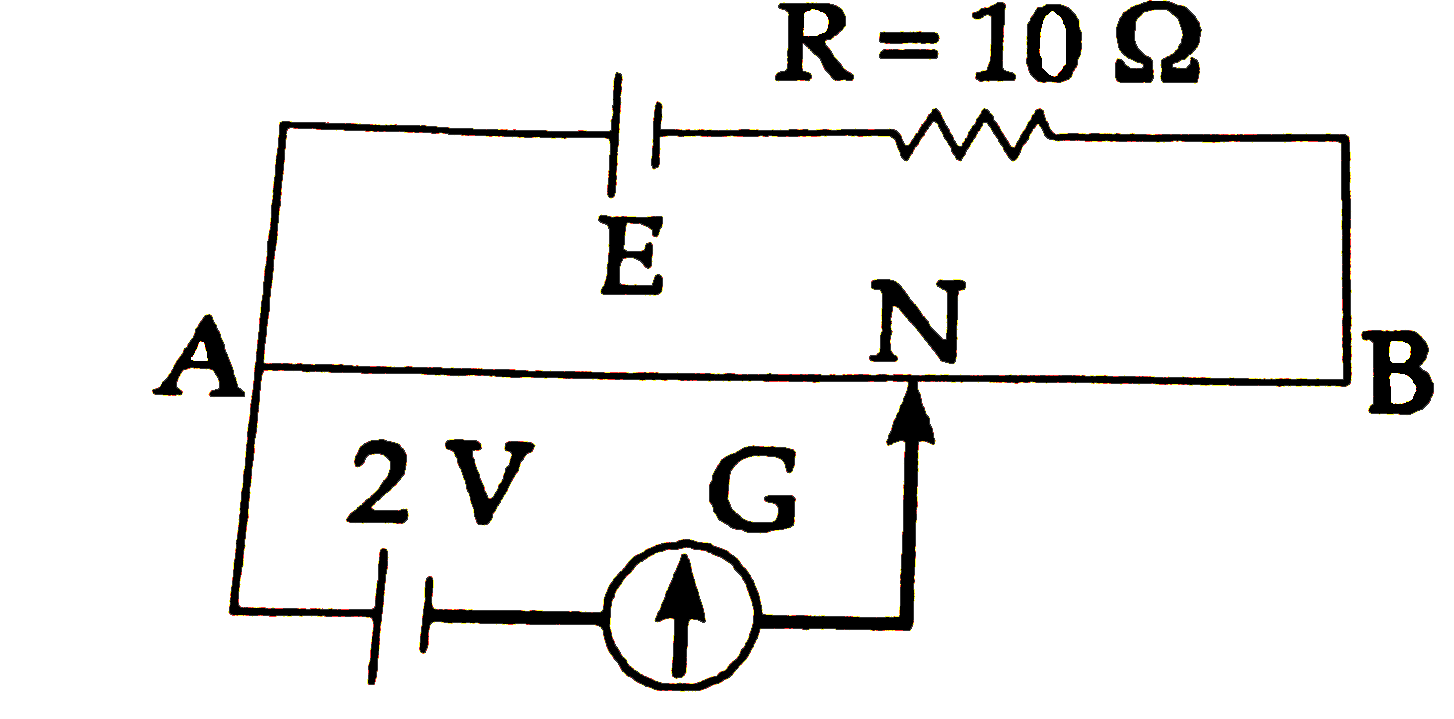
View SolutionA battery having e.m.f. 5 V and internal resistance 0.5Ω is connected with a resistance of 4.5Ω then the voltage at the terminals of battery is
View SolutionA battery having e.m.f. 5 V and internal resistance 0.5Ω is connected with a resistance of 4.5Ω then the voltage at the terminals of battery is
View SolutionThe e.m.f. of a standard cell balances across 150 cm length of a wire of potentiometer. When a resistance of 2Ω is connected as a shunt with the cell, the balance point is obtained at 100cm . The internal resistance of the cell is
View SolutionThe wire of potentiometer has resistance 4Ω and length 1m. It is connected to a cell of e.m.f. 2V and internal resistance 1Ω. The current flowing through the potentiometer wire is
View Solution