Monochromatic light is incident on a glass prism of angle A. If the refractive index of the material of the prism is μ, a ray, incident at an angle θ, on the face AB would get transmitted through the face AC of the prism provided:
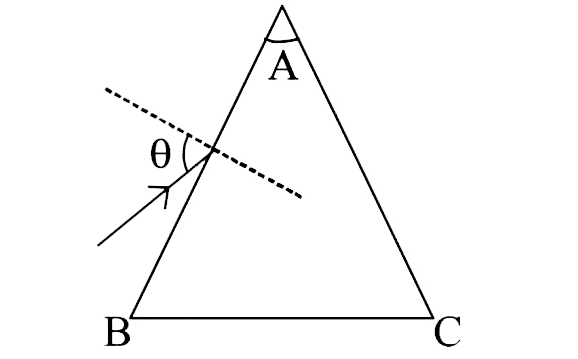
θ>cos−1[μsin(A+sin−1(1μ)]
θ<cos−1[μsin(A+sin−1(1μ)]
θ>sin−1[μsin(A−sin−1(1μ)]
θ<sin−1[μsin(A−sin−1(1μ)]
The correct Answer is:C
When r2=C ∠N2Rc=90∘
Where C=critical angle
As sinC=(1)/(v)=sinr_2<br>Apply∈gs≠ll'slawatR<br>musinr_2=1sin90^circ..(i)<br>Apply∈gs≠ll'slawatQ<br>1xxsintheta=musinr_1<br>Butr_1=A-r_2..(ii)<br>So,sintheta=mu sin (A-r_2)<br>sintheta=musinAcosr_2-cosA...(iii)[using(i)]<br>From(1)<br>cosr_2=sqrt(1-sin^2r_2)=sqrt(1-(1)/(mu^2))...(iv)<br>Byeq.(iii)and(iv)<br>sintheta=musinAsqrt(1-(1)/(mu^2))-cosA<br>onfurthersolv∈gwecanshowforray¬→transmiedthroughfaceAC<br>theta=sin^-1[usin(A-sin^(-1)((1)/(mu))]<br>So,fortransmissionthroughfaceAC<br>thetagtsin^(-1)[usin(A-sin^(-1)((1)/(mu))]

Topper's Solved these Questions
Similar Questions
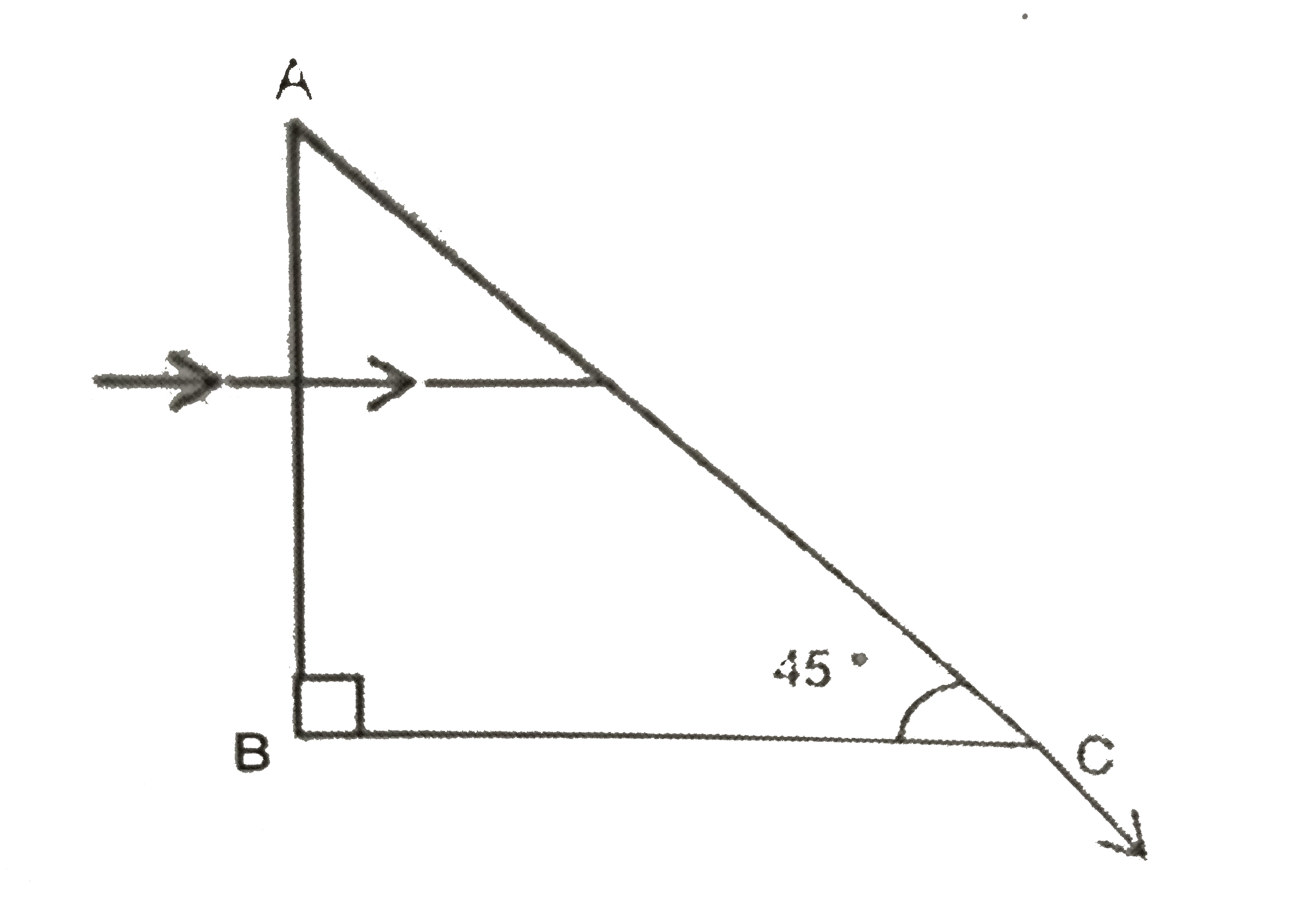
What is the refractive index of the material of the glass prism shown in the figure, if a ray of light incident normally at the face AB emerges along the face AC?
View SolutionThe refractive index of a prism is μ. Find the maximum angle of the prism for which a ray incident on it will be transmitted through other face without total internal reflection.
View Solution
Knowledge Check
A ray of light incident on a prism of angle 60∘, does not emerge out. Refractive index of material of prism may be
A1.50B2.30C1.80D1.20Refractive index of a prism is √7/3 and the angle of prism is 60∘. The minimum angle of incidence of a ray that will be transmitted through the prism is
A30∘B45∘C15∘D50∘A ray of monochromatic light is incident on one refracting face of a prism of angle 75∘. It passes through the prism and is incident on the other face at the critical angle. If the refractive index of the material of the prism is √2 , the angle of incidence on the first face of the prism is
A30∘B45∘C60∘D0∘
A ray of light is incident on a prism of refracting angle A. if θC is the critical angle for the material of the prism with respect to the surrounding air, then:
View SolutionA monochromatic light is incident at a certain angle on an equilateral triamgular prism and suffers minimum deviation . If the refractive index of the material of the prism is √3, them the angle of incidence is :
View SolutionA ray of monochromatic light is incident on one refracting face of a prism of angle 75∘. It passes through the prism and is incident on the other face at the critical angle. If the refractive index of the material of the prism is √2, the angle of incidence on the first face of the prism is
View SolutionA ray of monochromatic light is incident on one refracting face of a prism of angle 75∘. It passes thorugh the prism and is incident on the other face at the critical angle. If the refractive index of the material of the prism is √2, the angle of incidence on the first face of the prism is
View SolutionWhen light rays are incident on a prism at an angle of 45∘, the minimum deviation is obtained. If refractive index of the material of prism is √2, then the angle of prism will be
View Solution
