The probability mass function (p. m. f.) of X is given below :
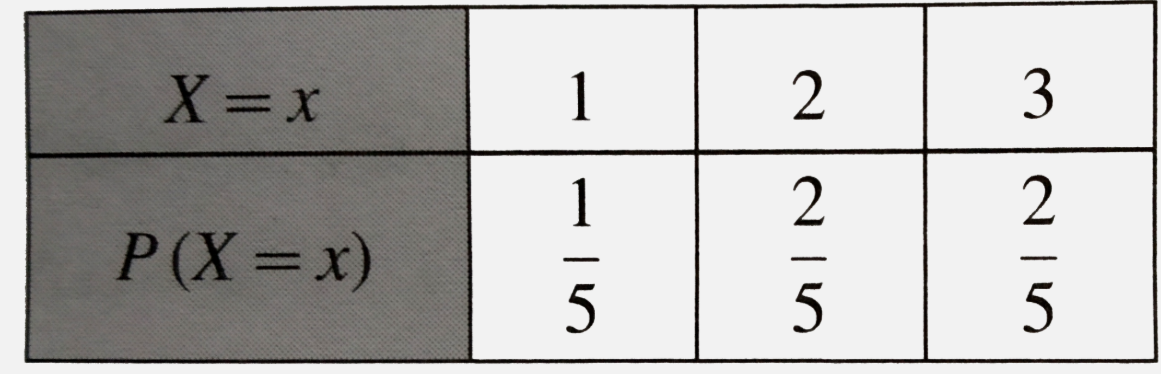
∣∣∣X=x123P(X=x)152525∣∣∣ find E(X2)
To find E(X2) using the given probability mass function (p.m.f.) of X, we will follow these steps:
Step 1: Identify the values of X and their corresponding probabilities
From the problem, we have:
- X=1 with P(X=1)=15
- X=2 with P(X=2)=25
- X=3 with P(X=3)=25
Step 2: Write the formula for E(X2)
The expected value E(X2) is calculated using the formula:
E(X2)=∑(x2⋅P(X=x))
where the summation is over all possible values of X.
Step 3: Substitute the values into the formula
Now we will substitute the values of X and their probabilities into the formula:
E(X2)=(12⋅P(X=1))+(22⋅P(X=2))+(32⋅P(X=3))
Substituting the values:
E(X2)=(12⋅15)+(22⋅25)+(32⋅25)
Calculating each term:
- For X=1: 12⋅15=1⋅15=15
- For X=2: 22⋅25=4⋅25=85
- For X=3: 32⋅25=9⋅25=185
Step 4: Sum the results
Now we sum these results:
E(X2)=15+85+185
Combining the fractions:
E(X2)=1+8+185=275
Final Answer
Thus, the expected value E(X2) is:
275
From the problem, we have:
- X=1 with P(X=1)=15
- X=2 with P(X=2)=25
- X=3 with P(X=3)=25
The expected value E(X2) is calculated using the formula:
E(X2)=∑(x2⋅P(X=x))
where the summation is over all possible values of X.
Now we will substitute the values of X and their probabilities into the formula:
E(X2)=(12⋅P(X=1))+(22⋅P(X=2))+(32⋅P(X=3))
E(X2)=(12⋅15)+(22⋅25)+(32⋅25)
- For X=1: 12⋅15=1⋅15=15
- For X=2: 22⋅25=4⋅25=85
- For X=3: 32⋅25=9⋅25=185
Now we sum these results:
E(X2)=15+85+185
Combining the fractions:
E(X2)=1+8+185=275
Thus, the expected value E(X2) is:
275
Topper's Solved these Questions
- BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - MARCH 2014EXERCISE - SECTION-II20videos
MARCH 2014
- BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - JULY 2018EXERCISE - SECTION II20videos
JULY 2018
- BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - MARCH 2015EXERCISE - SECTION-II20videos
MARCH 2015
Similar Questions
Knowledge Check
- Question 1 - Select One
If the probability mass function of a discrete random variable X is
P(x)=Cx3,x=1,2,3
=0, otherwise
Then E(X)=A343297B294251C297294D251294 - Question 2 - Select One
If the probability density function of a random variable X is f(x)=π2 in 0≤x≤2, then P(X>1.5X>1) is equal to
A716B34C712D2164 - Question 3 - Select One
The domain of the function f given by f(x) = x2+2x+1x2−5x+6
AR - { 3,-2}BR - {-3,2}CR - {3,-2}DR - {3,-2}
Find expected value of the random Variable X whose probability mass function is X=x,1,2,3<br>P(X=x)15,25,25
View SolutionFor the following probability distribution X→1,2,3,4,andP(x)→110,15,310,25 then E(X2) is equal to
View SolutionLet the p.m.f. ( probability mass function ) of random variable x be
P(x)=(4x)(59)x(4x)4−x,x=0,1,2,3,4
= 0 , otherwise
Find E(x) and Var.(x)View SolutionVerify whether the following functions can be regarded as the p.m.f for the given value of X :
(1) P(X=x)={x25x=0,1,20otherwise
(2) P(X=x){x−25x=1,2,3,40otherwiseView SolutionThe probability distribution of a random variable x is given as under
P(X=x)=⎧⎪⎨⎪⎩kx2x=1,2,32kxx=4,5,60,otherwise
where, k is a constant. Calculate
(i) E(X) (ii) E(3X2) (iii) P(X≥4)View Solution