Minimize z=6x+4y, subject to
3x+2y≥12,x+y≥5,0≤x≤4,0≤y≤4.
Video Solution
Text Solution
First we draw the line AB and CD whose equations are :
3x+2y=12
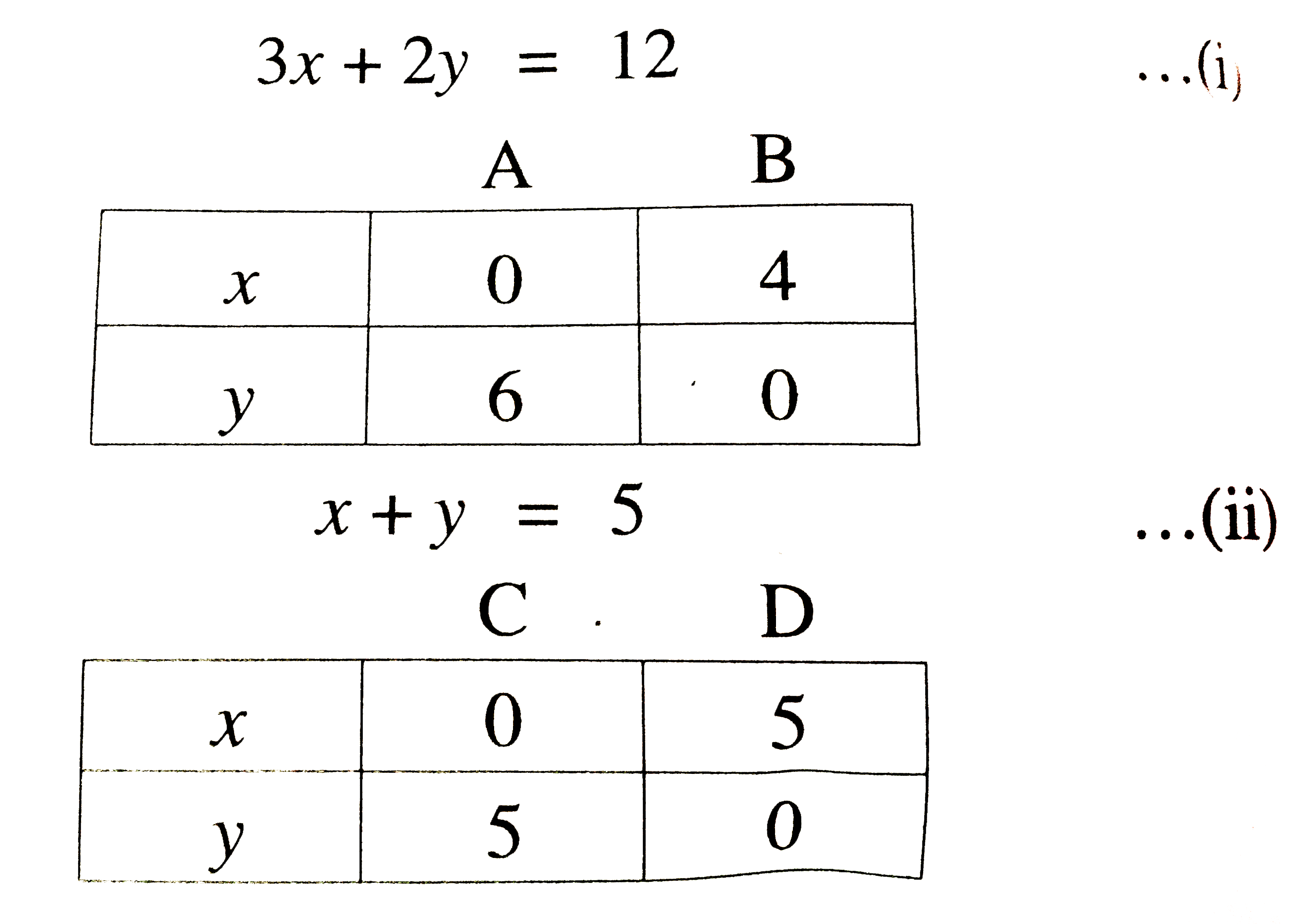
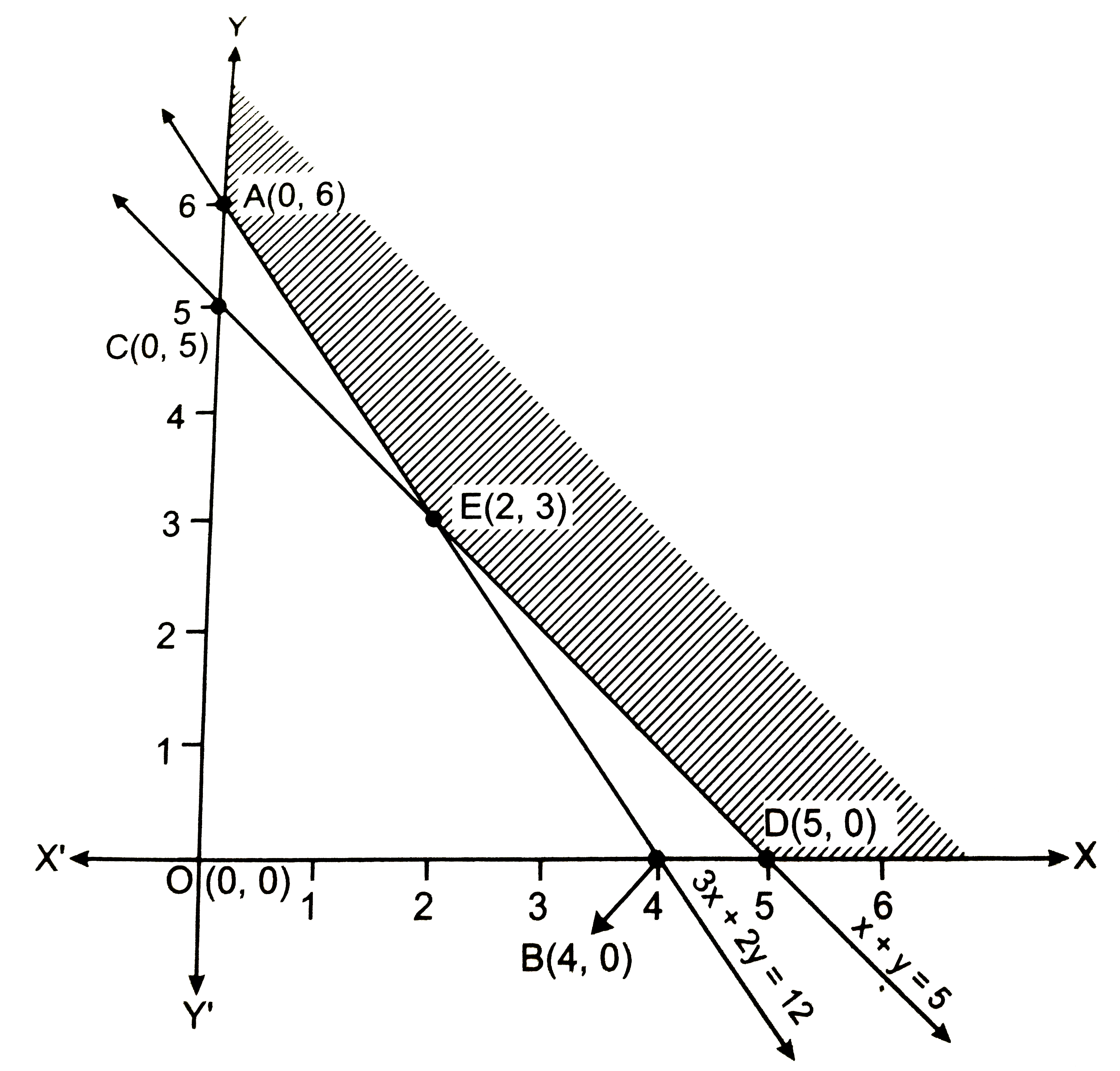
The feasible region is XDEAY which is shaded in the figure.
The vertices of the feasible region are A (0,6) and D (5,0).
E is the point of intersection of the lines . Now solving equation (i) and (ii) we get intersecting point E (2,3). Origin do not satisfied given condition (0,0) does not present in under lined area. Calculation of minimum value :
Corner points Z=6x+4yAtA(0,6)Z=6×0+4×6=24AtE(2,3)Z=6×2+4×3=24AtD(5,0)Z=6×5+4×0=30
Since Z has minimum value 24 at two consecutive vertices A and E of the feasible region , Z has minimum value 24 at every point of segment AE. Hence there are infinite number of optimal solutions.
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Minimise Z=−3x+4y
subject to x+2y≤8,3x+2y≤12,x≥0,y≥0.View SolutionMinimize z=6x+2y, subject to 5x+9y≤90,x+y≥4,y≤8,x≥0,y≥0.
View Solutionx+y≤5,4x+y≥4,x+5y≥5,x≤4,y≤3.
View SolutionMaximize z=4x+6y,subject to
3x+2y≤12,x+y≥4,x≥0,y≥0View SolutionMaximize z=10x+25y, subject to x≤3,y≤3,x+y≤5,x≥0,y≥0.
View SolutionMaximize z=6x+4y, subject to
2x+3y≤30,3x+2y≤24,x+y≥3,x≥0,y≥0.View Solutionx+2y≤10,x+y≥1,x−y≤0,x≥0,y≥0
View SolutionMinimize z=x+3y
Subject to
x+y≤5
2x+y≥4
x+5y≥5
x≥3
y≤3View SolutionMaximize z=4x+5y, subject to
2x+y≥7,2x+3y≤15,x≤3,x≥0,y≥0View SolutionMinimize Z=3x+2y subject to x+y≤8,x,y≥0.
View Solution

