Prove theoretically the relation between e.m.f. induced in a coil and rate of change of magnetic flux in electromagnetic induction. A parallel plate air condenser has a capacity of 20 $μ F$ . What will be the new capacity if:
(a) the distance between the two plates is doubled ?
(b) A marble slab of dielectric constant 8 is introduced between the two plates ?

Text Solution

Verified by Experts

Consider a rectangular loop of conducting wire PQRS partly placed in uniform magnetic field of induction 'B' which is perpendicular to the plane of paper and directed into the paper.

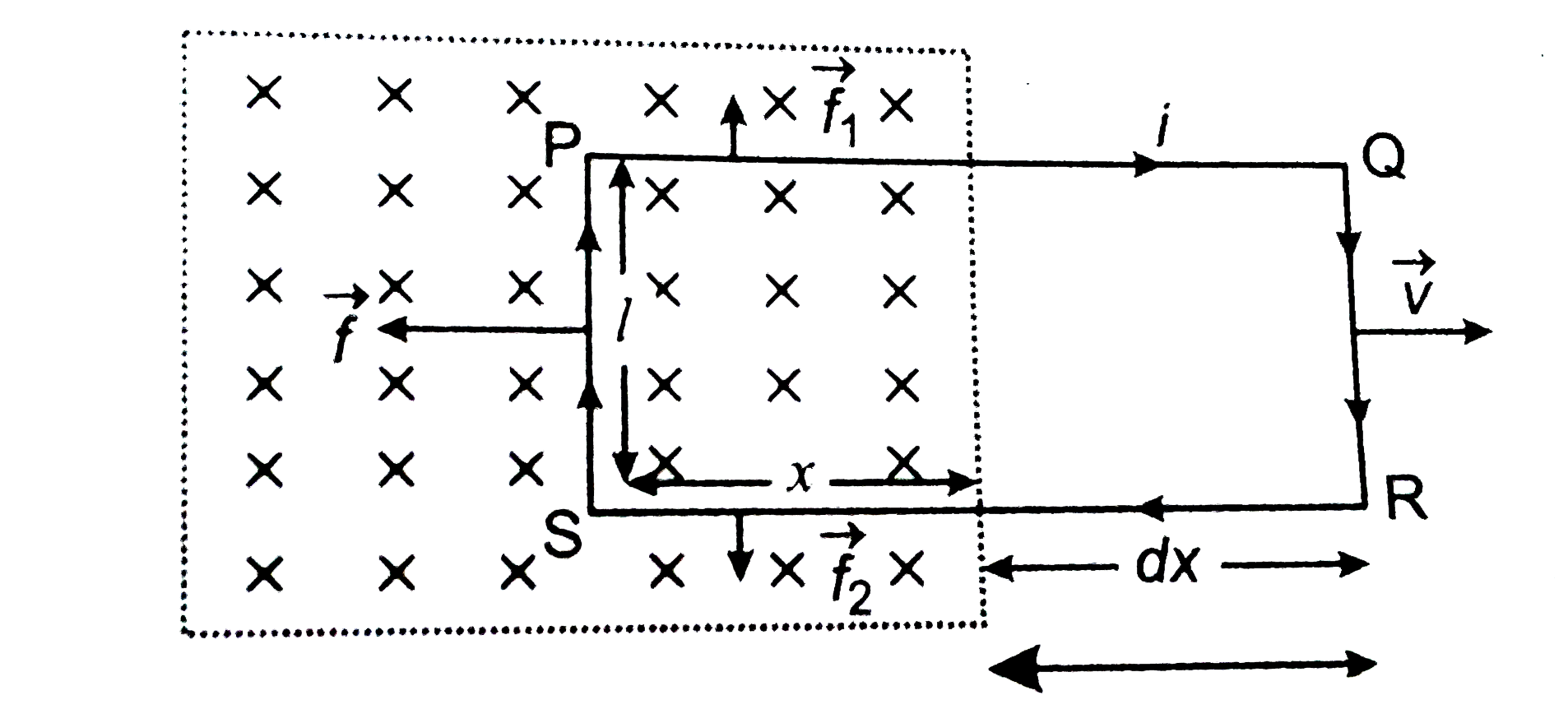
Let $l$ be the length of the side PS and x be the length of the loop within hte field.
Therefore, $A = l x$ , area of that loop which lies inside the field.
The magnetic flux $(ϕ)$ through the area A at certain time 't' is $ϕ = B A = B l x .$
The loop is pulled out of the magnetic field of induction 'B' to the right with a uniform velocity $\to v$ .
The rate of change of magnetic flux,
$\frac{d ϕ}{d t} = \frac{d}{d t} (B l x)$
$∴ \frac{d ϕ}{d t} = B l (\frac{d x}{d t})$
But, $v = (\frac{d x}{d t})$
$∴ \frac{d ϕ}{d t} = B l v$ ...(i)
Due to change in magnetic flux, induced current is set up in the coil. The direction of this current is clockwise according to Lenz's law. Due to this the sides of the coil experiences the forces $f_{1}, f_{2} and f .$
The magnitude of force $f$ acting on the side PS is
$f = B i l (∵ \to f = i \to l \times \to B)$
Since, ${\to f}_{1} and {\to f}_{2}$ are equal and opposite, so they cancel out.
The unbalanced force which opposes the motion of the coil is $f$ . Hence, the external agent like hand must do work against this force in order to pull the coil.
The work done in time dt during displacement dx is
$d W = - f d x$
(Negative sign shows that $\to f and - \to d x$ are opposite to each other)
$∴ d W = - (B i l) d x$ ...(ii)
The work done must be equal to the electrical energy generated. If e is the induced e.m.f. and i is the induced current,
Electric work $= \frac{d W}{d t} = e i$
$d W = e$ ...(iii)
Equating equations (ii) and (iii), we get
$e = - B i l d x$
$∴ e = - B l (\frac{d x}{d t})$
$∴ e = - B l v$
Using equation (i),
$e = \frac{d ϕ}{d t}$
Numerical :
(a) Given, $C = 20 μ F .$
We know,
Capacitance, $C = \frac{K a ε_{0}}{d}$
For air, $K = 1$
$∴ C = \frac{A ε_{0}}{d}$ ...(i)
Then,
New capacitance, $C' = \frac{A ε_{0}}{d'}$
$∴ d' = 2 d$
$= \frac{A ε_{0}}{2 d}$
$= \frac{C}{2}$ [From equation (i)]
$= \frac{20}{2}$
$= 10 μ F$
So, if the distance between the plates is doubled capacity of condenser becomes $10 μ F$ .
(b) When K = 8
$∴ C'' = \frac{K' A ε_{0}}{d} = 8 \frac{A ε_{0}}{d}$
$= 8 C [∵ \frac{A ε_{0}}{d} = C]$
$= 8 \times 20$
$= 160 μ F$ [From equation (i)]
If a slab of dielectric constant 8 is introduced between the plates, the capacity becomes 8 times i.e., $160 μ F .$

Updated on:21/07/2023

The capacity of a paralel plate condenser is $12 μ F$ . What is its new capacity if the separation between the plates is doubled and the area is halved?
A $1.5 μ F$
B $3 μ F$
C $6 μ F$
D $8 μ F$
A parallel plate condenser is connected with the terminals of a battery. The distance between the plates is $6 m m$ . If a glass plate (dielectric constant $K = 9)$ of $4.5 m m$ is introduced between them, then the capacity will become
A $2$ times
Bthe same
C $3$ times
D $4$ times
The distance between the plates of a parallel plate condenser is $8 m m$ and $P . D . 120$ volts. If a $6 m m$ thick slab of dielectric constant $6$ is introduced between its plates, then
Athe charge on the condenser will be doubled
Bthe charge on the condenser will be reduced to half
Cthe $P . D .$ across the condenser will be $320$ volts
Dthe $P . D .$ across the condenser will be $45$ volts