When a resistance of 12 ohm is connected across a cell, its terminal potential difference is balanced by 120 cm length of potentiometer wire. When the resistance of 18 ohm is connected across the same cell, the balancing length is 150 cm. Find the balancing length when the cell is in open circuit. Also calculate the internal resistance of the cell.
To solve the problem, we need to find the balancing length when the cell is in open circuit and also calculate the internal resistance of the cell using the given data.
Given:
1. When a resistance of R1=12Ω is connected, the balancing length L1=120cm.
2. When a resistance of R2=18Ω is connected, the balancing length L2=150cm.
Step 1: Set up the equations
The potential difference across the cell when a resistance is connected can be expressed in terms of the balancing lengths and resistances as follows:
ER+r=Lk
Where:
- E is the EMF of the cell.
- R is the external resistance.
- r is the internal resistance of the cell.
- L is the balancing length.
- k is a constant that relates the length of the potentiometer wire to the potential difference.
From the two scenarios, we can set up two equations:
1. For R1=12Ω:
E12+r=120k
2. For R2=18Ω:
E18+r=150k
Step 2: Rearranging the equations
From the first equation, we can express E:
E=120(12+r)k
From the second equation, we can express E as well:
E=150(18+r)k
Step 3: Equate the two expressions for E
Setting the two expressions for E equal to each other:
120(12+r)k=150(18+r)k
Step 4: Simplifying the equation
Since k is common on both sides, we can cancel it out:
120(12+r)=150(18+r)
Expanding both sides:
1440+120r=2700+150r
Step 5: Rearranging to find r
Rearranging gives:
1440−2700=150r−120r
−1260=30r
r=−126030=−42Ω
Step 6: Finding the open circuit length
Now we can find the balancing length when the cell is in open circuit. Using the first equation:
Er=L0k
Where L0 is the balancing length in open circuit.
Using E=120(12+r)k and substituting r:
L0=E⋅kr=120(12−42)−42
Calculating:
L0=120⋅(−30)−42=360042≈85.71cm
Final Results:
1. The balancing length when the cell is in open circuit is approximately 85.71cm.
2. The internal resistance of the cell is 42Ω.
1. When a resistance of R1=12Ω is connected, the balancing length L1=120cm.
2. When a resistance of R2=18Ω is connected, the balancing length L2=150cm.
The potential difference across the cell when a resistance is connected can be expressed in terms of the balancing lengths and resistances as follows:
- E is the EMF of the cell.
- R is the external resistance.
- r is the internal resistance of the cell.
- L is the balancing length.
- k is a constant that relates the length of the potentiometer wire to the potential difference.
E12+r=120k
E18+r=150k
From the first equation, we can express E:
E=120(12+r)k
E=150(18+r)k
Setting the two expressions for E equal to each other:
120(12+r)k=150(18+r)k
Since k is common on both sides, we can cancel it out:
120(12+r)=150(18+r)
1440+120r=2700+150r
Rearranging gives:
1440−2700=150r−120r
−1260=30r
r=−126030=−42Ω
Now we can find the balancing length when the cell is in open circuit. Using the first equation:
Er=L0k
Where L0 is the balancing length in open circuit.
L0=E⋅kr=120(12−42)−42
Calculating:
L0=120⋅(−30)−42=360042≈85.71cm
1. The balancing length when the cell is in open circuit is approximately 85.71cm.
2. The internal resistance of the cell is 42Ω.
Similar Questions
When a resistor of 5Ω is connected across a cell, its terminal potential differnce is balanced by 140 cm of potentiometer wire and when a resistance of 8Ω is connected across the cell, the terminal potential difference is balanced by 160 cm of the potentiometer wire. Find the internal resistance of the cell.
View SolutionWhen a resistor of 5Ω is connected across a cell, its terminal potential differnce is balanced by 140 cm of potentiometer wire and when a resistance of 8Ω is connected across the cell, the terminal potential difference is balanced by 160 cm of the potentiometer wire. Find the internal resistance of the cell.
View Solution
Knowledge Check
The emf of cell is balanced by balancing length 450 cm . When the resistance of 10Ω is connected across the cell, balancing length changes by 100 cm. The internal resistance of a cell is
A1.8ΩB2.8ΩC3.8ΩD2.5ΩCell of emf 1 V is connected across a potentiometer, balancing length is 600 cm. What will be the balancing length for 25 V?
A400 cmB600 cmC1500 cmD1200 cmA cll balances against a length of 150 cm on a potentiometer wire when it is shunted by a resistance of 5Ω. But when it is shunted by a resistance of 10Ω, the balancing length increases by 25 cm. What is the balancing length when the cell is in an open circuit ?
A200 cmB210 cmC225 cmD250 cm
The e.m.f. of a standard cell balances across 150 cm length of a wire of potentiometer. When a resistance of 2Ω is connected as a shunt with the cell, the balance point is obtained at 100cm . The internal resistance of the cell is
View SolutionA cell of e.m.f. 1.2 V is balanced by 150 cm of potentiometer wire. When the cell is shunted by a resistance of 4Ω, the balancing length is reduced by 30 cm. What is the internal resistance of the cell ?
View SolutionIn the given figure, battery E is balanced on 55 cm length of potentiometer wire but when a resistance of 10Ω is connected in parallel with the battery then it balances on 50 cm length of the potentiometer wire then internal resistance r of the battery is
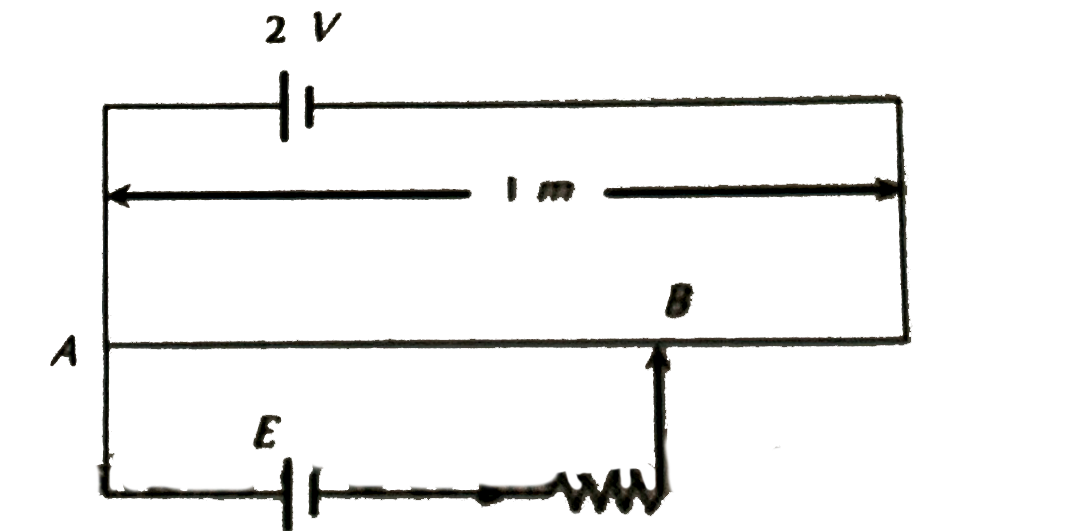
View SolutionIn a potentiometer experiment, the balancing length with a cell is 560cm. When an external resistance of 10ohms is connected in parallel to the cell the balancing length changs by 60cm. The internal resistance of the cell in ohm is
View SolutionA cell of emf E and internal resistance r connected in the secondary gets balanced against length l of potentiometer wire. If a resistance R is connected in parallel with the cell, then the new balancing length for the cell will be
View Solution
