A stone of mass 100 g attached to a string of length 50 cm is whirled in a vertical circle by giving it a velocity of 7 m/s at the lowest point. Find the velocity at the highest point .
Given :
mass of stone, m=100gm=100×10−3kg
length of string, l=50cm=0.5m
Velocity of mass in a vertical circle at lowest point,
VL=7m/sec
Acceleration due to gravity.
g=9.8m/sec2
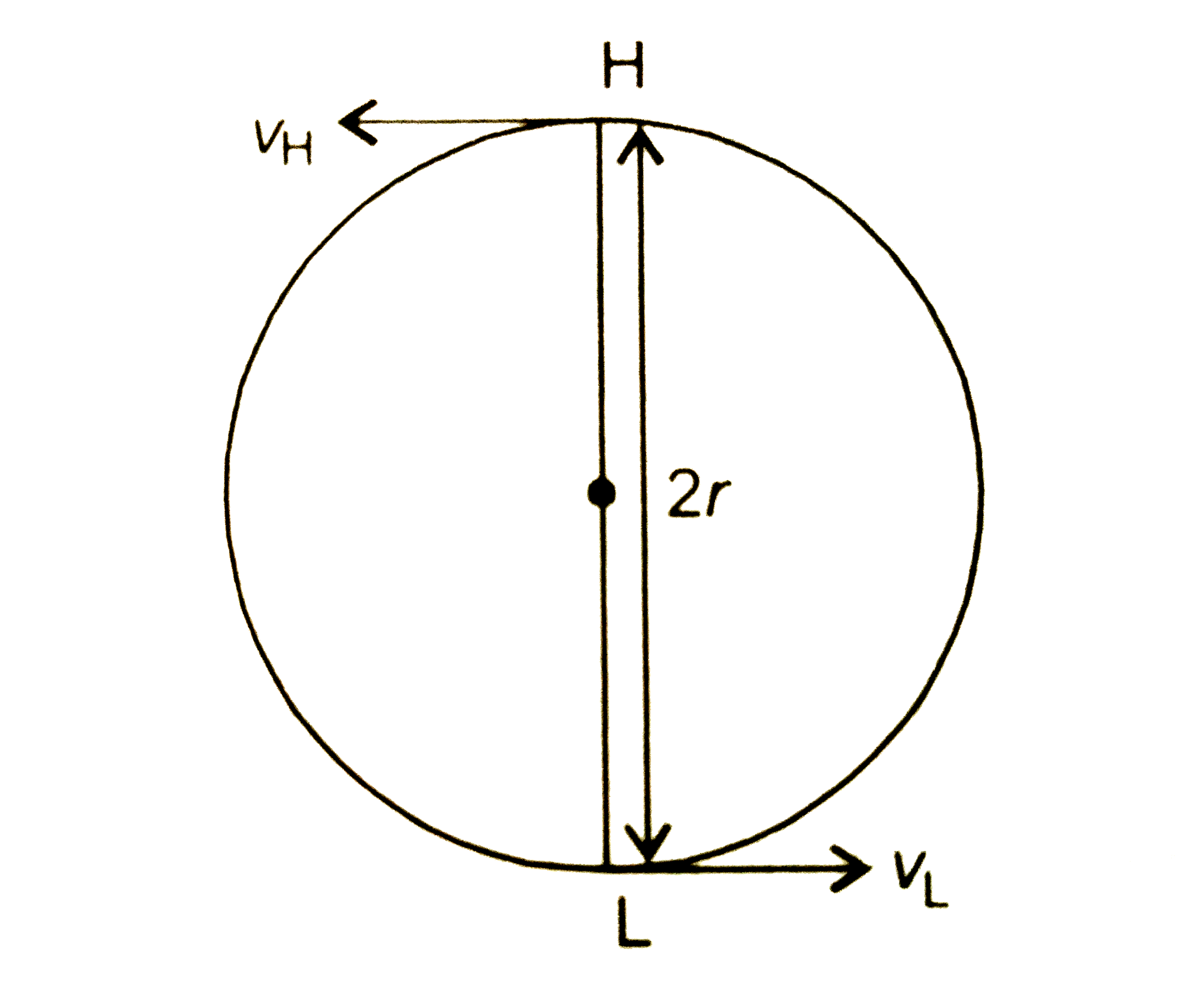
According to law of conversation of energy,
Total energy at L= Total energy at H
(K.E.)L+(P.E.)L=(K.E.)H+(P.E.)H
At lowest point, P.E.=0
K.E.=12mv2L
At highest point, P.E.=mg(2r)
K.E.=12mv2H
Putting the values in equation (i), we get
12mv2L+0=12mv2H+mg(2r)
v2L=v2H+4gr
72=v2H+4×9.8×0.5
v2H=49−4×4.9
vH=5.422m/sec.
Topper's Solved these Questions
- OCTOBER 2015BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - OCTOBER 2015EXERCISE - SECTION-2
21 Videos
- OCTOBER 2015BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - OCTOBER 2015EXERCISE - SECTION-2
21 Videos
- MARCH 2017BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - MARCH 2017EXERCISE - SECTION II
21 Videos
- SEPTEMBER 2014BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - SEPTEMBER 2014EXERCISE - SECTION-2
21 Videos
Similar Questions
A stone of mass 100 g attached to a string of length 50 cm is whirled in a vertical circle by giving it a velocity of 7 m/s at the lowest point. Find the velocity at the highest point .
View SolutionA stone of mass 5kg, tied to one end of a rope of length 0.8m, is whirled in vertical circle. Find the minimum velocity at the highest point and at the midway point [g=9.8m/s2]
View Solution
Knowledge Check
A stone of mass 250 gram , attached at the end of a string of length 1.25 m is whirled in a horizontal circle at a speed of 5 m/s . What is the tension in the string ?
A2.5NB5NC6ND8NA body of mass 100 gram is tied at the end of a string of length 1m. It is rotated in a vertical circle at a critical speed of 4 m/s at the highest point . The tension in the string at the huighest point in its path is [ take g=10m/s2]
A0.3NB0.6NC0.9ND1.2NA stone of mass 0.5 kg is attached to a string of length 2m and is whirled in a horizontal circle. If the string can withstand a tension of 9N, the maximum velocity with which the stone can be whirled is
A6ms−1B8ms−1C4ms−1D12ms−1
A child revolves a stone of mass 0 .5 kg tied to the end of a string of length 40 cmin a vertical circle . The speed of the stone at the lowest point of the circle is 3m/s Calculate tension in the string at this point.
View SolutionA body of mass m is tied to a string of length l and whirled in a verticle cirlce. The velocity of the body at the lowest position is u. Then the tension in the string at a position when the string makes an angle θ with the vertical is
View SolutionA stone of mass 1kg is tied with a string and it is whirled in a vertical circle of radius 1 m. If tension at the highest point is 14 N, then velocity at lowest point will be
View SolutionA stone of mass 0.5kg is attached to a string of length 2m and is whirled in a horizontal circle. If the string can withstand a tension of 9N the maximum velcoity with which the stone can be whirled is .
View SolutionA stone of mass 1 kg tied at the end of a string of length 1 m and is whirled in a verticle circle at a constant speed of 3ms−1. The tension in the string will be 19 N when the stone is (g=10ms−1)
View Solution
