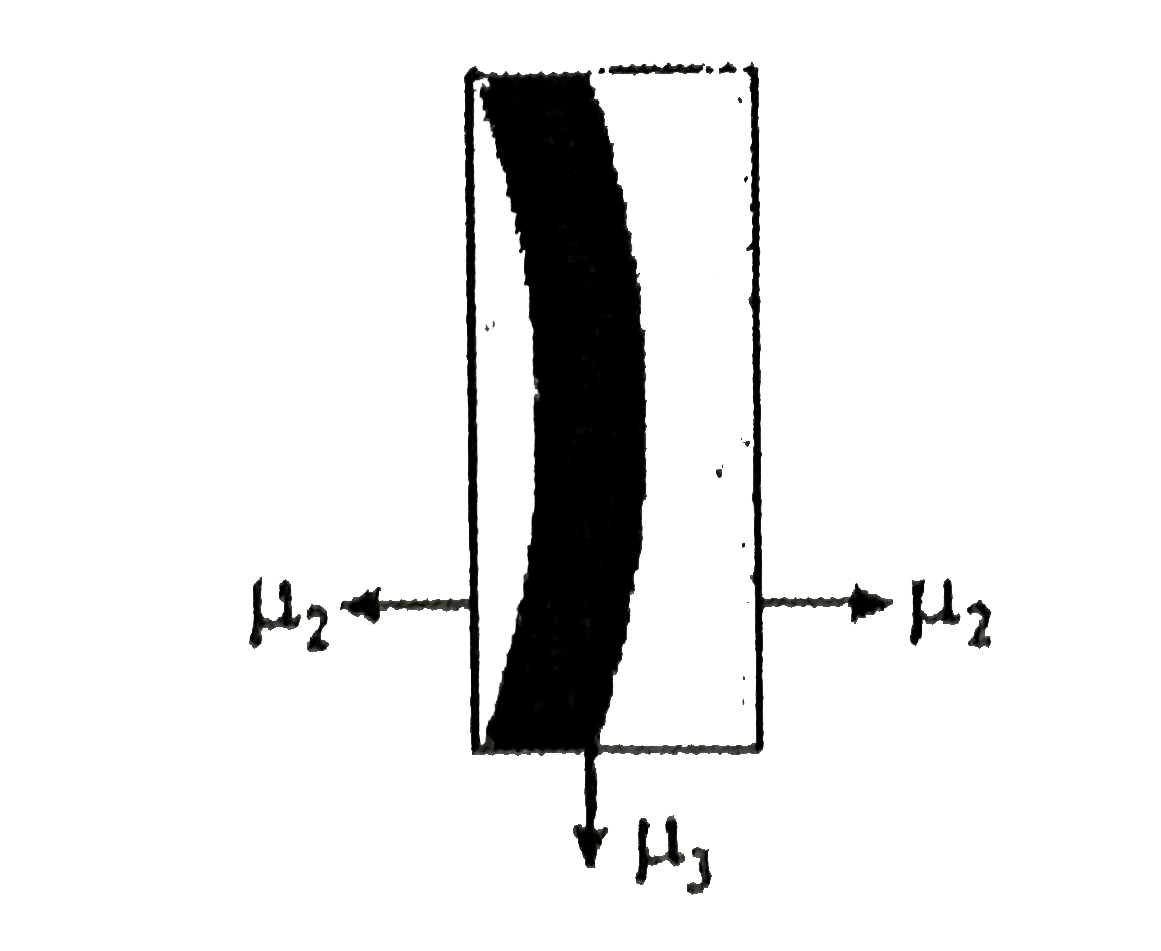
The adjacent figure shows a thin plano-convex lens of refractive index μ1 and a thin plano-concave lens of refractive index μ2, both having same radius of curvature R of their curved surfaces. The thin lens of refractive index μ3 has radius of curvature R of both its surfaces. This lens is so placed in between the plano-convex and plano-concave lenses that the plane surfaces are parallel to each other.
The focal length of the combination is

R(μ1+μ2−μ3−1)
R(μ1+μ2+μ3)
R(μ1−μ2)
R(μ1−μ2−μ3−3)
The correct Answer is:C
1f1=(μ1−1)(1∞+1R)=μ1−1R
⇒1f1=(μ3−1)(−1R+1R)=0
1f3=(μ2−1)(−1R−1∞)=−(μ2−1)R
1f=1f1+1f2+1f3=(μ1−1)R−(μ2−1)R−1R(μ1−μ2)
⇒f=R(μ1−μ2)
Topper's Solved these Questions
Similar Questions
The figure below shows a thin plano-convex lens of refractive index μ1 and a thin plano-concave lens of refractive index μ2, both having same radius of curvature R of their curved surfaces. Another thin lens of refractive index μ3 has same radius of curvature R on the two surface between the plano-convex and plano-concae lenses that the plane surfaces are parallel to each other. Find the focal length of the combination.
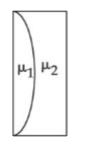
View SolutionCurved surfaces of a plano-convex lens of refractive index μ1 and a plano-concave lens of refractive index μ2 have equal radius of curvature as shown in figure. Find the ratio of radius of curvature to the focal length of the combined lenses.
View Solution
Knowledge Check
A plano-convex lens is made of refractive index 1.6. The radius of curvature of the curved surface is 60 cm. The focal length of the lens is
A200 cmB100 cmC50 cmD400 cmA plano-convex lens is made of glass of refractive index 1.5. the radius of curvature of its convex surface is R. its focal length is
AR2BRC2RD1.5RThe refractive index and the focal length of a plano convex lens are 1.5 and 50 cm respectively. What is the radius of curvature of the curved surface ?
A10 cmB15 cmC20 cmD25 cm
A plano-concave lens is made of glass of refractive index 1.5 and the radius of curvature of its curved face is 100 cm. What is the power of the lens?
View SolutionA concave lens of glass, refractive index 1.5, has both surface of same radius of curvature R. On immersion in a medium of refractive index 1.75, it will have as a
View SolutionA plano-convex lens is made of glass of refractive index 1.6. the radius of curvature of its curved surface is 30 cm. What is the focal length of the lens ?
View SolutionA plano-convex lens is made of glass of refractive index 1.5. The radius of curvature of its convex surface is R. Its focal length is
View SolutionA concave lens of glass, refractive index 1.5 has both surfaces of same radius of curvature R. On immersion in a medium of refractive index 1.75, it will behave as a
View Solution