If f(x)= kx, 0 lt x lt 2 = 0 otherwise, is a probability density function of a random variable X , then find (i) value of k, (ii) P(1 lt x lt2).
To solve the problem, we need to follow these steps:
Step 1: Determine the value of k
Since f(x) is a probability density function (PDF), the integral of f(x) over its range must equal 1. The function is defined as:
f(x)={kxfor 0<x<20otherwise
We need to integrate f(x) from 0 to 2:
∫20f(x)dx=∫20kxdx=1
Calculating the integral:
∫20kxdx=k∫20xdx=k(x22)20=k(222−022)=k(42)=2k
Setting this equal to 1:
2k=1
Solving for k:
k=12
Step 2: Find the probability P(1<x<2)
Now that we have k, we can express f(x) as:
f(x)=12xfor 0<x<2
To find P(1<x<2), we need to integrate f(x) from 1 to 2:
P(1<x<2)=∫21f(x)dx=∫2112xdx
Calculating the integral:
∫2112xdx=12∫21xdx=12(x22)21=12(222−122)
Calculating the limits:
=12(42−12)=12(2−12)=12(42−12)=12(32)=34
Final Answers
(i) The value of k is 12.
(ii) The probability P(1<x<2) is 34.
---
Topper's Solved these Questions
Similar Questions
Show that the function f(x) defined by .f(x)=17, for 1≤x≤8 =0, otherwise, is a probability density function for a random variable. Hence find P(3<X<10)
View SolutionThe following is a probability distribution function of a random variable :
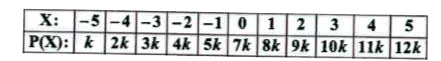
(i) Find k (ii) Find P(X>3) (iii) Find P(−3<X<4)
(iv) Find P(X<−3).
View Solution
Knowledge Check
The p.d.f. of a random variable X is
(f)x=2x,0≤x≤1
=0, otherwise
Then the value of P(13<X<12) isA136B536C736D1136If the function f(x)=x23,−1<x<2
= 0 , otherwise is a p.d.f of X, then P(X<0) isA19B29C39D49The p.d.f. of a random variable X is
(f)x=15,0≤x≤5
=0, otherwise
Then the value of P(1<X<3) isA15B25C35D45
Find k, if the function f defined by f(x)=kx,0<x<2 =0 , otherwise is the p.d.f of a random variable X.
View SolutionThe p. d. f. of a continuous r. v. Xis
f(x)=3x280<x<20otherwise
Determine the c.d.f of X and hence find
(i) P(X<1),(ii)P(1<X<2).
View SolutionIf f(x)=kx(1−x), for 0<x<1=0 , otherwise , is the p.d.f. of a r. v. X then k = 12 . True or False.
View SolutionThe following is the p.d.f (Probability Density Function ) of a continous random variable X :
f(x)=x32,0<x<8
= 0 , otherwise
Also, find its value at x = 0.5 and 9.
View SolutionThe following is the p.d.f (Probability Density Function ) of a continous random variable X :
f(x)=x32,0<x<8
= 0 , otherwise
Find the expression for c.d.f (Cumulative Distribution Function ) of X
View Solution
