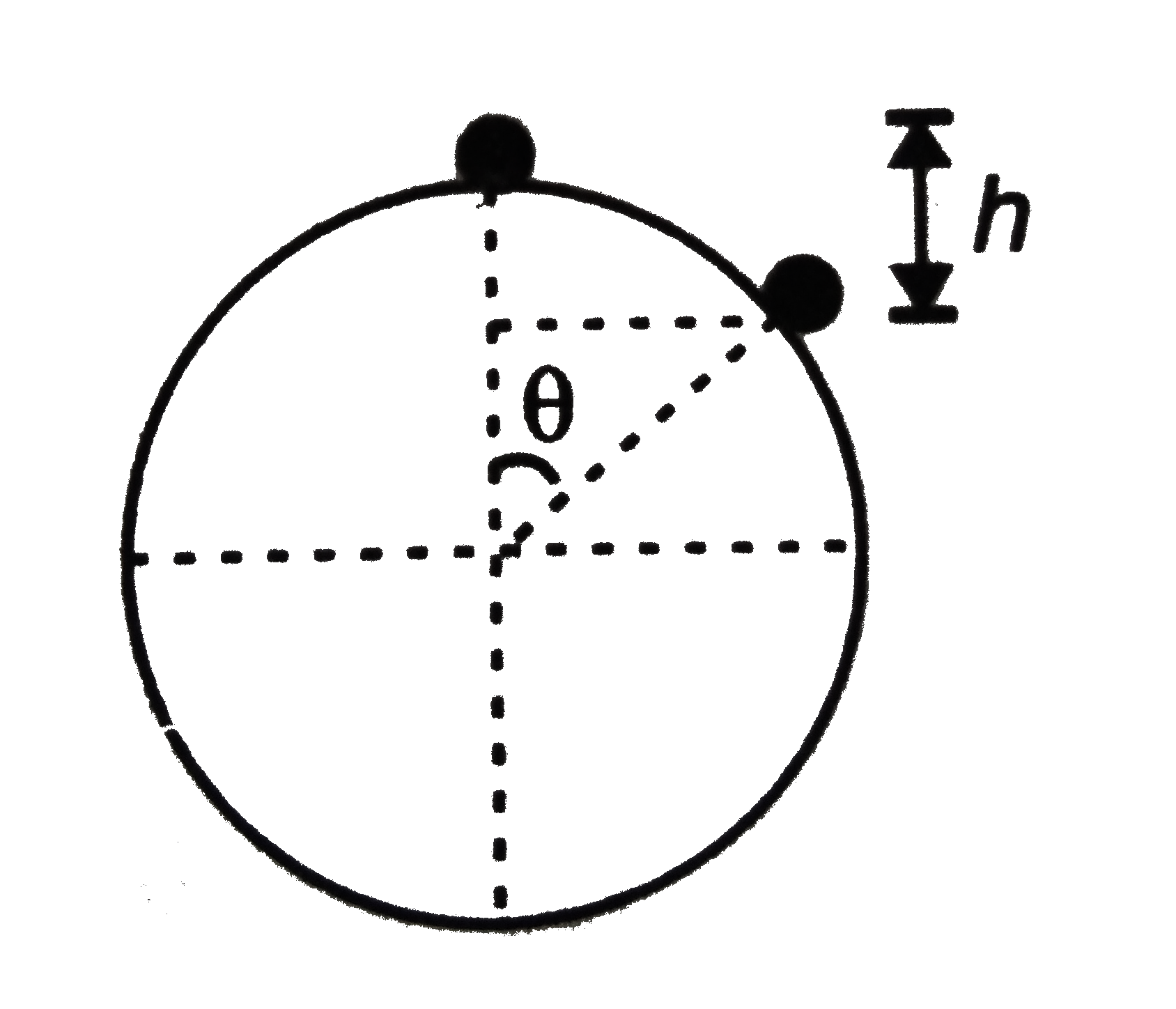
A small mass m starts from rest and slides down the smooth spherical surface of F. Assume zero potential energy at the top. Find
(a) the change in potential energy,
(b) the kintic energy,
(c) the speed ot the mass as a function of the angle made by the radius through the mass with the vertical.
, ,
, ,
, ,
, ,
The correct Answer is:A
In the figure,
(a) As the mass comes down, potential energy will decrease. Hence,
(b) Magnitude of decrease in potential energy = increase in kinectic energy
Kinetic energy
(c)
.
 .
.
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Exercise 9.110videos
WORK, ENERGY & POWER
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Exercise 9.29videos
WORK, ENERGY & POWER
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - TYPE21videos
WORK, ENERGY & POWER
- BOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question11videos
WAVE MOTION
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY33videos
WORK, ENERGY AND POWER
Similar Questions
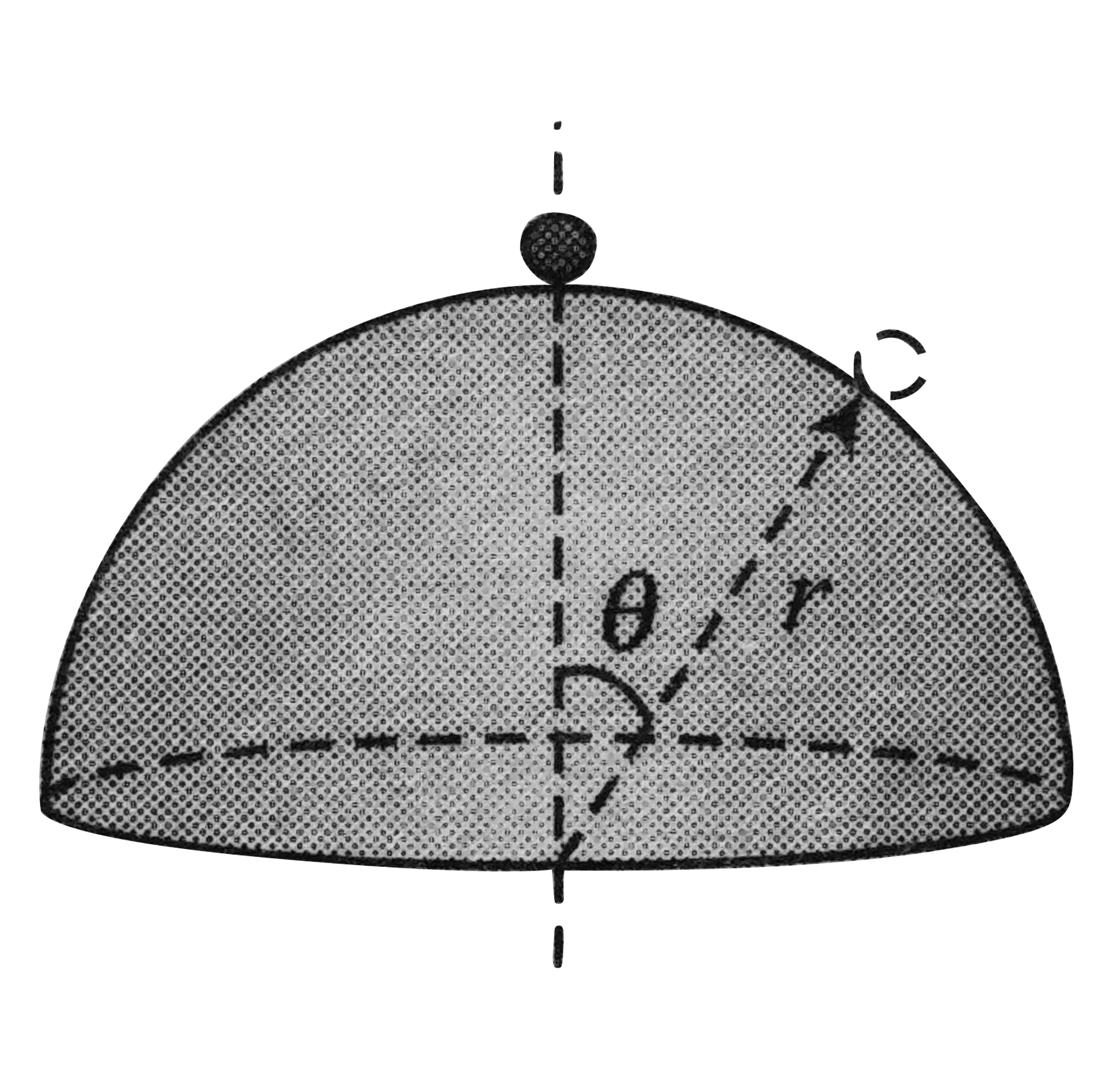
A point mass m starts from rest and slides down the surface of a frictionless hemisphere of radius r as shown figure. Measure angle from the vertical and potential energy from the top. Find
a. Find the changes in potential energy of the mass with angle
b. Find the kinetic energy as a function of angle
c. Find the radial and tangential acceleration as a function of angle
d. Find the angle at which the mass files off the hemisphere
e. If there is friction between the mass and hemisphere, does the mass fly off at a greater or lesser angle than in part (d) ?
 View Solution
View SolutionA point mass m starts from rest and slides down the surface of a frictionless hemisphere of radius r as shown figure. Measure angle from the vertical and potential energy from the top. Find
a. Find the changes in potential energy of the mass with angle
b. Find the kinetic energy as a function of angle
c. Find the radial and tangential acceleration as a function of angle
d. Find the angle at which the mass files off the hemisphere
e. If there is friction between the mass and hemisphere, does the mass fly off at a greater or lesser angle than in part (d) ?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A body of mass m is lifted up from the surface of earth to a height three times the radius of the earth R. The change in potential energy of the body is
A3mgRB54mgRC34mgRD2mgR - Question 2 - Select One
A mass is taken from surface to a height h. The change in potential energy in this process is equal to the change in potential energy if it is now taken from that point to infinity. What is the value of h?
Ah=RBh=2RCh=3R2Dh=4R - Question 3 - Select One
The change in the gravitational potential energy when a body of mass m is raised to a height nR above the surface of the earth is (here R is the radius of the earth)
A(nn+1)mgRB(nn−1)mgRCnmgRDmgRn
Find the change in the gravitational potential energy when a body of mass m is raised to a height nR above the surface of the earth. (Here, R is the radius of the earth)
View Solution" The potential energy of a body of mass "m" on the surface of earth is "
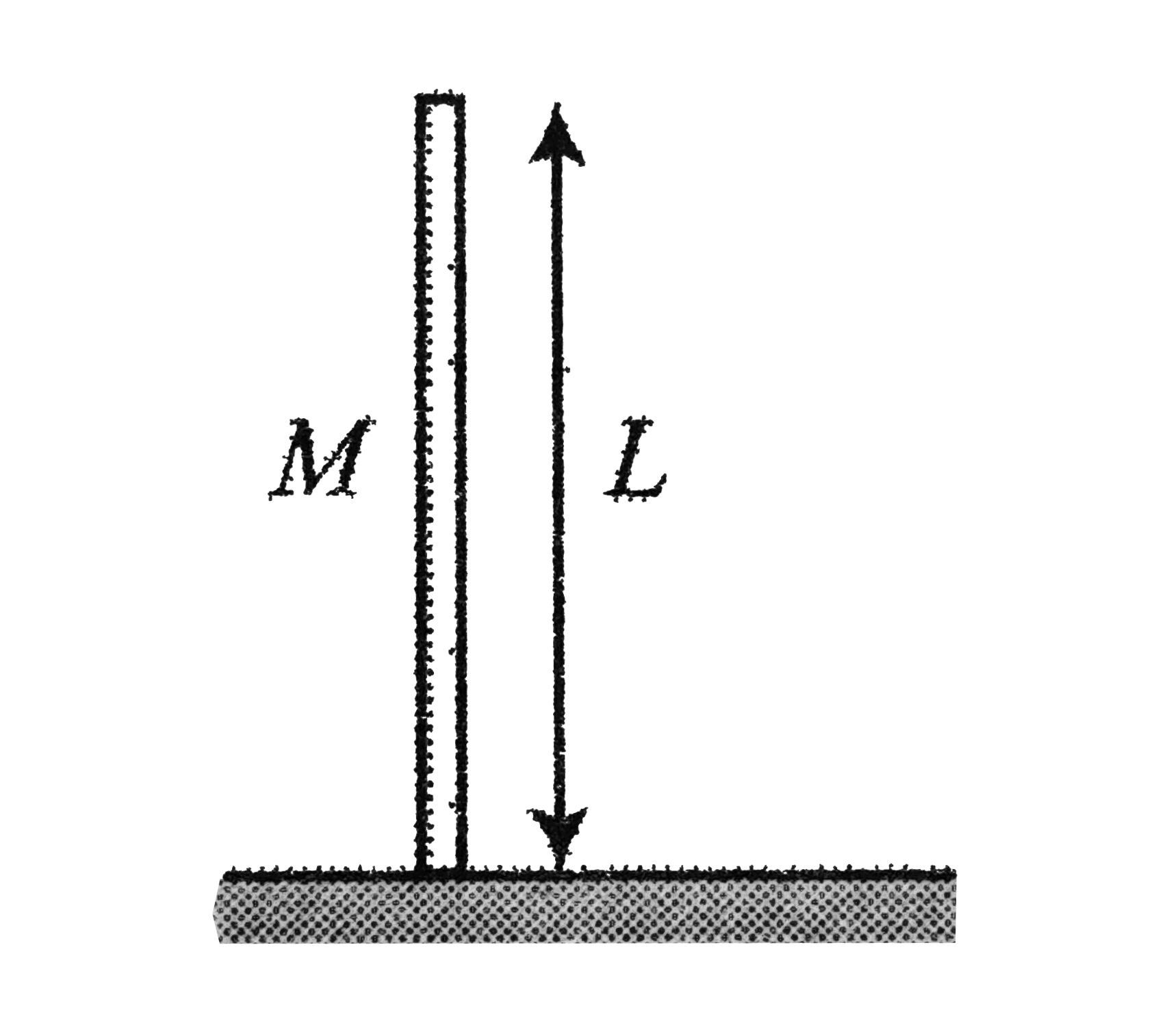
View SolutionA uniform rod of mass M and length L is held vertically upright on a horizontal surface as shown in figure. Assuming zero potential energy at the base of the rod, determine the potential energy of the rod.
 View Solution
View SolutionThe change in the gravitational potential energy when a body of a mass m is raised to a height nR above the surface of the earth is (here R is the radius of the earth)
View SolutionA particle of mass m starts to slide down from the top of the fixed smooth sphere. What is the tangential acceleration when it break off the sphere ?
View Solution

