A rocket is moving vertically upward against gravity. Its mass at time t is m=m0−μt and it expels burnt fuel at a speed u vertically downward relative to the rocket. Derive the equation of motion of the rocket but do not solve it. Here, μ is constant.
The correct Answer is:B, D
To derive the equation of motion for the rocket moving vertically upward against gravity, we will follow these steps:
Step 1: Identify the forces acting on the rocket
The forces acting on the rocket are:
1. The thrust force Ft due to the expulsion of burnt fuel.
2. The weight of the rocket W=mg, where g is the acceleration due to gravity.
Step 2: Write the expression for the mass of the rocket
The mass of the rocket at time t is given by:
m(t)=m0−νt
where m0 is the initial mass of the rocket and ν is the rate at which the mass decreases (burnt fuel).
Step 3: Apply Newton's second law
According to Newton's second law, the net force F acting on the rocket is equal to the mass of the rocket multiplied by its acceleration a:
F=m(t)a
The net force can also be expressed as the thrust force minus the weight:
F=Ft−mg
Step 4: Substitute the expressions into Newton's second law
Substituting the expressions for mass and forces into Newton's second law gives:
Ft−mg=m(t)a
Substituting m(t)=m0−νt and mg=(m0−νt)g:
Ft−(m0−νt)g=(m0−νt)a
Step 5: Express acceleration in terms of velocity
The acceleration a can be expressed as the time derivative of velocity v:
a=dvdt
Thus, we can rewrite the equation as:
Ft−(m0−νt)g=(m0−νt)dvdt
Step 6: Rearranging the equation
Rearranging the equation gives:
(m0−νt)dvdt=Ft−(m0−νt)g
Step 7: Final form of the equation of motion
The final form of the equation of motion for the rocket can be expressed as:
(m0−νt)dvdt=Ft−(m0−νt)g
Summary
The derived equation of motion for the rocket is:
(m0−νt)dvdt=Ft−(m0−νt)g
The forces acting on the rocket are:
1. The thrust force Ft due to the expulsion of burnt fuel.
2. The weight of the rocket W=mg, where g is the acceleration due to gravity.
The mass of the rocket at time t is given by:
m(t)=m0−νt
where m0 is the initial mass of the rocket and ν is the rate at which the mass decreases (burnt fuel).
According to Newton's second law, the net force F acting on the rocket is equal to the mass of the rocket multiplied by its acceleration a:
F=m(t)a
The net force can also be expressed as the thrust force minus the weight:
F=Ft−mg
Substituting the expressions for mass and forces into Newton's second law gives:
Ft−mg=m(t)a
Substituting m(t)=m0−νt and mg=(m0−νt)g:
Ft−(m0−νt)g=(m0−νt)a
The acceleration a can be expressed as the time derivative of velocity v:
a=dvdt
Thus, we can rewrite the equation as:
Ft−(m0−νt)g=(m0−νt)dvdt
Rearranging the equation gives:
(m0−νt)dvdt=Ft−(m0−νt)g
The final form of the equation of motion for the rocket can be expressed as:
(m0−νt)dvdt=Ft−(m0−νt)g
The derived equation of motion for the rocket is:
(m0−νt)dvdt=Ft−(m0−νt)g
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Exercise 11.5
4 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Exercise 11.6
11 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Exercise 11.3
7 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery
19 Videos
Similar Questions
A rocket with an initial mass of 1000kg, is launched vertically upward from rest under gravity. The rocket burns fuel at the rate of 10kg per second. The burnt matter is ejected vertically downwards with a speed of 2000m/s relative to the rocket . Find the velocity of the rocket after 1min of start.
View SolutionA rocket of initial mass m0 has a mass m0(1−t/3) at time t. The rocket is lauched from rest vertically upwards under gravity and expels burnt fuel at a speed u relative to the rocket vertically downward. Find the speed of rocket at t=1.
View Solution
Knowledge Check
A rocket, with an initial mass of 1000kg, is launched vertically upwards from rest under gravity. The rocket burns fuel at the rate of 10kg per second. The burnt matter is ejected vertically downwards with a speed of 2000ms−1 relative to the rocket. If burning ceases after one minute, find the maximum velocity of the rocket. (Take g as constant at 10ms−2)
A1232.6m//sB123.6m//sC12323.6m//sDNONE OF THE ABOVEA rocket of mass 1000 kg is to be projected vertically upwards. The gases are exhausted vertically downwards with velocity 100m/s with respect to the rocket. (What is the minimum rate of burning fuel, so as to just lift the rocket upwards against the gravitational attraction?) (Take g=10m/s2)
A50 kg/sB100kg/sC200kg/sD400kg/sA rocket of mass 5000 kg is to be projected vertically upward. The gases are exhausted vertically downwards with velocity 1000ms−1 with respect to the rocket. What is the minimum rate of burning the fuel so as to just lift the rocket upwards against gravitational attraction ?
A49kgs−1B147kgs−1C98kgs−1D196kgs−1
A rocket of initial mass m ( including fuel) ejects mass at a constant rate of 25 kg/s with a speed 60 m/s relative to the rocket. If the acceleration of the rocket 3.5 minutes after the firing is 2m/s2. What is the initial mass of the rocket ? [Neglect gravity] Express your answer in unit of 103kg
View Solutiona rocket of mass 1000 kg is to be projected vertically upwards the gases are exhausted vertically downwards with velocity 100ms−1 with respect to the rocket what is the min rate of burning of fuel so as to just lift the rocket upwards against the gravitational attraction?(Take g=10ms−2)
View Solutiona rocket of mass 1000 kg is to be projected vertically upwards the gases are exhausted vertically downwards with velocity 100ms−1 with respect to the rocket what is the min rate of burning of fuel so as to just lift the rocket upwards against the gravitational attraction?(Take g=10ms−2)
View Solution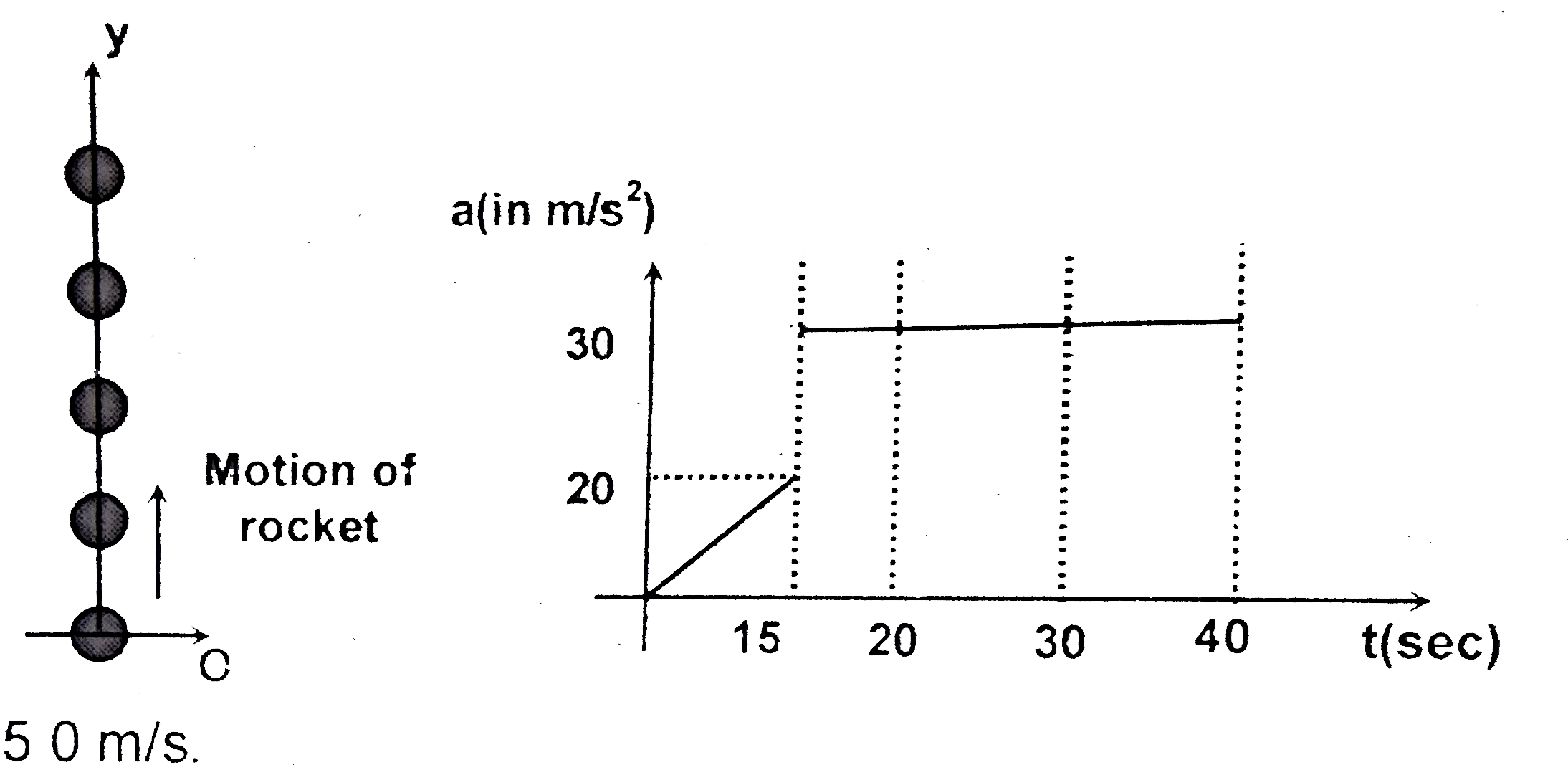
A two stage rocket is fired vertically upward from rest with acceleration as shown in a-t graphs. After 15 sec, the first stage burns out and second stage ignites. Choose the INCORRECT statement regarding motion of rocket in the time interval 0≤t≤40s.
View Solution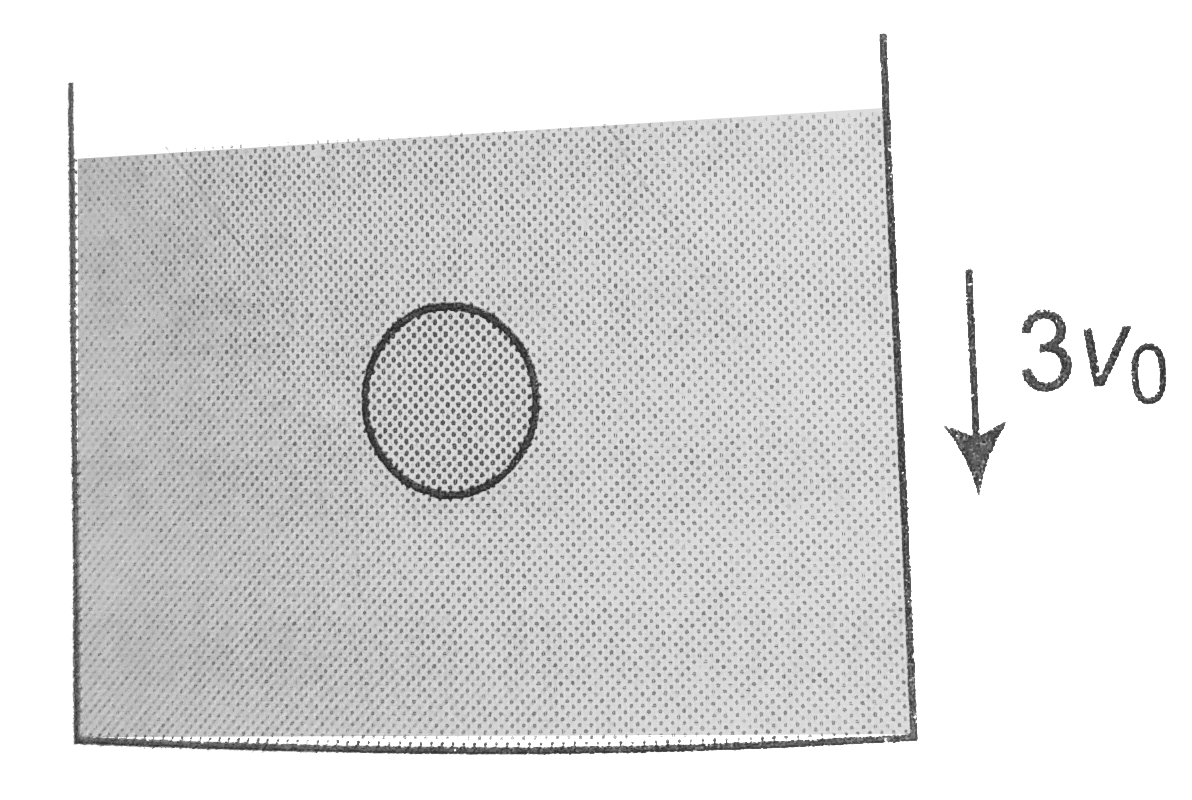
A container filled with viscous liquid is moving vertically downwards with constant speed 3v0. At the instant shown, a sphere of radius r is moving vertically downwards (in liquid) has speed v0. The coefficient of viscosity is η. There is no relative motion between the liquid and the container. Then at the shoen instant, The magnitude of viscous force acting on sphere is
View Solution
