Assertion: A ring is rolling without slipping on a rough ground. It strikes elastically with a smooth wall as shown in figure. Ring will stop after some time while travelling in opposite direction.
Reason: After impact net angular momentum about an axis passing through bottommost point and perpendicular to plane of paper is zero.
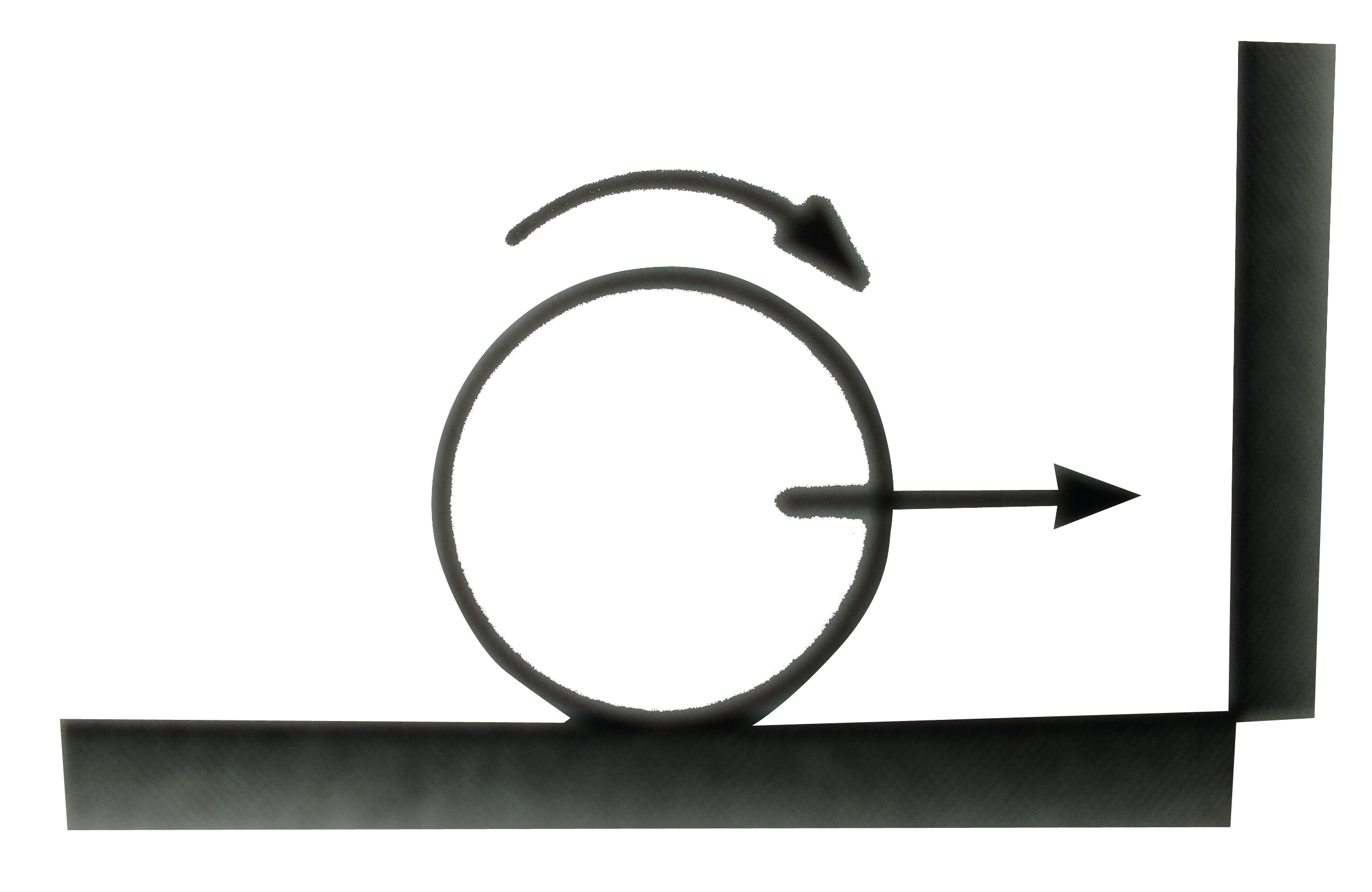
If both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
If assertion is true, but the reaction is false.
If assertion is false but the reason is true.
In second figure. Net angular momentum about bottommost axis
L=mvR−ICω
=mvR−(mr2)(vR)
=0
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 1 Objective
30 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 1 Subjective
32 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercise 12.11
2 Videos
- ROTATIONBOOK - DC PANDEYCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEYCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
Similar Questions
A sphere rolling on a horizontal rough surface collides elastically with a smooth vertical wall, as shown in figure. During collision, angular momentum of the sphere is conserved about ____________. (Any point O on the surface/point of contact P)

View SolutionA sphere rolling on a horizontal rough surface Collides elastically with a smooth vertical wall, as shown in Fig. State which of the following statements is true or false.
 a. After collision, the velocity of the centre of mass gets reversed.
a. After collision, the velocity of the centre of mass gets reversed.
b. Angular momentum of the sphere about the point of contact with the wall is conserved.
c. Angular momentum of the sphere about a stationary point on the horizontal surface is conserved.
d. Just after collision the point of contact with the horizontal surface is moving towards the wall.
e. After collision the friction force acts on the sphere such that it decreases the linear speed and increases the angular speed.
f. Finally, when the sphere starts rolling, it is moving away from the wall.
View Solution
Knowledge Check
A disc of mass m and radius R is rolling on horizontal ground with linear velocity v. What is the angular momentum of the disc about an axis passing through bottommost point and perpendicular to the plane of motion ?
A32mvRBmvRC12mvRD43mvRAssertion: A ball is released on a rough ground in the condition shown in figure. it will start pure rolling after some time towards left side.
Reason: Friction will convert the pure rotational motion of the ball into pure rolling AIf both Assertion and Reason are true and the Reason is correct explanation of the Assertion.BIf both Assertion and Reason are true but Reason is not the correct explanation of Assertion.CIf assertion is true, but the reaction is false.DIf assertion is false but the reason is true.
AIf both Assertion and Reason are true and the Reason is correct explanation of the Assertion.BIf both Assertion and Reason are true but Reason is not the correct explanation of Assertion.CIf assertion is true, but the reaction is false.DIf assertion is false but the reason is true.A uniform and constant magnetic field B is directed perpendicularly into the plane of the page everywhere within a rectangular region as shown in figure-5.277. A wire circuit in the shape of a semicircle is rotated at uniform angular speed in counter clockwise direction in the plane of the page about an axis passing through point A. The axis A is perpendicular to straight line portion of the circuit. Which of the following graphs best approximates the EMF E induced in the the page at the edge of the field and directed through the centre of the circuit as a function of time l ?
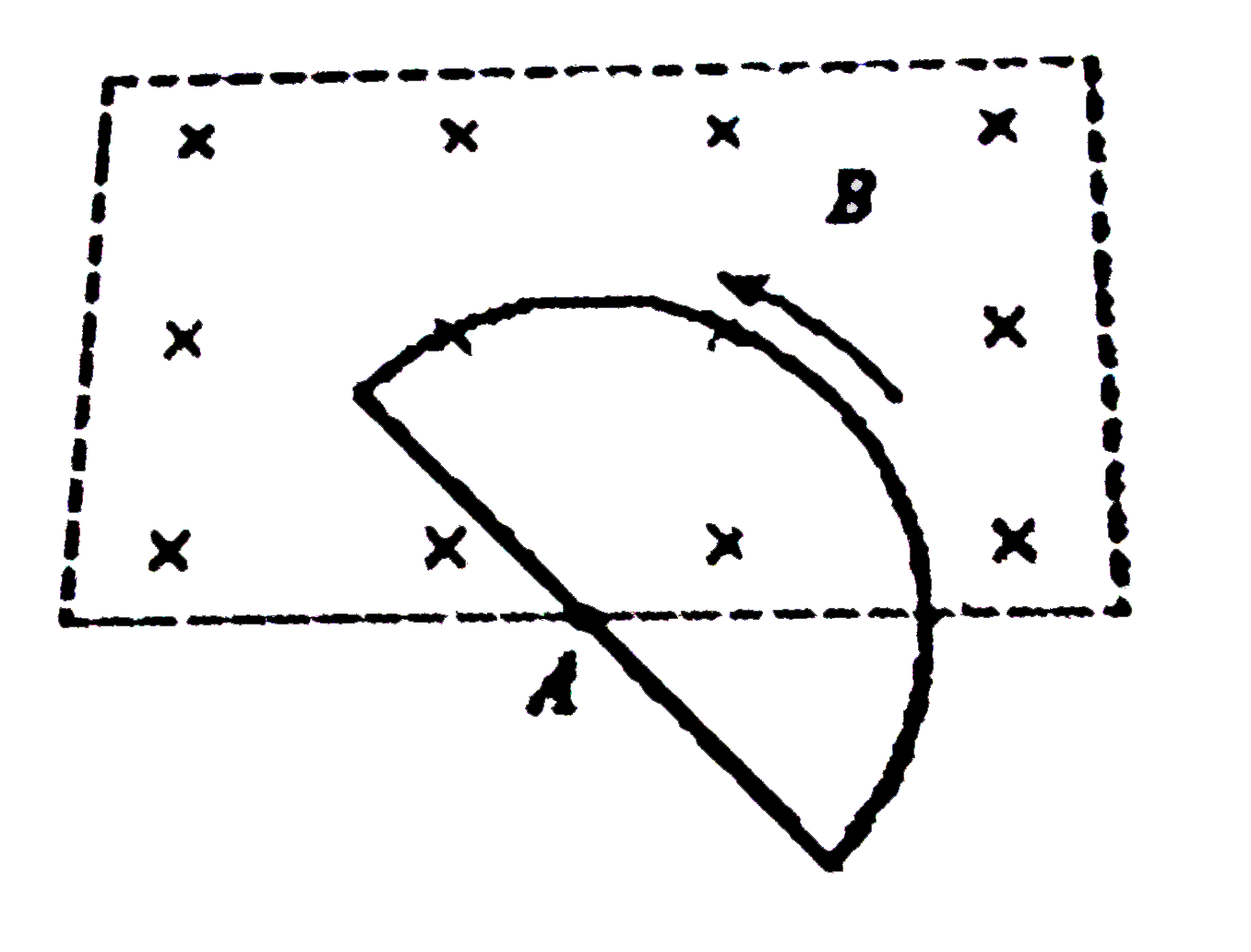 A
A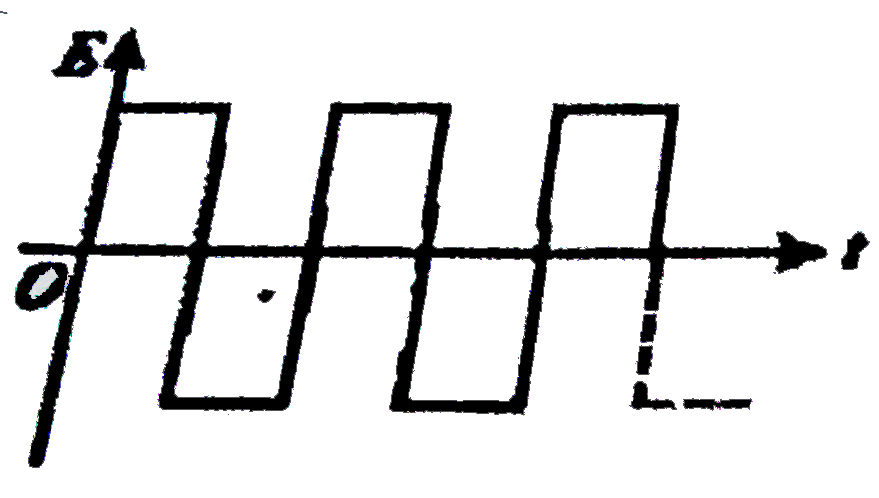 B
B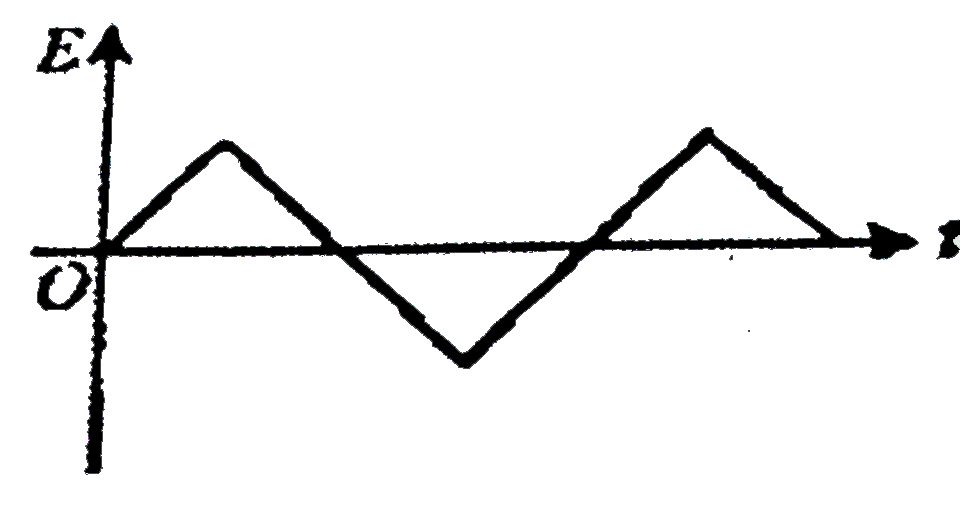 C
C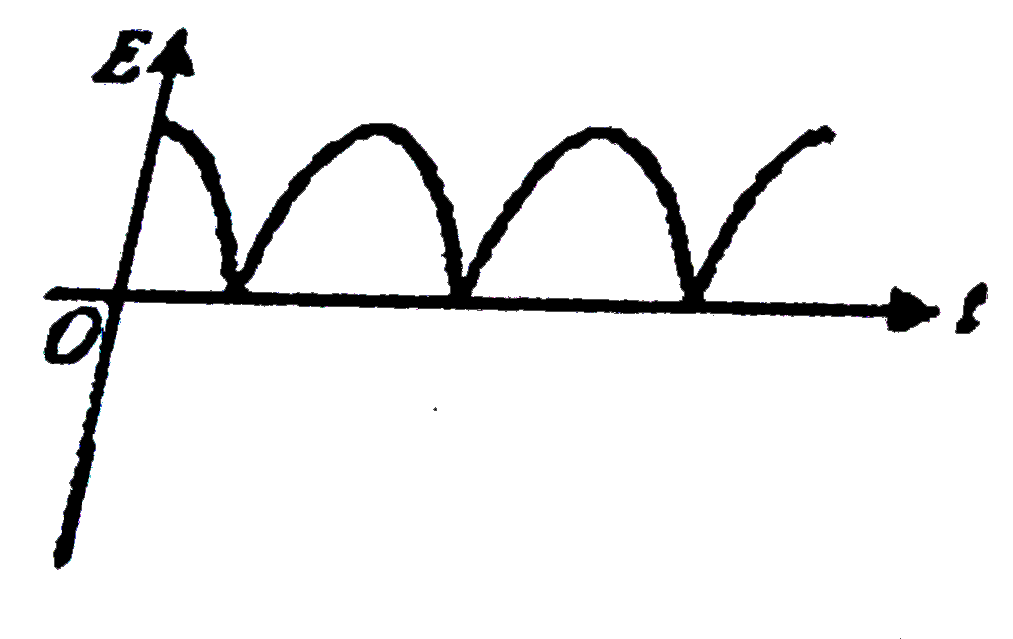 D
D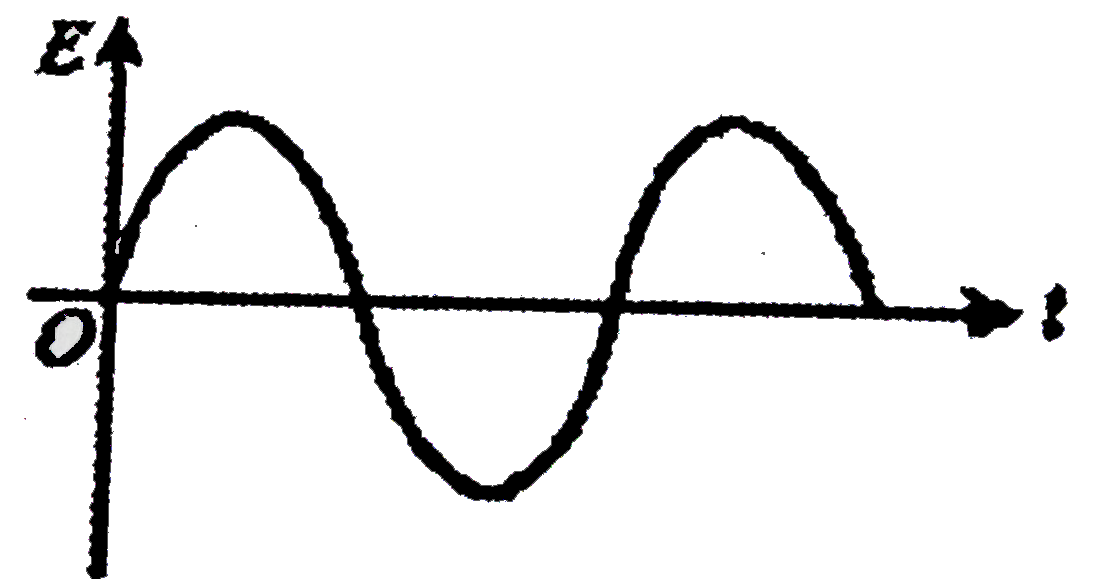
A solid sphere is rolling without slipping on rough ground as shown in figure. It collides elastically with another identical sphere at rest. There is no friction between the two spheres. Radius of each sphere is R and mass is m.

What is the net angular impulse about centre of mass imparted to second sphere by the external forces?
View SolutionA disc shaped body (having a hole shown in the figure) of mass m=10kg and radius R=109m is performing pure rolling motion on a rough horizontal surface. In the figure point O is geometrical center of the disc and at this instant the centre of mass C of the disc is at same horizontal level with O. The radius of gyration of the disc about an axis pasing through C and perpendicular to the plane of the disc is R2 and at the insant shown the angular velocity of the disc is ω=√gR rad/sec in clockwise sence. g is gravitation acceleration =10m/s2. Find angular acceleation α ( in rad/s2) of the disc at this instant.

View SolutionA non-conducting ring of radius R having uniformly distributed charge Q starts rotating about x-x' axis passing through diameter with an angular acceleration α, as shown in the figure. Another small conducting ring having radius a(a<<R) is kept fixed at the centre of bigger ring is such a way that axis xx' is passing through its centre and perpendicular to its plane. If the resistance of small ring is r=1Ω , find the induced current in it in ampere.
(Given q=16×102μ0C,R=1m,a=0.1m,α=8rad s−2
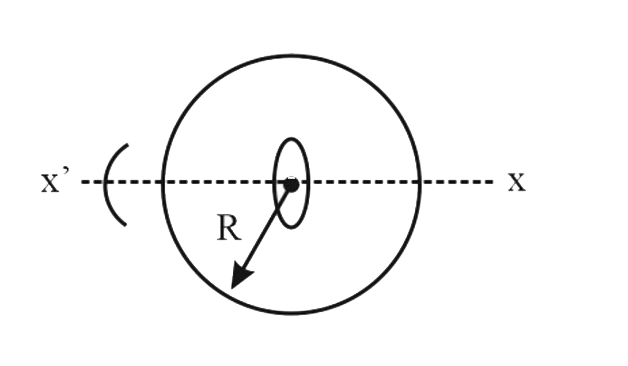
View SolutionA non-conducting ring of radius R having uniformly distributed charge Q starts rotating about x-x' axis passing through diameter with an angular acceleration α, as shown in the figure. Another small conducting ring having radius a(a<<R) is kept fixed at the centre of bigger ring is such a way that axis xx' is passing through its centre and perpendicular to its plane. If the resistance of small ring is r=1Ω , find the induced current in it in ampere.
(Given q=16×102μ0C,R=1m,a=0.1m,α=8rad s−2

View SolutionIn figure, there is conducting ring having resistance R placed in the plane of paper in a uniform magnetic field B0. If the rings is rotating in the plane of paper about an axis passing through point O and perpendicular to the plane of paper with constant angular speed ω in clockwise direction, then
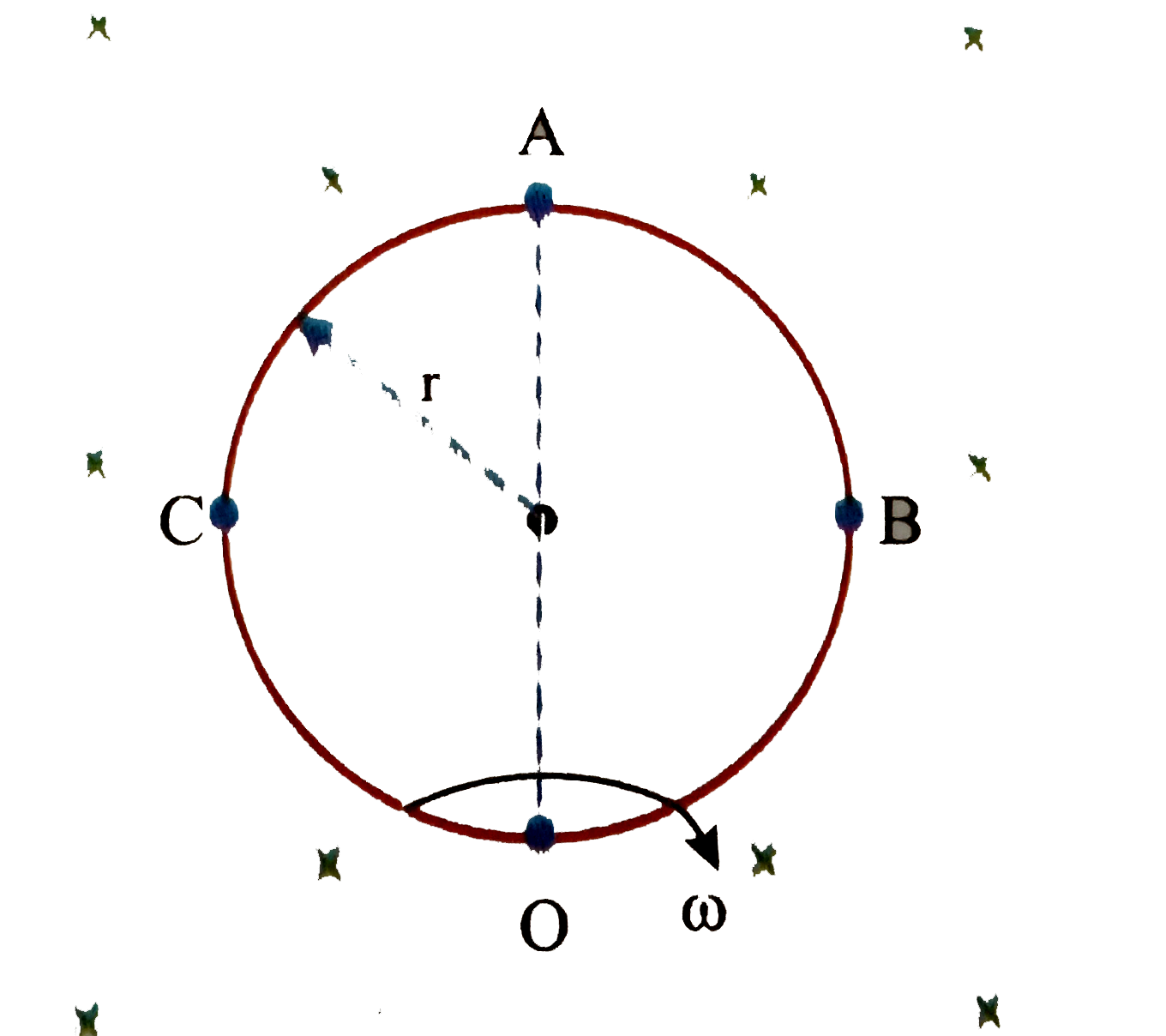
View Solution
