Show that the combined spring energy and gravitational energy for a mass m hanging from a light spring of force constant k can be expressed as U0+12ky2, where y is the distance above or below the equilibrium position and U0 is constant.
The correct Answer is:A
At equilibrium position, kx0=mg

In displaced position, U=12k(x0+y)2−mgy
=12kx20+12ky2+kx0y−mgy
Substituting kx0=mg we get,
U=12kx20+12ky2
=12kx20+12ky2+kx0y−mgy
But 12kx20 = constant say U0
∴U=U0+12ky2.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 Single Correct
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 More Than One Correct
8 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 1 Single Correct
24 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEYCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEYCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
Define elastic potential energy. An elastic spring of force constant k is compressed by an amount x. Show that its pulenlial energy is 12kx2
View SolutionA block of mass m hangs from a vertical spring of spring constant k. If it is displaced from its equilibrium position, find the time period of oscillations.
View Solution
Knowledge Check
A cage of mass M hangs from a light spring of force constant k. A body of mass m falls from height h inside the cage and stricks to its floor. The amplitude of oscillations of the cage will be-
_E01_061_Q01.png) A(2mghk)1/2B(k2mgh)1/2CmgkD(mgk)1/2
A(2mghk)1/2B(k2mgh)1/2CmgkD(mgk)1/2A particle of mass m is hanging vertically by an ideal spring of force constant K . If the mass is made to oscillate vertically, its total energy is
AMaximum at extreme positionBMaximum at mean positionCMinimum at mean positionDSame at all positionThe period of oscillation of a mass M, hanging from a spring of force constant k is T. When additional mass m is attached to the spring, the period of oscillation becomes 5T/4. m/M =
A9:16B25:16C25:9D16:9
The frequency f of vibrations of a mass m suspended from a spring of spring constant k is given by f=Cmxky , where C is a dimensionnless constant. The values of xandy are, respectively,
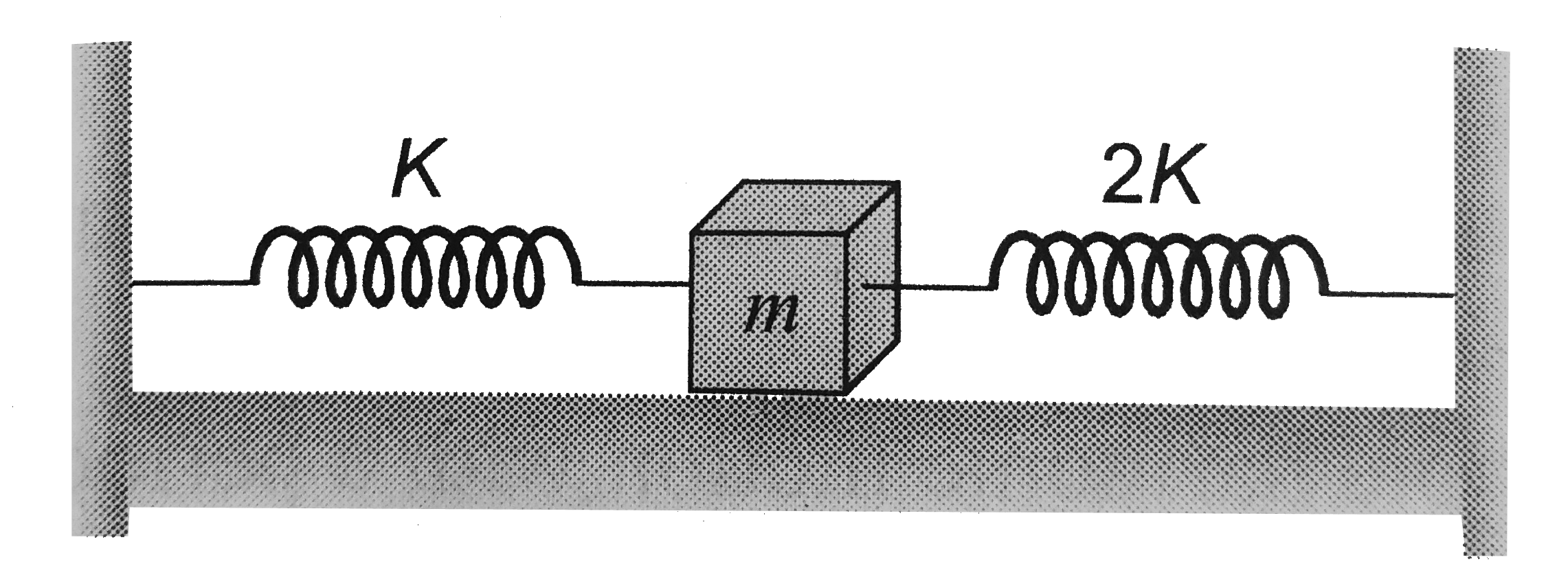
View SolutionTwo spring of force constants K and 2K are connected a mass m below The frequency of oscillation the mass is
View SolutionGiven M is the mass suspended from a spring of force constant. k.The dimensional formula for [M/k]1/2 is same as that for
View SolutionAs shown in the figure, two light springs of force constant k1 and k2 oscillate a block of mass m. Its effective force constant will be
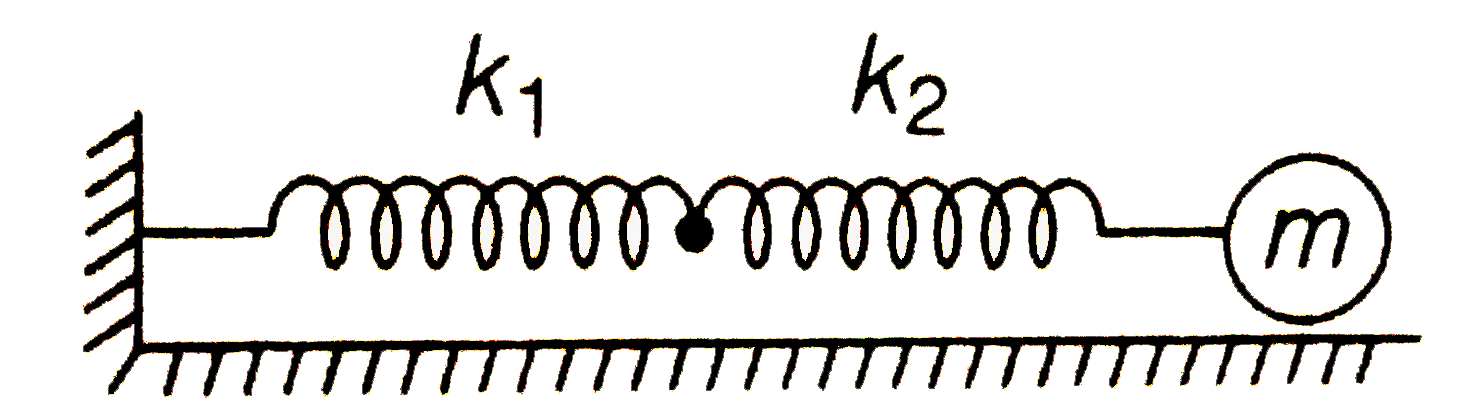
View SolutionA block of mass 4 kg hangs from a spring constant k=400Nm. The block is pulled down through 15 cm below and released. What is its kinetic energy when the block is 10 above the equilibrium position.?
View Solution
