An aluminium wire of cross-sectional area is joined to a steel wire of the same cross-sectional area. This compound wire is stretched on a sonometer pulled by a weight of 10kg. The total length of the compound wire between the bridges is 1.5m of which the aluminium wire is and the rest is steel wire. Transverse vibrations are setup in the wire by using an external source of variable frequency. Find the lowest frequency of excitation for which the standing waves are formed such that the joint in the wire is a node. What is the total number of nodes at this frequency? The density of aluminium is and that of steel is
The correct Answer is:A, B, C, D
Let loops are formed in aluminium wire and in steel. Then,
or
or
But,
Therefore,
Substituting the values, we have
i.e. at lowest frequency, one loop is formed in aluminium wire and three loops are formed in steel wire as shown in figure.
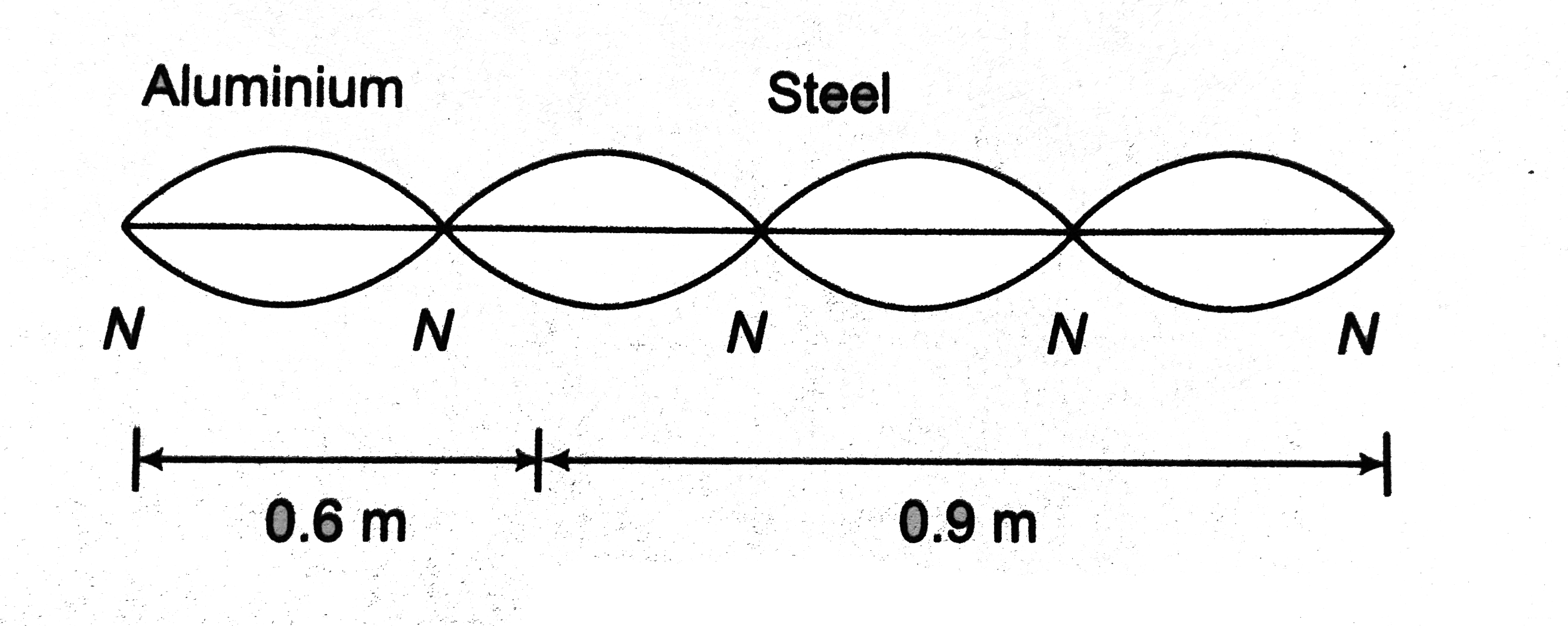
or Hz.
Total number of nodes are five as shown in figure.
Topper's Solved these Questions
- SUPERPOSITION OF WAVESBOOK - DC PANDEYCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Exercise 18.1
3 Videos
- SUPERPOSITION OF WAVESBOOK - DC PANDEYCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Exercise 18.2
4 Videos
- SUPERPOSITION OF WAVESBOOK - DC PANDEYCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Example Type 4
1 Videos
- SOUND WAVESBOOK - DC PANDEYCHAPTER - SOUND WAVESEXERCISE - Exercise 19.7
4 Videos
- THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESBOOK - DC PANDEYCHAPTER - THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESEXERCISE - Medical entrance gallary
30 Videos
Similar Questions
An aluminium wire of cross-sectionbal area 1×10−6m2 is joined to a steel wire of same cross-sectional ara. This compound wire is stretched on a sonometer, pulled by a weight of 10kg. The total length of the compound wire between the bridges is 1.5m, of which the aluminium is 0.6m and the rest is steel wire. Transverse vibrationas are set up in the wire by using an ecternal force of variable frequency. Find the lowest frequency of excitation for which standing waves are formed such that the joint in the wire is a node. What is the total number of nodes observed at this frequency, excluding the two at the ends of the wire? The density of aluminium is 2.6×103kg/m3 and that of steel is 1.04×104kg/m3.
View SolutionAn aluminium wire of cross-sectionbal area 1×10−6m2 is joined to a steel wire of same cross-sectional ara. This compound wire is stretched on a sonometer, pulled by a weight of 10kg. The total length of the compound wire between the bridges is 1.5m, of which the aluminium is 0.6m and the rest is steel wire. Transverse vibrationas are set up in the wire by using an ecternal force of variable frequency. Find the lowest frequency of excitation for which standing waves are formed such that the joint in the wire is a node. What is the total number of nodes observed at this frequency, excluding the two at the ends of the wire? The density of aluminium is 2.6×103kg/m3 and that of steel is 1.04×104kg/m3.
View Solution
Knowledge Check
An alumminium rod and steel wire of same length and cross-section are attached end to end. Then compound wire is hung fron a rigid support and load is suspended from the free end. Y for steel is (207) times of aluminium. The ratio of increase in length of steel wire to the aluminium wire is
A20:3B10:7C7:20D1:7
An aluminimum wire of cross - sectional area 1×10−6m2 is joined to a steel wire of the same cross - sectional area . This compound wire is stretched on a sonometer , pulled by a weight of 10kg. The total length of the compound wire between the bridges is 1.5m of which the aluminium wire is 0.6m and the rest is steel wire . Transverse vibrations are set up in the wire by using an external source of variable frequency . Find the lowest frequency of excitation for which standing waves are formed , such that the joint in the wire is a node . What is the total number of nodes observed at this frequency , excluding the two at the ends of the wire ? The density of aluminium is 2.6×103kg/m3 and that of steel is 1.04×104kg/m3
View SolutionAn aluminium wire of length 0.6 m and cross-sectional area 10−6m2 is connected to a steel wire of the same crosssectional area and length 0.866 m. The compound wire is loaded with 10 kg. Find the lowest frequency of excitation for which the joint in the wire is a node. Also find the number ofnodes, excluding the two at the ends of the wire. The density of aluminium is 2600kg/m3 andthatofsteel is 7800kg/m3
View SolutionA steel wire having cross-sectional area 2 mm^(2) is stretched by 20 N. Find the lateral strain produced in the wire Y_("steel") = 2 xx 10^(11) Nm^(-2), sigma = 0.391
View SolutionA wire whose cross-sectional area is 2mm2 is stretched by 0.1 mm by a certain load, and if a similar wire of triple the area of cross-section is stretched by the same load, then the elongation of the second wire would be
View SolutionA wire of cross-sectional area A breaks due to its own weight when length of the wire is l. If area of cross-section of the wire is made 3A then maximum length of the wire can be hung without breaking is
View SolutionA wire of cross-sectional area A breaks due to its own weight when length of the wire is l. If area of cross-section of the wire is made 3A then maximum length of the wire can be hung without breaking is
View SolutionAn aluminium wire of length 0.6 m and cross sectioal are and length 0.866 m .The compound wire is loaded with 10kg.Find the lowest frequency of exciation for which the joint In the the wire.The density of alumimium is 2600 kg m−3 and that of steel is 7800 kgm−3
View Solution
