Call the (rms) speed of the molecules in an ideal gas V0 at temperature T0 and pressure p0. Find the speed if (a) the temperature id raised from T0=293Kto573K (b) the pressure is doubled and T=T0 ( c) the molecular weight of each of the gas molecules is tripled.
The correct Answer is:A, B, C, D
(a) vrms∝√T
∴V0=(√T'T)v0=(√573293)v0
= 1.40v0
(b) vrms does not depend on pressure, as long as temperature remains constant.
(c ) vrms=√3RTMorvrms∝1√(M).
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESEXERCISE - Level 2 Single Correct12videos
THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASES
- BOOK - DC PANDEYCHAPTER - THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESEXERCISE - Level 2 More Than One Correct7videos
THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASES
- BOOK - DC PANDEYCHAPTER - THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESEXERCISE - Level 1 Objective10videos
THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASES
- BOOK - DC PANDEYCHAPTER - THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESEXERCISE - Medical entrance gallary30videos
THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASES
- BOOK - DC PANDEYCHAPTER - UNIT AND DIMENSIONSEXERCISE - Assertion And Reason2videos
UNIT AND DIMENSIONS
Similar Questions
The most probable speed of the molecules in a gas at temperature T2 is equal to the average speed of the molecules at temperature T1 Find T2/T1
View SolutionThe root mean square speed of the molecule at constant pressure at temperature T is v, what is its root mean square speed, if temperature is reduced to T2.
View Solution
Knowledge Check
- Question 1 - Select One
The translational K.E. of the molecules of a gas at absolute temperature (T) can be doubled
Aby increasing T to 4TBby increasing T to √2TCby decreasing T to T2Dby increasing T to 2T - Question 2 - Select One
The root mean square speed of gas molecules at a temperature 27K and pressure 1.5 bar is 1×104cm/sec If both temperature and pressure are raised three times calculate the new rms speed of gas molecules .
A9×104cm/s2B3×104cm/sC√3×104cm/sD1×104cm/s - Question 3 - Select One
One mole of an ideal monoatomic gas at temperature T0 expands slowely according to the law P/V=constant. If the final temperature is 2T0 heat supplied to the gas is -
A2RT0B32RT0CRT0D12RT0
The r.m.s speed of the molecules of an ideal gas Is V0 If pressure of the gas is halved but temperature is kept constant then r.m.s. speed of the gas molecule will be
View SolutionThe root mean square speed of the molecule at constant pressure at temperature T is v, what is its rms speed, if temperature is reduced to T2?
View SolutionA quantity of gas occupies an initial volume V0 at pressure p0 and temperature T0. It expands to a volume V (a) constant temperature and (b) constant pressure. In which case does the gas do more work ?
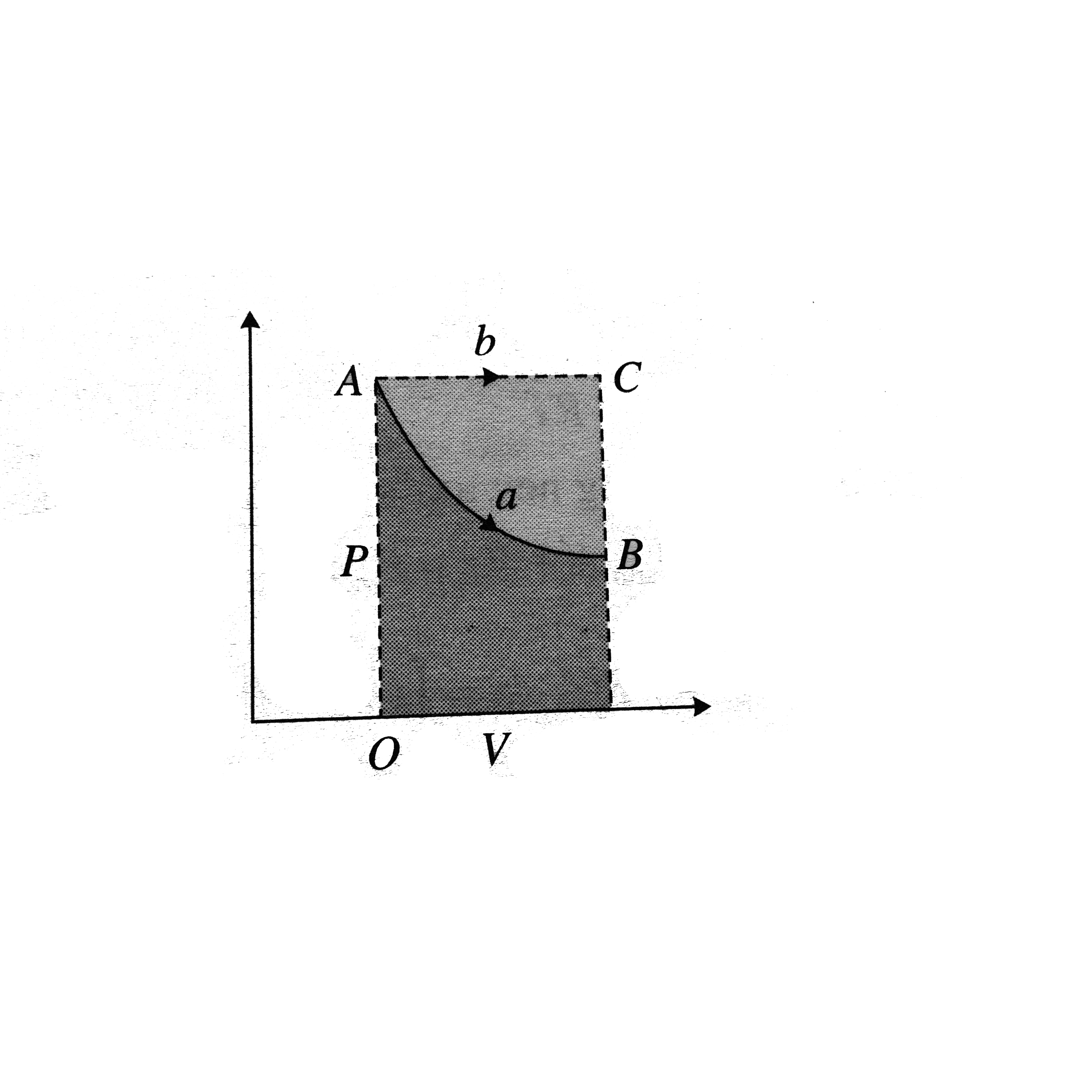 View Solution
View SolutionOne mole of an ideal monoatomic gas at temperature T0 expands slowly according to the law pV = constant. If the final temperature is 2T0, heat supplied to the gas is
View SolutionThe heat supplied to one mole of an ideal monoatomic gas in increasing temperature from T0 to 2T0 is 2RT0. Find the process to which the gas follows
View Solution

