Three charges q1=1μC,q2=2μC and q3=3μC are placed on the vertices of an equilateral triangle of side 1.0 m. Find the net electric force acting on charge q1
The correct Answer is:A, B, C, D
Method I. In the figure
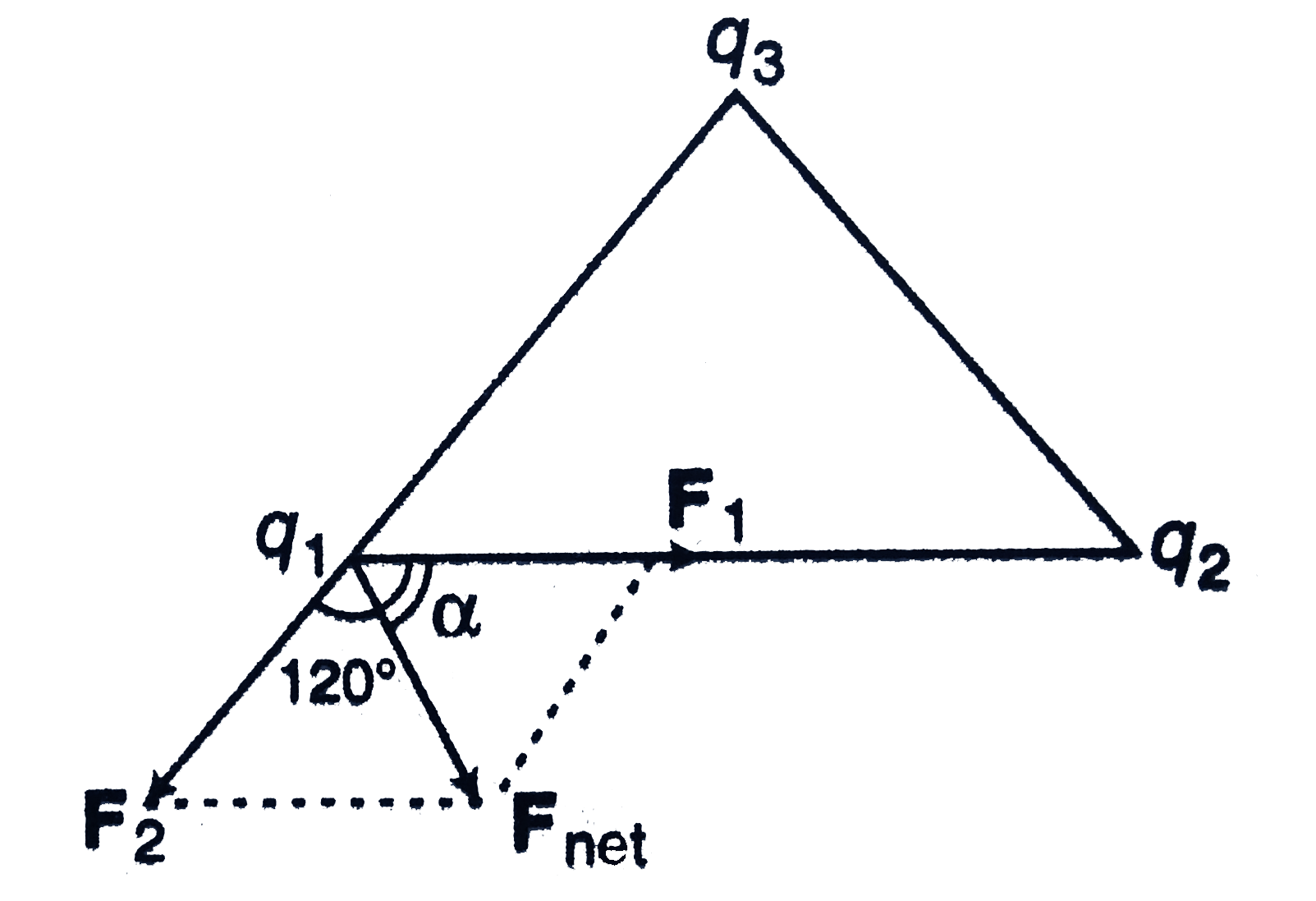 <br>|F_(1)|=F_(1)=1/(4piepsilon_0).(q_1q_2)/r^2<br>=magnitudeofforcebetweenq_1 and q_2<br>((9.0xx10^9)(1.0xx10^-6)(2.0xx10^-6))/((1.0)^2)<br>=1.8xx10^-2N<br>Similarly |F2|=F2=14πε0.q1q3r2
<br>|F_(1)|=F_(1)=1/(4piepsilon_0).(q_1q_2)/r^2<br>=magnitudeofforcebetweenq_1 and q_2<br>((9.0xx10^9)(1.0xx10^-6)(2.0xx10^-6))/((1.0)^2)<br>=1.8xx10^-2N<br>Similarly |F2|=F2=14πε0.q1q3r2
=magnitudeofforcebetweenq_1andq_3<br>=((9.0xx10^9)(1.0xx10^-6)(3.0xx10^-6))/((1.0)^2)<br>=2.7xx10^-2N<br>Now,|F_"net")|=sqrt(F_1^2+F_2^2+2F_1F_2cos120^@)<br>=(sqrt((1.8)^2+(2.7)^2=2(1.8)(2.7)(-1/2)))xx10^-2N<br>=2.38xx10^-2N<br>andtan alpha=(F_2sin120^@)/(F_1+F_2cos120^@)<br>=((2.7xx10^-2)(0.87))/((1.8xx10^-2)+(2.7xx10^-2)(-1/2))<br>oralpha=79.2^@<br>Thus,the≠tforceonchar≥q_1is2.38xx10^-2Natan∠alpha=79.2^@withal∈ejo∈∈gq_1andq_2asshown∈thefigure.<br>Method2.Inthisfiguremethod≤tusas∑eac∞rd∈ateaxeswithq_1atorig∈asshown∈figure.Thec∞rd∈ateofq_1,q_2andq_3∈thisc∞rd∈atesystemare(0,0,0), (1m,0,0)and(0.5m, 0.87m,0)respectively.Now<br><imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/DCP_VOL_4_C24_S01_008_S02.pngwh=80%mx-autosty≤=background:white;>
F1=force of q1 due to charge q2
=14πε0.q1q2|r1−r2|3(r1−r2)
=(9.0×109)(1.0×10−6)(−2.0×10−6)(1.0)3[(0.1)ˆi+(0−0)ˆj+(0−0)ˆk]
=(1.8×10−2ˆi)N
and F2=Force on q1 due to charge q3
=14πε0.q1q3|r1−r3|3(r1−r3)
=(9.0×109)(1.0×10−6)(3.0×10−6)(1.0)3[(0−0.5)ˆi+(0−0−0.87)ˆj+(0−0)ˆk]
=(−1.35ˆi−2.349ˆj)×10−2N
Therefore net force on q1 is F=F1+F2
=(0.45ˆi−2.349ˆj)×10−2N
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Example Type 55videos
ELECTROSTATICS
- BOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Example Type 64videos
ELECTROSTATICS
- BOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises50videos
ELECTROSTATIC POTENTIAL AND CAPACITORS
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions120videos
GRAVITATION
Similar Questions
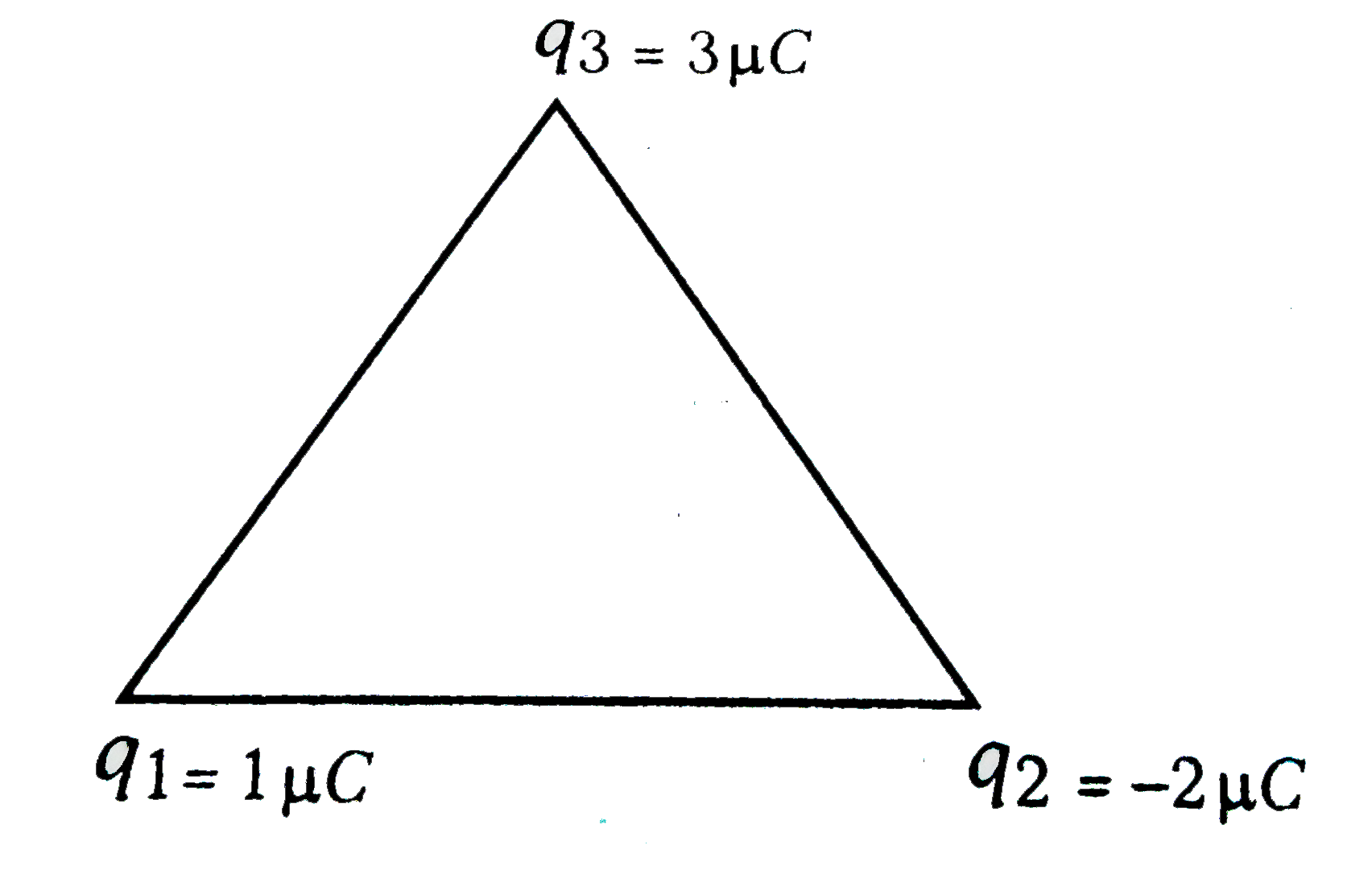
Three charges q1=1μC,q2=−2μC and q3=3μC are placed on the vertices of an equilateral triangle of side 1.0 m. find the net electric force acting on charge q1.
How to proceed Charge q2 will attract charge q1 (along the line joining them) and charge q3 will repel charge q1. Therefore, two forces will act on q1, one due to q2 and another due to q3. Since , the force is a vector quantity both of these force (say F1 and F2) will be added by vector method. Following are two methods of their addition
 View Solution
View SolutionThree point charges 2μC,3μCand5μC are placed at the corners of an equilateral triangle of side 200 cm. Calculate the potentail energy of the system.
View Solution
Knowledge Check
- Question 1 - Select One
Three charges 4μC,4μCand−4μC are placed at vertices of an equilateral triangle of side length 20cm. The potential centre is nearly.
A3.1×103VB3.1×104VC3.1×105VD3.1×106V - Question 2 - Select One
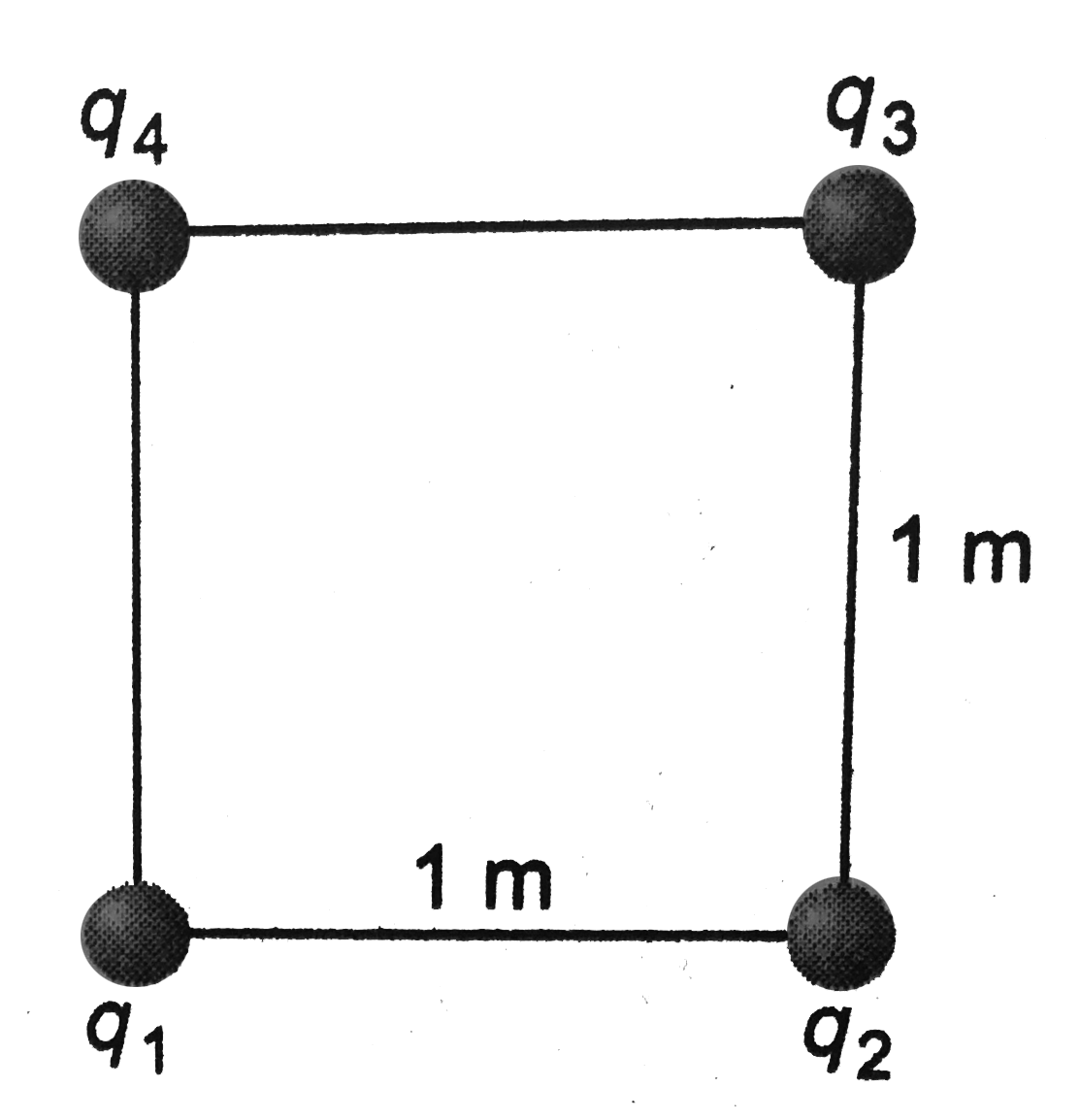
Four charges q1=1μC,q2=2μC,q3=−3μC and q4=4μC are kept on the vertices of a square of side 1m. Find the electric potential energy of this system of charges.
 A−5.28×10−2JB−7.62×10−2JC−8.91×10−2JD−4.33×10−2J
A−5.28×10−2JB−7.62×10−2JC−8.91×10−2JD−4.33×10−2J - Question 3 - Select One
Three point charges q are placed at three vertices of an equilateral triangle of side a. Find magnitude of electric force on any charge due to the other two.
A(√34πε0)(qa)2B(√24πε0)(qa)2C(√34πε0)(q2a)2D(√24πε0)(2qa)2
Four charges q1=1μC,q2=2μC,q3=−3μCandq4=4μC are kept on the vertices of a square of side 1m. Find the electric potential energy of this system of charges.
 View Solution
View SolutionConsider the charges q,q and -q placed at the vertices of an equilateral triangle of each side l. What is the force on each charge ?
View SolutionCharges of +2 +2, and −2μC respectively are placed at the vertices of an equilateral triangle of side 0.3m each in Find the net forces experienced by each charges.
View SolutionThree charges 2q,−q,and−q are located at the vertices of an equilateral triangle. At the center of the triangle,
View SolutionThree charges Q,(+q) and (+q) are placed at the vertices of an equilateral triangle of side l as shown in the figure. It the net electrostatic energy of the system is zero, then Q is equal to
 View Solution
View Solution

