(A) Two electric field lines cannot cross each other. Also, they cannot crossed loops. Give reasons. (B) A particle of charge 2 μC and mass 1.6 g is moving with a velocity 4ˆims−1.At t=0 the particle enters in a region having an electric field →E( in NC−1) = 80ˆi+60ˆj. Find the velocity of the particle at t=5s
Solution:
(A) To understand why two electric field lines cannot cross each other and cannot form crossed loops, let’s analyze the situation:
1. Unique Direction of Electric Field: Electric field lines represent the direction of the electric field at any point in space. If two lines were to cross, it would imply that at the point of intersection, the electric field has two different directions. This contradicts the definition of an electric field, which states that at any given point in space, the electric field can only have one unique direction.
2. Conservative Nature of Electric Fields: Electric fields are conservative in nature, meaning that the work done in moving a charge between two points is independent of the path taken. If electric field lines were to form crossed loops, it would imply that the work done in moving a charge around the loop could be zero, which is not possible in a conservative field. Therefore, electric field lines cannot cross each other or form loops.
(B) Now, let’s solve the second part of the problem regarding the charged particle.
1. Given Data:
- Charge of the particle, q=2μC=2×10−6C
- Mass of the particle, m=1.6g=1.6×10−3kg
- Initial velocity, →v0=4^im/s
- Electric field, →E=80^i+60^jN/C
2. Acceleration due to Electric Field:
The force on the particle due to the electric field is given by:
→F=q→E
Substituting the values:
→F=(2×10−6)(80^i+60^j)=(1.6×10−4^i+1.2×10−4^j)N
The acceleration →a can be calculated using Newton's second law:
→a=→Fm=(1.6×10−4^i+1.2×10−4^j)1.6×10−3=(0.1^i+0.075^j)m/s2
3. Velocity after 5 seconds:
The velocity of the particle at any time t can be calculated using the equation:
→v=→v0+→at
Substituting the values:
→v=(4^i)+(0.1^i+0.075^j)(5)
→v=(4^i)+(0.5^i+0.375^j)=(4.5^i+0.375^j)m/s
Final Answer:
The velocity of the particle at t=5s is:
→v=4.5^i+0.375^jm/s
- Charge of the particle, q=2μC=2×10−6C
- Mass of the particle, m=1.6g=1.6×10−3kg
- Initial velocity, →v0=4^im/s
- Electric field, →E=80^i+60^jN/C
The force on the particle due to the electric field is given by:
→F=q→E
Substituting the values:
→F=(2×10−6)(80^i+60^j)=(1.6×10−4^i+1.2×10−4^j)N
→a=→Fm=(1.6×10−4^i+1.2×10−4^j)1.6×10−3=(0.1^i+0.075^j)m/s2
The velocity of the particle at any time t can be calculated using the equation:
→v=→v0+→at
Substituting the values:
→v=(4^i)+(0.1^i+0.075^j)(5)
→v=(4^i)+(0.5^i+0.375^j)=(4.5^i+0.375^j)m/s
The velocity of the particle at t=5s is:
→v=4.5^i+0.375^jm/s
Similar Questions
A charged particle of charge 2μC and mass 10 milligram, moving with a velocity of 1000m/s enters a uniform electric field of strength 103N/C directed perpendicular to its direction of motion. Find the velocity and displacement of the particle after 10s.
View SolutionA charged particle ( mass m and charge q) moves along X axis with velocity V0 . When it passes through the origin it enters a region having uniform electric field →E=−Eˆj which extends upto x=d . Equation of path of electron in the region . x>d is :
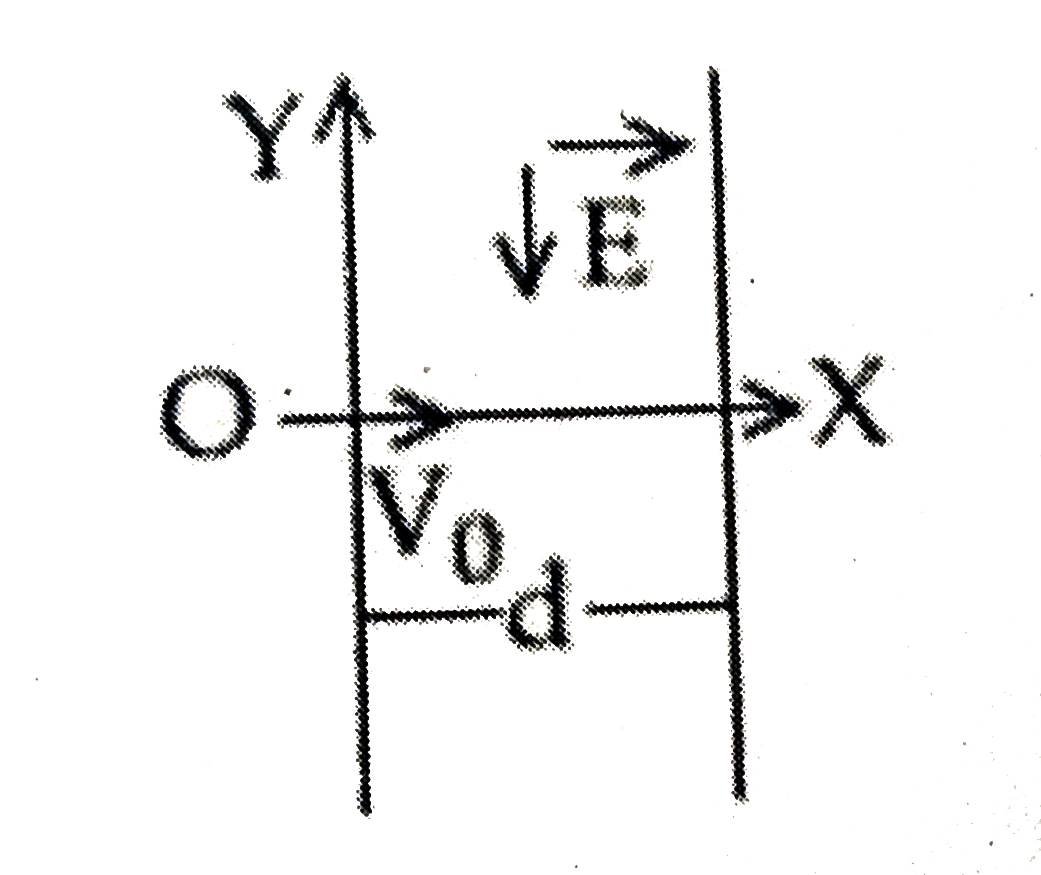
View Solution
Knowledge Check
A particle of mass 1 g moving with a velocity →v1=3ˆi−2ˆj ms−1 experiences a perfectly in elastic collision with another particle of mass 2 g and velocity →v2=4ˆj−6ˆk ms−1. The velocity of the particle is
A2.3ms−1B4.6ms−1C9.2ms−1D6ms−1A particle of charge q=4μC and mass m = 10 mg starts moving from the origin under the action of an electric field →E=4ˆi and magnetic field →B=(0.2T)ˆk. Its velocity at (x, 3, 0) is (4→i+3→j). The value of x is -
A11516mB12516mC13516mD14516mA particle moves in a straight line with an acceleration ams−2 at time 't' seconds where a=−1t2 When t=1 the particle has a velocity of 3ms−1. Find the velocity of the particle at t=4s.
A5.25m/sB1.25m/sC2.25m/sD8.25m/s
The velocity of a particle at t=0is→U=4ˆi+3ˆjm/sec and a constant acceleration is →a=6ˆi+4ˆjm/sec2. Find the velocity and displacement of the particle at t=2sec.
View SolutionA particle is moving with initial velocity 1m/s and acceleration a=(4t+3)ms2. Find velocity of particle at t=2sec.
View SolutionA particle having mass m and charge q is released from the origin in a region in which electric field and magnetic field are ginen by
B=+B0ˆj and →E=+E0ˆi.
Find the speed of the particle as a function of its X-coordinate.
View SolutionA particle moves with a velocity (5ˆi−3ˆj+6ˆk)ms−1 under the influence of a constant force →F=(10ˆi−10ˆj+20ˆk) N . The instantaneous power applied to the particle is
View SolutionA particle of mass 1.0 g moving with a velocity →v1=(3ˆi−2ˆj) m/s experiences a perfectly inelastic collision with another particle of mass 2.0 g and velocity →v2=(4ˆi−6ˆj) m/s. Find the magnitude of the velocity vector →v of the coalesced particles.
View Solution
