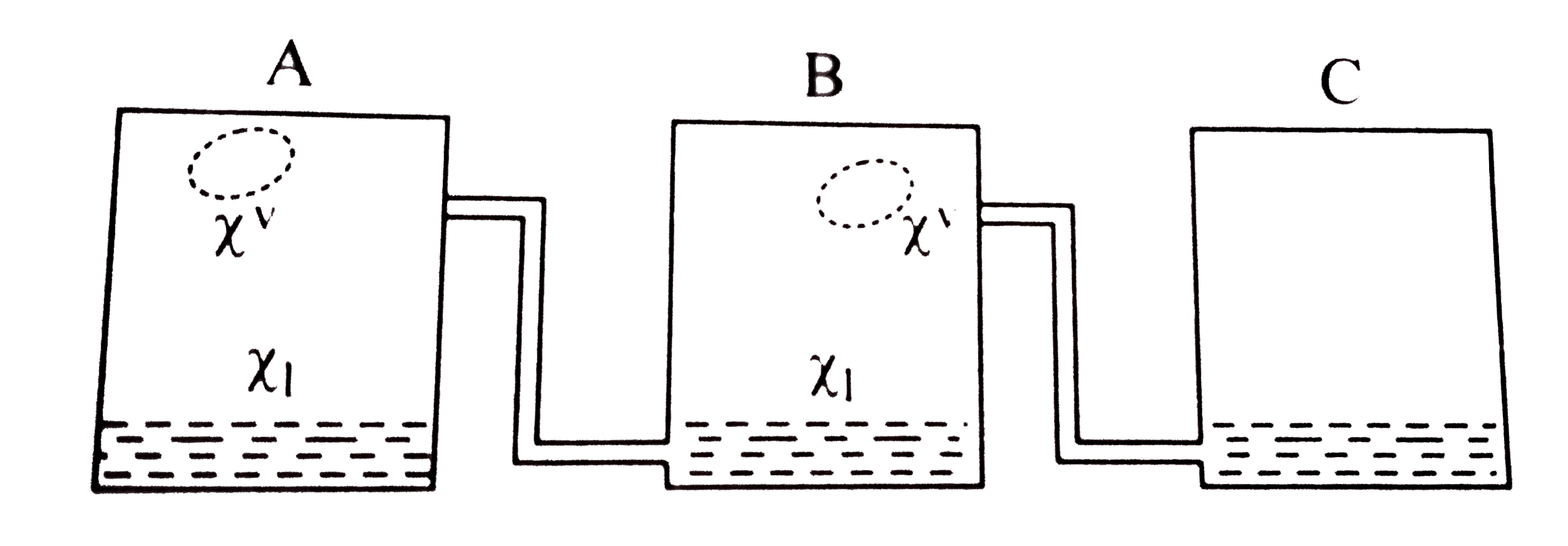
Liquids $A$ and $B$ form an ideal mixture, in which the mole fraction of $A$ is $0.25$ . At temperature $T$ , a small quantity of the vapour in equilibrium with the liquid is collected and condensed. This process is repeated for a second time with first condensate. The second condensate now contains 0.645 mole fraction of $A$ . Calculate the ratio $(P_{A}^{\circ} / P_{B}^{\circ})$ . What will be the mole fraction of $B$ in the third condensate?

Text Solution

Verified by Experts

Mole fraction of $A (χ_{A}) = 0.25$
Mole fraction of $B (χ_{B}) = 0.75$
After first condensation
Partial presssure of $A (p_{A}) = P_{A}^{\circ} χ_{A} = P_{A}^{\circ} \times 0.25$
Partial presssure of $B (p_{B}) = P_{B}^{\circ} \times 0.75$
$∴ \frac{p_{A}}{p_{B}} = \frac{P_{A}^{\circ}}{P_{B}^{\circ}} \times \frac{0.25}{0.75}$
After second condensation
Mole fraction of $A (χ_{A}) = 0.645$
Mole fraction of $B (χ_{B}) = 1 - 0.645 = 0.355$
Ratio of partial pressures
$p_{A}^{/p_(A)^} = {(\frac{p_{A}^{\circ}}{p_{B}^{\circ}})}^{2} \times \frac{0.25}{0.75} = \frac{0.645}{0.345}$
$∴ {(\frac{p_{A}^{\circ}}{p_{B}^{\circ}})}^{2} = \frac{0.645}{0.345} \times \frac{0.75}{0.25}$
$∴ \frac{P_{A}^{\circ}}{P_{B}^{\circ}} = 2.33$
After third condensation
$p_{A}^{'/p_(A)^}' = {(\frac{p_{A}^{\circ}}{p_{B}^{\circ}})}^{3} \times \frac{0.25}{0.75} = {(2.33)}^{3} \times \frac{0.25}{0.75} = 4.242$
$∴$ Mole fraction of B
$p_{B}^{'/p_(B)^}' + p_{B}' = \frac{1}{5.42} = 0.191$ =Mole fraction of $B$

Updated on:21/07/2023

Two liquids $A$ and $B$ have $P_{A}^{\circ}$ and $P_{B}^{\circ}$ in the ratio of $1 : 3$ and the ratio of number of moles of $A$ and $B$ in liquid phase are $1 : 3$ then mole fraction of $A$ in vapour phase in equilibrium with the solution is equal to :
A0.1
B0.2
C0.5
D $1.0$
Two liquids A and B have $P_{A}^{0} : P_{B}^{0} = 1 : 3$ at a certain temperature. If the mole fraction ratio of $x_{A} : x_{B} = 1 : 3$ , the mole fraction of A in vapour in equilibrium with the solution at a given temperature is :
A0.1
B0.2
C0.5
D $1.0$
Two liquids $A$ and $B$ have $P_{A}^{\circ}$ and $P_{B}^{\circ}$ in the ratio of $1 : 3$ and the ratio of number of moles of $A$ and $B$ in liquid phase are $1 : 3$ then mole fraction of $A$ in vapour phase in equilibrium with the solution is equal to :
A $0.1$
B $0.2$
C $0.5$
D $1.0$