The molar conductivity of KCl solution at different concentrations at 298K is given below :
corM(molL−1)∧m(Scm2mol−1)0.000198148.610.000309148.290.000521147.810.000989147.09
Show that a plot between ∧m and √c is a straight line. Determine the value of ∧∘m and A for KCl .
To solve the problem, we will follow these steps:
Step 1: Calculate the square root of the concentration values
We will take the square root of each concentration value (c) given in the table.
- For c=0.000198mol L−1:
√c=√0.000198≈0.01407
- For c=0.000309mol L−1:
√c=√0.000309≈0.01757
- For c=0.000521mol L−1:
√c=√0.000521≈0.02283
- For c=0.000989mol L−1:
√c=√0.000989≈0.03143
Step 2: Prepare the data for plotting
Now we have the following pairs of √c and λm:
√c:0.01407,0.01757,0.02283,0.03143λm:148.61,148.29,147.81,147.09
Step 3: Plotting the graph
We will plot λm (y-axis) against √c (x-axis).
Step 4: Determine if the plot is a straight line
From the graph, we can visually inspect if the points form a straight line. If they do, we can proceed to find the slope and intercept.
Step 5: Determine the slope (A) and intercept (λ0m)
The relationship between λm and √c can be expressed as:
λm=λ0m−A√c
Where:
- A is the slope of the line,
- λ0m is the intercept on the y-axis.
To find A, we can use two points from our data. Let’s take the first and last points for calculation:
1. (0.01407,148.61)
2. (0.03143,147.09)
Using the slope formula:
A=Y2−Y1X2−X1
Substituting the values:
A=147.09−148.610.03143−0.01407=−1.520.01736≈−87.5
Step 6: Determine λ0m
To find λ0m, we can substitute one of the points into the equation:
Using (0.01407,148.61):
148.61=λ0m−(−87.5)(0.01407)
Calculating:
148.61=λ0m+1.23⟹λ0m≈147.38
Final Results
- λ0m≈147.38S cm2mol−1
- A≈87.5S cm2mol−1
We will take the square root of each concentration value (c) given in the table.
√c=√0.000198≈0.01407
√c=√0.000309≈0.01757
√c=√0.000521≈0.02283
√c=√0.000989≈0.03143
Now we have the following pairs of √c and λm:
We will plot λm (y-axis) against √c (x-axis).
From the graph, we can visually inspect if the points form a straight line. If they do, we can proceed to find the slope and intercept.
The relationship between λm and √c can be expressed as:
λm=λ0m−A√c
Where:
- A is the slope of the line,
- λ0m is the intercept on the y-axis.
2. (0.03143,147.09)
A=Y2−Y1X2−X1
Substituting the values:
A=147.09−148.610.03143−0.01407=−1.520.01736≈−87.5
To find λ0m, we can substitute one of the points into the equation:
Using (0.01407,148.61):
148.61=λ0m−(−87.5)(0.01407)
Calculating:
148.61=λ0m+1.23⟹λ0m≈147.38
- λ0m≈147.38S cm2mol−1
- A≈87.5S cm2mol−1
Topper's Solved these Questions
- ELECTROCHEMISTRYBOOK - CENGAGE CHEMISTRYCHAPTER - ELECTROCHEMISTRYEXERCISE - Solved Examples (Electrochemical Cell)
36 Videos
- ELECTROCHEMISTRYBOOK - CENGAGE CHEMISTRYCHAPTER - ELECTROCHEMISTRYEXERCISE - Solved Examples(Electrolysis And Electrolytic Cells)
12 Videos
- D AND F BLOCK ELEMENTSBOOK - CENGAGE CHEMISTRYCHAPTER - D AND F BLOCK ELEMENTSEXERCISE - Archives Subjective
29 Videos
- GENERAL PRINCIPLES AND PROCESS OF ISOLATION OF ELEMENTSBOOK - CENGAGE CHEMISTRYCHAPTER - GENERAL PRINCIPLES AND PROCESS OF ISOLATION OF ELEMENTSEXERCISE - Archives (Subjective)
14 Videos
Similar Questions
The molar conductivity of KCl solution at different concentrations at 298K is given below :
corM(molL−1)∧m(Scm2mol−1)0.000198148.610.000309148.290.000521147.810.000989147.09
Show that a plot between ∧m and √c is a straight line. Determine the value of ∧∘m and A for KCl .
View SolutionThe molar conductivity (Λm) of KCl solutions at different concentrations at 298 K is plotted as shown in the figure below:
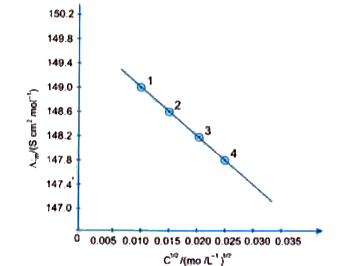
Determine the value of Λ∘m and A for KCl.
View Solution
Knowledge Check
Molar conductivity of 0.15 M solution of KCI at 298 K, if its conductivity is 0.0152 S cm−1 will be
A124Ω−1cm2mol−1B204Ω−1cm2mol−1C101Ω−1cm2mol−1D300Ω−1cm2mol−1Calculate molar conductivity of 0.15 M solution of KCl at 298 K if its conductivity is 0.0152 S cm−1
A124Ω−cm2mol−1B204Ω−1cm2mol−1C101Ω−1cm2mol−1D30Ω−1cm2mol−1Molar conductivity of 0.15 M solution of KCI at 298 K, if its conductivity is 0.0152 S cm−1 will be
A124Ω−cm2mol−1B204Ω−1cm2mol−1C101Ω−1cm2mol−1D30Ω−1cm2mol−1
The conductivity of sodium CHMloride at 298K has been determine at different concentrations and the results are given below :
Concentration(M):0.0010.0100.0200.0500.100102×k(Sm−1):1.23711.8523.1555.531.06.74
Calculate ∧m for all concentrations and draw a plot between ∧m and c1/2. Find the value of ∧∘m.
View SolutionThe conductivity of sodium Chloride at 298K has been determine at different concentrations and the results are given below :
Concentration(M):0.0010.0100.0200.0500.100102×k(Sm−1):1.23711.8523.1555.531.06.74
Calculate ∧m for all concentrations and draw a plot between ∧m and c1/2. Find the value of ∧∘m.
View SolutionThe conductivity of 0.20 M KCl solution at 298 K is 0.025Scm−1 . Calculate its molar conductivity .
View SolutionThe conductivity of a 0.01 M solution of acetic acid at 298 k is 1.65×10−4Scm−1. Calculate molar conductivity (∧m) of the solution.
View SolutionThe conductivity of 0.20M solution of KCl at 298K is 0.0248Scm−1 . Calculate its molar conductivity.
View Solution
