How does free energy change explain the criterion of spontaneity?
The total entropy change for a system and its surroundings accompanying a process is given by,
ΔStotal=ΔSsystem+ΔSsurr
By second law, for a spontaneous process,
ΔStotal>0. If +ΔH is the enthalpy change (or enthalpy increase) for the process, or a reaction at constant temperature (T) and pressure, then enthalpy change (or enthalpy decrease) for the surroundings will be −ΔH .
∴ΔSSurr=−ΔHT
∵ΔStotal=ΔSsystem+ΔSsurr
∴ΔStotal=ΔSsystem−ΔTT
∴TΔStotal=TΔSsystem−ΔH
∴−TΔStotal=−TΔSSystem−ΔH
∴−TΔStotal=ΔH−TΔSsystem
By Gibbs equation,
ΔG=ΔH−TΔS
By comparing above two equations,
∴ΔG=−TΔStotal
As ΔStotal increase, ΔG decrease.
For a spontaneous process, ΔStotal>0
which is according to second law of thermodynamics. ∴ΔG<0
Hence in a spontaneous process, Gibbs free energy decreases (ΔG<0) while entrophy increase (ΔS>0) .
Therefore for a non-spontaneous process Gibbs free energy increases (ΔG>0).
It can be concluded that for a process at equilibrium, ΔG=0
Hence (i) For the spontaneous process, ΔG<0.
(ii) For the non-spontaneous process, ΔG>0.
(iii) For the process at equilibrium, ΔG=0.
Similar Questions
Knowledge Check
Which of the following describes the criterion of spontaneity?
AΔS(Total)>0BΔGTP>0CΔHTP>0DNone of aboveWhich of the following expression represent the criterion of spontaneity ?
A(δS)E, V<0B(δG)T,P<0C(δH)T,P<0D(δG)S,V<0A reaction is spontaneous if free energy change ΔG , for the reaction is negative . The question arises, how we can explain , the spontaneous of reversible reaction taking the example of dissociation equilibrium N2O4⇔2NO2(g) the variation of free energy with the fraction of N2O4 dissociated under standard conditions is shown in the figure below.
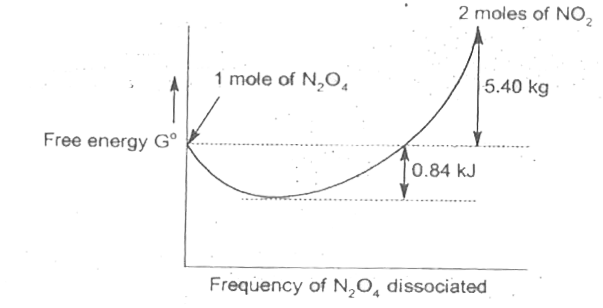
The standard free energy change for the conversion of 1 mole of N2O4 into 2 moles of NO2 isA5.40 KJB−5.40KJC(5.4+0.84 )KJD(5.40 +2 ×0.84)kJ
Free Energy Change And Spontaneity
View SolutionA reaction is spontaneous if free energy change ΔG , for the reaction is negative .
The question arises, how we can explain , the spontaneous reversible reaction taking the example of dissociation equilibrium ,N2O4⇔2NO2(g).
the variation of free energy with the fraction of N2O4 dissociated under standard conditions is shown in the figure below.

The standard free energy change for the conversion of 1 mole of N2O4 into 2 moles of NO2 is
View SolutionHow are internal energy change( at constant pressure) , free energy change and entropy change related to one another?
View SolutionA reaction is spontaneous if free energy change ΔG , for the reaction is negative . The question arises, how we can explain , the spontaneous of reversible reaction taking the example of dissociation equilibrium N2O4⇔2NO2(g) the variation of free energy with the fraction of N2O4 dissociated under standard conditions is shown in the figure below.
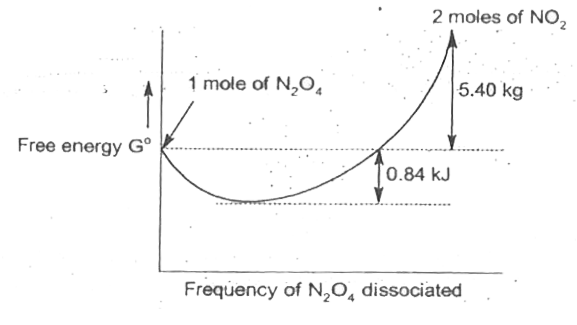
When 1 mole of N2O4 changes into the equilibrium mixture the volume of ΔG∘ is
View SolutionA reaction is spontaneous if free energy change ΔG , for the reaction is negative . The question arises, how we can explain, the spontaneous of reversible reaction taking the example of dissociation equilibrium N2O4⇔2NO2(g) the variation of free energy with the fraction of N2O4 dissociated under standard conditions is shown in the figure below.
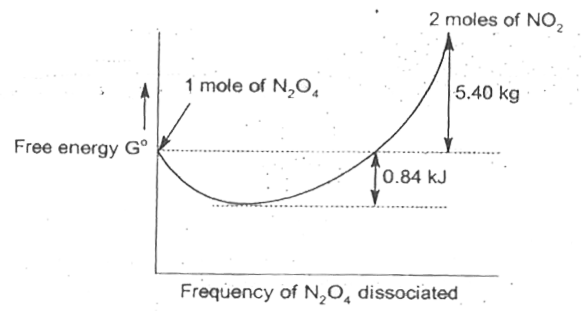
When 2 moles of NO2 change into equilibrium mixture the value of ΔG∘ is
View Solution
