A parallel plate air capacitor has a capacitance C. When it is half filled with a dielectric of dielectric constant 5, the percentage increase in the capacitance will be
400%
66.6%
33.3%
200%
The correct Answer is:B
To solve the problem of finding the percentage increase in capacitance when a parallel plate air capacitor is half-filled with a dielectric of dielectric constant 5, we can follow these steps:
Step 1: Understand the Initial Capacitance
The initial capacitance C of a parallel plate capacitor filled with air (where the dielectric constant k=1) is given by the formula:
C=Aϵ0d
where:
- A is the area of the plates,
- ϵ0 is the permittivity of free space,
- d is the distance between the plates.
Step 2: Analyze the Configuration After Filling with Dielectric
When the capacitor is half-filled with a dielectric of dielectric constant k=5, we can treat the capacitor as two capacitors in series:
1. Capacitor 1 (C1): The portion filled with the dielectric (height = d2).
2. Capacitor 2 (C2): The portion filled with air (height = d2).
Step 3: Calculate the Capacitance of Each Section
For Capacitor 1 (C1):
C1=Aϵ0k1d1=Aϵ0⋅5d2=10Aϵ0d
For Capacitor 2 (C2):
C2=Aϵ0k2d2=Aϵ0⋅1d2=2Aϵ0d
Step 4: Find the Equivalent Capacitance
Since C1 and C2 are in series, the equivalent capacitance Ce is given by:
1Ce=1C1+1C2
Substituting the values:
1Ce=110Aϵ0d+12Aϵ0d
=d10Aϵ0+d2Aϵ0
Finding a common denominator (which is 10):
=d10Aϵ0+5d10Aϵ0=6d10Aϵ0
Thus,
Ce=10Aϵ06d=5Aϵ03d
Step 5: Calculate the Percentage Increase in Capacitance
Now, we can find the percentage increase in capacitance:
Percentage Increase=(Ce−CC)×100
Substituting C=Aϵ0d and Ce=5Aϵ03d:
Percentage Increase=⎛⎜⎝5Aϵ03d−Aϵ0dAϵ0d⎞⎟⎠×100
=⎛⎝53−11⎞⎠×100=(5−33)×100=(23)×100≈66.67%
Conclusion
The percentage increase in capacitance when the capacitor is half-filled with a dielectric of dielectric constant 5 is approximately 66.67%.
The initial capacitance C of a parallel plate capacitor filled with air (where the dielectric constant k=1) is given by the formula:
C=Aϵ0d
where:
- A is the area of the plates,
- ϵ0 is the permittivity of free space,
- d is the distance between the plates.
When the capacitor is half-filled with a dielectric of dielectric constant k=5, we can treat the capacitor as two capacitors in series:
1. Capacitor 1 (C1): The portion filled with the dielectric (height = d2).
2. Capacitor 2 (C2): The portion filled with air (height = d2).
For Capacitor 1 (C1):
C1=Aϵ0k1d1=Aϵ0⋅5d2=10Aϵ0d
C2=Aϵ0k2d2=Aϵ0⋅1d2=2Aϵ0d
Since C1 and C2 are in series, the equivalent capacitance Ce is given by:
1Ce=1C1+1C2
Substituting the values:
1Ce=110Aϵ0d+12Aϵ0d
=d10Aϵ0+d2Aϵ0
Finding a common denominator (which is 10):
=d10Aϵ0+5d10Aϵ0=6d10Aϵ0
Thus,
Ce=10Aϵ06d=5Aϵ03d
Now, we can find the percentage increase in capacitance:
Percentage Increase=(Ce−CC)×100
Substituting C=Aϵ0d and Ce=5Aϵ03d:
Percentage Increase=⎛⎜⎝5Aϵ03d−Aϵ0dAϵ0d⎞⎟⎠×100
=⎛⎝53−11⎞⎠×100=(5−33)×100=(23)×100≈66.67%
The percentage increase in capacitance when the capacitor is half-filled with a dielectric of dielectric constant 5 is approximately 66.67%.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTIPSCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Energy Stored In Capacitor
18 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTIPSCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Van De Graaff Generator
5 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTIPSCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Effect Of Dielectric On Capacitance
6 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT FINGERTIPSCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason
15 Videos
- MAGNETISM AND MATTER BOOK - NCERT FINGERTIPSCHAPTER - MAGNETISM AND MATTER EXERCISE - Assertion And Reason
15 Videos
Similar Questions
A parallel plate air capacitor has a capacitance C. The capacitor is half-filled with a dielectric of dielectric constant 5. The percentage increase in the capacitance must have been -- %.
View SolutionA paralle plate air capacitor has capacitance C. Now half of the space is fillel with a dielectric material with dielectric constant K as shown in figure . The new capacitance is C . Then

View Solution
Knowledge Check
A parallel plate air capacitor has a capacitance C. When it is half filled with a dielectric of dielectric constant 5, then the percentage increase in the capacitance will be
 A400%B66.6%C33.3%D200%
A400%B66.6%C33.3%D200%A parallel plate air capacitor has a capacitance C. When it is half filled with a dielectric (K=2), the percentage increase in the cpacitance will be
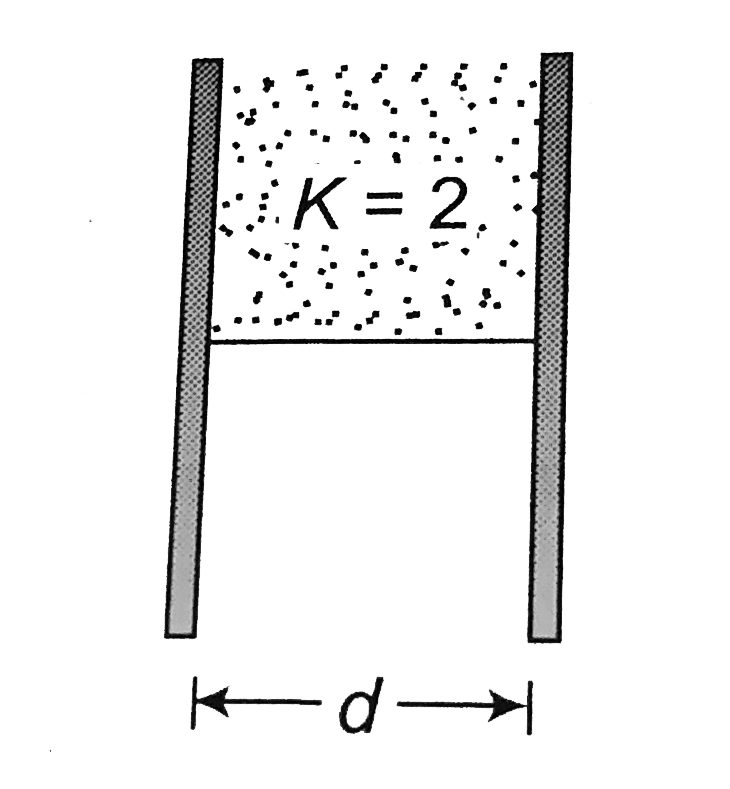 A25%B50%C75%D100%
A25%B50%C75%D100%A parallel plate air capacitor has capacitance C. Half of space between the plates is filled with dielectric of dielectric constant K as shown in figure . The new capacitance is C . Then
 AC=C[KK+1]BC'=C[2KK+1]CC'=2CK+1DC'=C[1+K2]
AC=C[KK+1]BC'=C[2KK+1]CC'=2CK+1DC'=C[1+K2]
Assertion (A): If the distance between plates of a parallel plate capacitor is halved and the intervening space is filled with a dielectric of dielectric constant 3, the capacitance of capacitor becomes 6 times of its original capacitance.
Reason (R) : The capacitance of a capacitor does not depend on the material of the metal plates.
View SolutionA parallel plate capacitor has capacitance C. If it is equally filled with parallel layers of materials of dielectric constants K1 and K2 its capacity becomes C1. The ratio of C1→C is
View SolutionA parallel plate capacitor with air between the plates has a capacitance C. If the distance between the plates is doubled and the space between the plates is filled with a dielectric of dielectric constant 6, then the capacitance will become.
View SolutionA capacitor of plate area A and separation d is filled with two dielectrics of dielectric constant K1=6andK2=4. New capacitance will be

View SolutionA parallel plate capacitor A is filled with a dielectric whose dielectric constant varies with applied voltage as K = V. An identical capacitor B of capacitance C0 with air as dielectric is connected to voltage source V0=30V and then connected to the first capacitor after disconnecting the voltage source. The charge and voltage on capacitor.
View Solution
