For any arbitrary motion in space, which of the following relations are true?
a) vaverage=(1/2)(v(t1+v(t2)
b) vaverage=[r(t2)−rt1t2−t1
v(t)=v(0)+at
d) aaverage=[v(t2)−vt1t2−t1
The average stands for average of the quantity over time interval t1 to t_(2)
→vaverage=12[→v(t1)+→v(t2)]
→vaverage=→r(t2)−→r(t1)t2−t1
→v(t)=→v(0)+→at
→r(t)=→r(0)+→v(0)t+12→at2
The correct Answer is:B
The relation (b) is true, others are false because relations (a), (c ) and (d) hold only for uniformly
Topper's Solved these Questions
- BOOK - NCERT FINGERTIPSCHAPTER - MOTION IN A PLANE EXERCISE - Motion In A Plane With A Constant Acceleration2videos
MOTION IN A PLANE
- BOOK - NCERT FINGERTIPSCHAPTER - MOTION IN A PLANE EXERCISE - Relative Velocity In Two Dimensions4videos
MOTION IN A PLANE
- BOOK - NCERT FINGERTIPSCHAPTER - MOTION IN A PLANE EXERCISE - Vector Addition - Analytical Method11videos
MOTION IN A PLANE
- BOOK - NCERT FINGERTIPSCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assertion And Reason15videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NCERT FINGERTIPSCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Assertion And Reason15videos
MOTION IN A STRAIGHT LINE
Similar Questions
For any arbitrary motion in space, which of the following relations are true?
(a) vaverage=(1/2)(v(t1)+v(t2))
(b) vaverage=[r(t2)−r(t1]/(t2−t1)
(c) v(t)=v(0)+at
(d) aaverage=[v(t2)−v(t1)]/(t2−t1)
The average stands for average of the quantity over time interval t1 to t2View SolutionFoe an arbitrary motion in sparce, which ot the following relations are true :
(a0 −−−−−−→avera≥=12[→v(t10+→v(t2)]
(b) →–––(avera≥)=[→r9t2)−→r(t−10]/(t2−t10
(c ) →v(t)=→v(0)+→at
(d) →r9t)=→r90)+→v(0)t+(1/2)→at2
(e) →aavera≥=[→v(t2)−→v(t−2)]/(t2−t1)1<br>Theavera≥standsforavera≥ofthewuantityoverthetime∫erval t_1andt2 ].View Solution
Knowledge Check
- Question 1 - Select One
Which of the following statements is correct about the average velocity of a particle?
(The average stands for average of the quantity over the time interval , t1 to t2 )A→vaverage=→v(t2)−→v(t1)2B→vaverage=→aaverage(t2−t1)C→vaverage=→v(t2)+→v(t1)2D→vaverage=→r(t2)+→r(t1)t2−t−1 - Question 2 - Select One
Let→V=vxˆi+vyˆj+vzˆk, then ∫t2t1Vy represents: (for the duration t1→t2)
ADistance travelled along y-axisBDisplacement along y-axisCTotal displacement - displacement along y-axisDTotal distance travelled - distance travels along y-axis - Question 3 - Select One
The quantity ∫t2t1→Vdt represents:
ADistance travelled during t1→t2BDisplacement during t1→t2CAverage acceleration during t1→t2DNone of these
The velocity-time graph of a particle in one-dimensional motion is shown in Fig.:
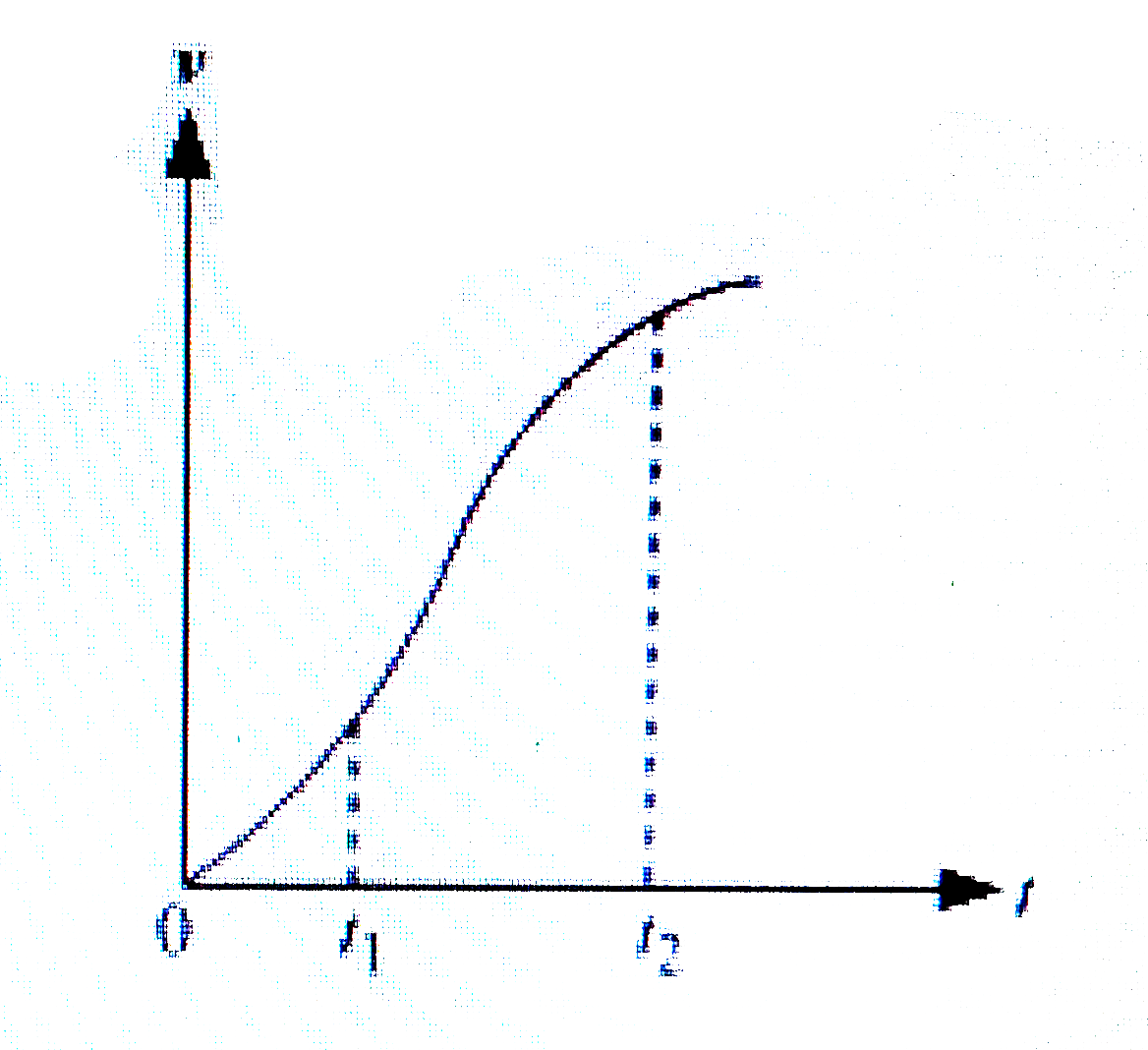
(a) Which of the following formulae are correct for describing the motion of the particle over the time-interval t1 to t2:
(a) x(t2)=x(t1)+v(t1)(t2−t1)+(1/2)a(t2−t1)2
(b) v(t2)=v(t1)+a(t2−t1)
(c) vaverage=(x(t2)−x(t1))/(t2−t1)
(d) aaverage=(v(t2)−v(t1))/(t2−t1)
(e) x(t2)=x(t1)+vaverage(t2−t1)+(1/2)aaverage(t2−t1)2
(f) x(t2)−x(t1) = area under the v−t curve bounded by the t-axis and the dotted line shown.View SolutionExamine the following plots and predict whether in (i) P1<P2andT1>T2 , in (ii) T1=T2<T3, in (iii) V1>V2,in(iv)P1>P2orP2>P1
_E01_267_Q01.png) View Solution
View SolutionA particle moves with a velocity v(t)=(12)kt2 along a straight line . Find the average speed of the particle in a time t.
View SolutionGive expression for average value of a.c. voltage V=V0sinωt over interval t=0 to t=πω
View SolutionA body is moving with variable acceleartion (a) along a straight line. The average acceleration of body in time interval t1 to t2 is :-
View Solution

