How many geometrical isomers are there for
(a) ${[C o {(N H_{3})}_{2} C I_{4}]}_{2}^{Θ}$
(b) ${[A u C I_{2} B r_{2}]}_{2}^{Θ}$ (square planar) (c ) ${[C u C I_{2} B r_{2}]}_{2}^{2 -}$ (tetrahedral) .

A

Two cis and trans, no geometrical isomers .

B

Two cis and trans , two cis and trans.

C

No geometrical isomers , two cis and trans .

D

No geometrical isomers , no geometrical isomers .

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

${[C o {(N H_{3})}_{2} C l_{4}]}_{2}^{-} {[A u C l_{2} B r_{2}]}_{2}^{-}$

|

Updated on:21/07/2023

Class 12 CHEMISTRY COORDINATION COMPOUNDS

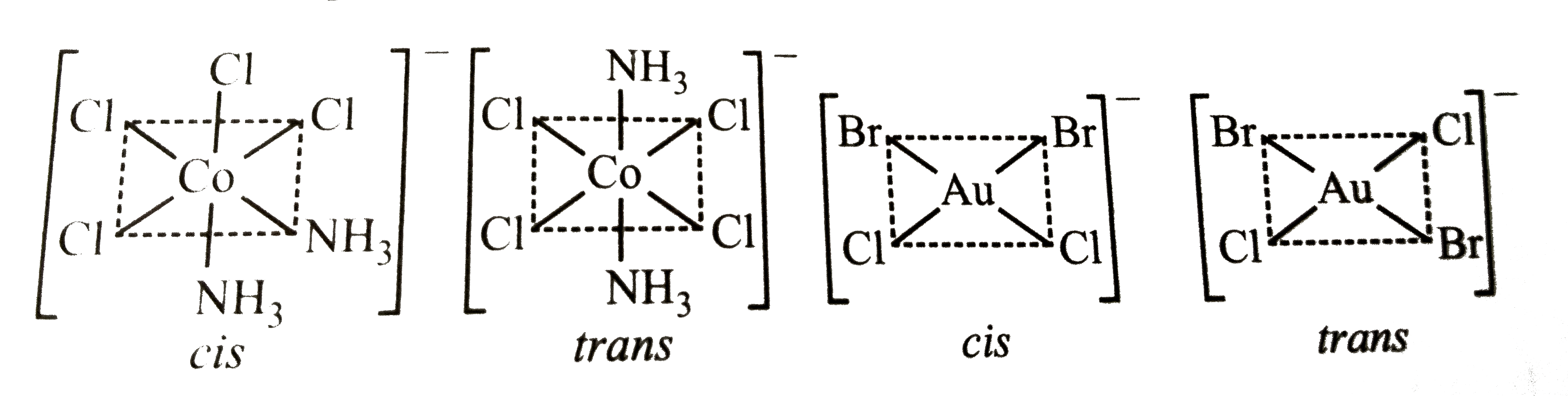
Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The number of geometrical isomers for octahedral ${[C o {(N H_{3})}_{2} C l_{4}]}_{2}^{-}$ and square planar ${[A u C l_{2} B r_{2}]}_{2}^{-}$ respectively are :
ATwo cis and trans, no geometrical isomers
BTwo cis and trans, two cis and trans
CNo geometrical isomers, two cis and trans
DNo geometrical isomers, no geometrical isomer
Question 2 - Select One
The number of geometrical isomers for $[P t {(N H_{3})}_{2} C I_{2}]$ is
A3
B4
C1
D2
Question 3 - Select One
How many geometrical isomers are possible for ${[P d^{2 +} {(N H_{2} - C H (C H_{3}) - C O_{2}^{-})}_{2}]}_{2}^{0}$
A $2$
B $3$
C $4$
D $6$

NCERT FINGERTIPS-COORDINATION COMPOUNDS -Isomerism In Coordination Compounds

Home
Ask Doubt
Profile