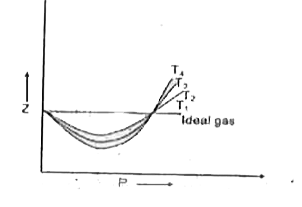
Assertion : Compressibility factor (Z) is the ratio of actual molar volume of a gas to the molar volume of it , if it were an ideal gas at that temperature and pressure .
Reason : At high pressure all the gases have Z lt 1 and can be easily compressed .

A

If both assertion and reason are true and reason is the correct explanation of assertion .

B

If both assertion and reason are true but reason is not the correct explanation of assertion .

C

If assertion is true but reason is false .

D

If both assertion and reason are false .

Text Solution

Verified by Experts

The correct Answer is:C

At high pressure all the gases have Z gt 1 and are difficult to compress.

|

Updated on:21/07/2023

Class 11 CHEMISTRY STATES OF MATTER

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The compressibility factor Z for an ideal gas will be .........
A $1.5$
B $1.0$
C $2.0$
Dzero
Question 2 - Select One
At high pressure, the compressibility factor Z is equal to
Aunity
B $1 - \frac{P b}{R T}$
C $1 + \frac{P b}{R T}$
DZero
Question 3 - Select One
The rise is compressibility factor ( $Z$ ) with increasing pressure of a gas is due to
Avan der Waals constant $a$
Bvan der Waals constant $b$
CBoth ( $a$ ) and ( $b$ )
DNot related to either $a$ or $b$

NCERT FINGERTIPS-STATES OF MATTER -Assertion And Reason

Home
Ask Doubt
Profile