The mean of the frequency distribution of a variable is 25 and its median is 23. Find the value od ita mode.Solution in Bengali
We know that the relation between mean, median and mode is given by Mean- Mode=3(Mean-Median)
∴Mode=3×Median−2×Mean=3×23=69−50=19
∴
the required mode=19.
Topper's Solved these Questions
Similar Questions
What are the measure of central tendency. Define them.
View SolutionHow arithmetic mean is changed when the values of the variable are added or subtracted by a constant number ?
View Solution
Find the median of the above frequency distribution by constructing ogives of less than and more than type cumulative frequencies along the same axes .
View SolutionIf the mean and mode of a frequency distribution be 53.4 and 55.2 respectively, find the median
View SolutionIf the mode and median of a frequency distribution are 31 and 37 respectively, find the arithmetic mean.
View SolutionIf the mean and median of a frequency distribution are 35 and 33 respectively, find mode.
View SolutionThe mean of a distribution is 23, the median is 24 and the mode is 25.5. It is most likely that this distribution is :
View SolutionFind mode of the following frequency distribution.
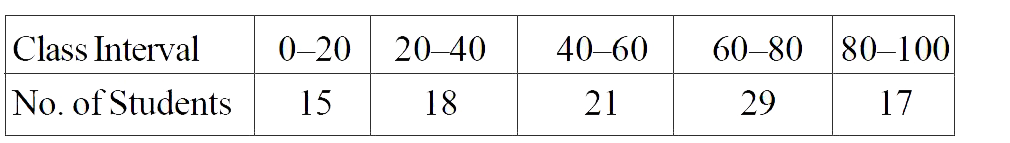
The mean of above distribution is 53. Empirical formula to find approximate value of median.
View SolutionIf in a frequency distribution, the mean and median are 21 and 22 respectively, then its mode is approximately :
View SolutionIn a frequency distribution , the mean and median are 21 and 22 respectively , then its mode is approximately
View SolutionIf in a frequency distribution, the mean and median are 21 and 22 respectively, then its mode is approximately
View SolutionIf in the frequency distribution,the mean and median are 21 and 22 respectively,then find its mode.
View SolutionThe median and mode of a frequency distribution are 26 and 29 then its mean is
View SolutionIn a frequancy distribution,if the mean = 35 & median = 33, then find the mode of the frequency distribution.
View SolutionIf in a frequency distribution, the mean and median are 25 and 26 , then its mode is approximately.
View Solution
