Find the Median and Mode of the variable from the following frequency distribution table:

Text Solution
Here, the class ranges are not equal. There will be no problem in determining the median, but to find the mode, the class ranges must be mede equal. Let us draw the following table of cumulative frequency for median.

We know that median, Me =xl+N2−Ff×c
Here,N2=1202=60
∴ the medina lies from 20.5 to 25.5, ∴xl=20.5.F+59andf=19 c= the range of the class interval in which lie 25.5−20.5=5
∴ the required median, Me
=20.5+60−5919×5=20.5+519=20.5+0.263=20.76
Now ,rearranging the above frequency distribution table by equalising the class-ranges we get,

we know that Mode , M0=xl+f0−f−12f0−f−1−f×c
Here xl=10.5,f0=52,f−1=7,f−1=34andc=10
∴the required mode =10.5+52−72×52−7−34×10
=10.5+45104−41×10=10.5+45063=10.5+7.143=17.643
|
Topper's Solved these Questions
Similar Questions
Find the median for the following frequency distribution

View SolutionFind the median for the following frequency distribution

View SolutionFind mode of the following frequency distribution.
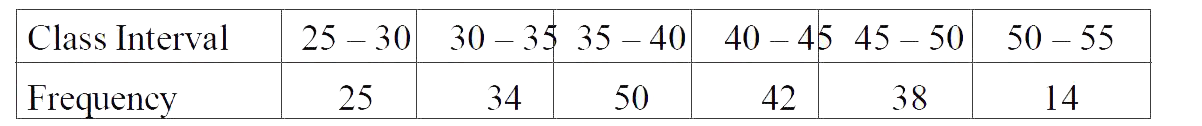
View SolutionFind the median for the following frequency distribution:
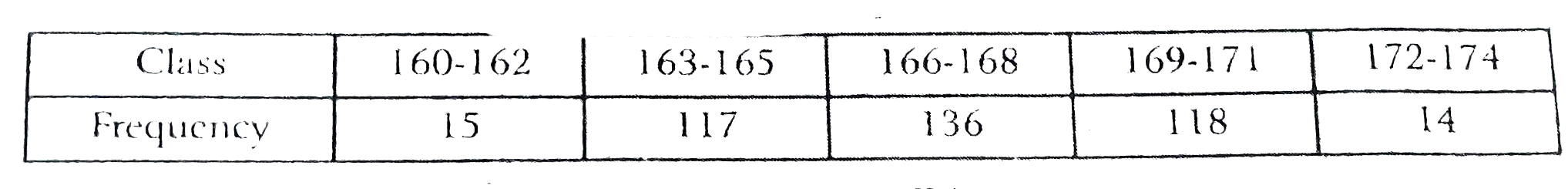
View SolutionFind the median for the following frequency distribution:

View SolutionFind the median for the following frequency distribution:
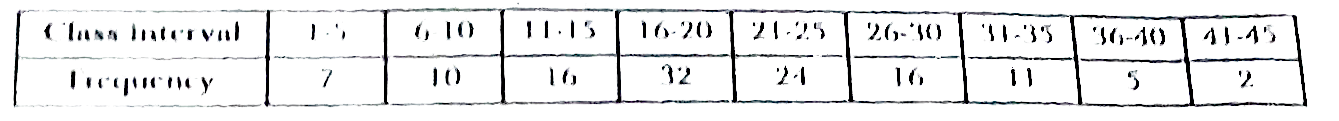
View SolutionFind the median for the following frequency distribution:
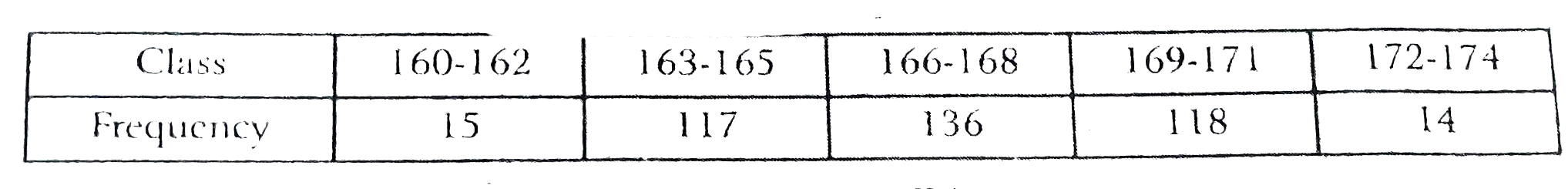
View SolutionFind the mode of the following frequency distribution
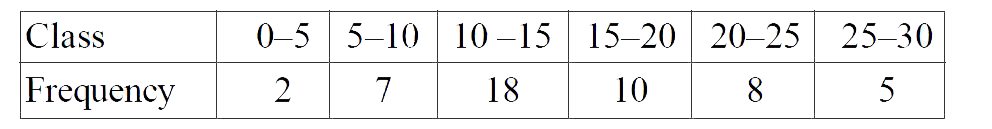
View SolutionFind the mode of the following frequency distribution
View SolutionFind the mode of the following frequency distribution

View Solution
