It has been observed that velocity of ripple waves produced in water (ρ) , and surface tension (T) . Prove that V2∝T/λρ.
The correct Answer is:v2∝Tλρ
According to the problem ,
v∝λaρbTc
v=kλaρbTc
Where k is a dimensionless constant .
LT%(−1)=La(ML−3)b(MT−2)c
rArr M0L1T−1=Mb+cLa−3bT−2c
Using the principle of homogenity , we get
b+c=0,a−3b=1,−2c=−1
Solving these equations , we get
a=−12,b=−12,c=12
So , the relation becomes v=kλ−1/2ρ−1/2T1/2⇒v2∝Tλρ
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Single Correct93videos
DIMENSIONS & MEASUREMENT
- BOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Multiple Correct2videos
DIMENSIONS & MEASUREMENT
- BOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Exercise 1.317videos
DIMENSIONS & MEASUREMENT
- BOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE1videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE1videos
FLUID MECHANICS
Similar Questions
Time period(T) in terms of pressure(P), density(ρ) and surface tension(S) is on basis of dimensional analysis
View SolutionWater is filled up to a height h in a beaker of radius R as shown in the figure. The density of water is ρ, the surface tension of water is T and the atmospheric presure is p0. Consider a vertical section ABCDof the water on one side of this section by water on the other side of this section has magnitude
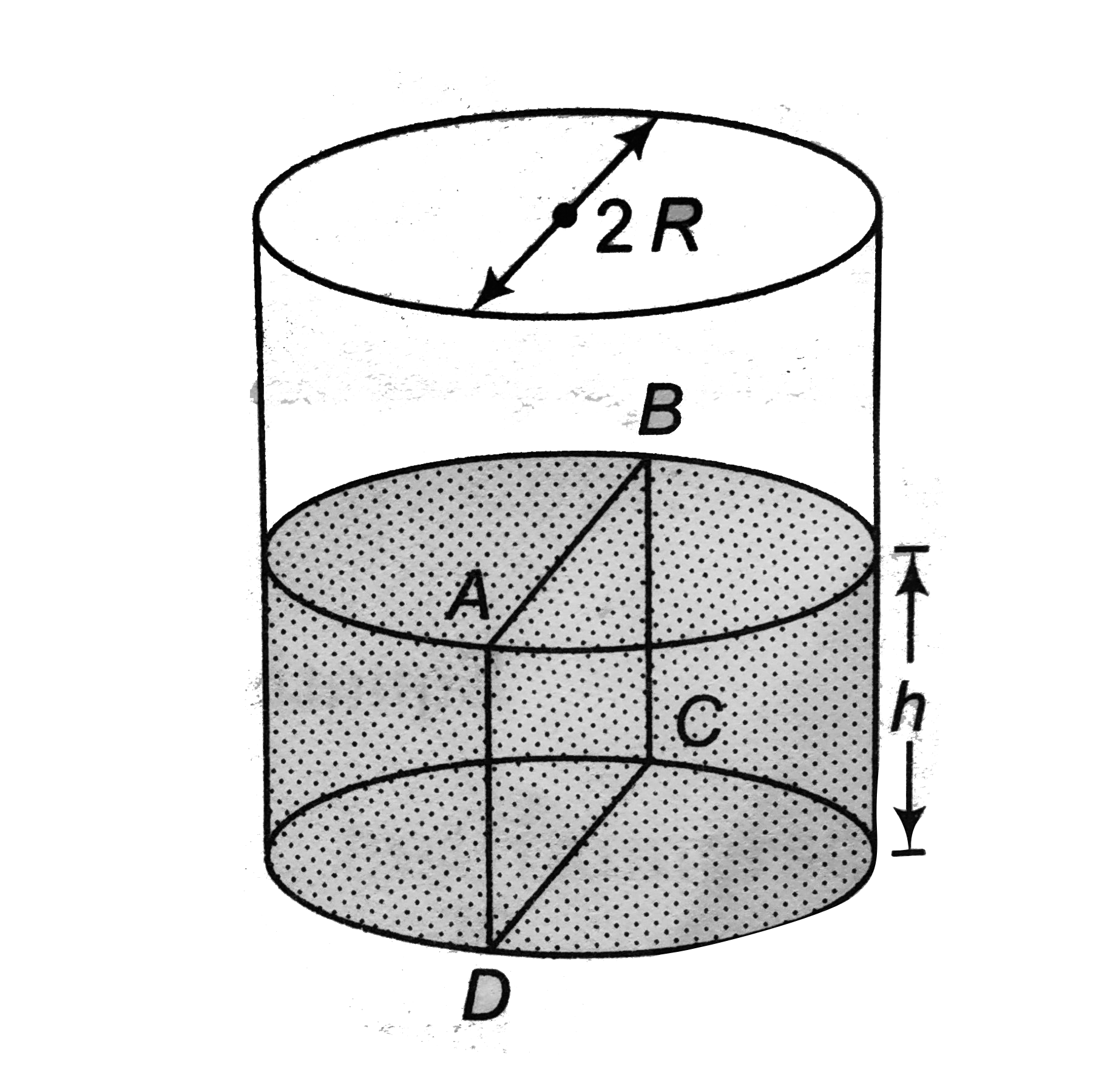
(a) ∣∣2p0Rh+πR2ρgh−2RT∣∣
(b)∣∣2p0Rh+Rρgh2=2RT∣∣
(c ) ∣∣p0(π)R2+Rρgh2−2RT∣∣
(d) ∣∣p0πR2+Rρgh2+2RT∣∣.View Solution
Knowledge Check
- Question 1 - Select One
Frequency is the function of density (ρ) , length (a) and surface tension (T) . Then its value is
Akρ−1/2a−3/2√TBkρ3/2a3/2/√TCkρ12a32/T3/4Dkρ1/2a1/2/T3/2 - Question 2 - Select One
In a string under tension T, the velocity of transverse wave travelling along it is
A∝TB∝√TC∝T2D∝T−1 - Question 3 - Select One
The speed (v) of ripples on the surface of waterdepends on surface tension (σ), density (ϕ) and wavelength (λ). The square of speed (v) is proportional to
AσρλBρσλCλσρDρλσ
The time of oscillation of a small drop of liquid under surface tension depends upon the density ρ, radius r and surface tension S as :
T∝ρaSbrc
find out a, b and c.View SolutionExperiments reveal that the velocity v of water waves may depend on their wavelength λ, density of water ρ, and acceleration due to gravity g. Establish a possible relation between vandλ,g,ρ.
View SolutionThe end of a capillary tube with a radius r is immerseed into water. What ammount of heat will be evolved when the water rises in the tube ? If surface tension of water 'T' density of water =ρ. Given T2ρg=2π.
View SolutionSurface tension of water is T1. When oil spreads on water surface tension becomes T2, then
View SolutionCalculate the pressure inside a small air bubble of radus r situated at a depth h below the free surface of liquids of densities ρ1 and ρ2 and surface tennsions T1 and T2. The thickness of the first and second liquids are h1 and h2 respectively. Take atmosphere pressure =P0.
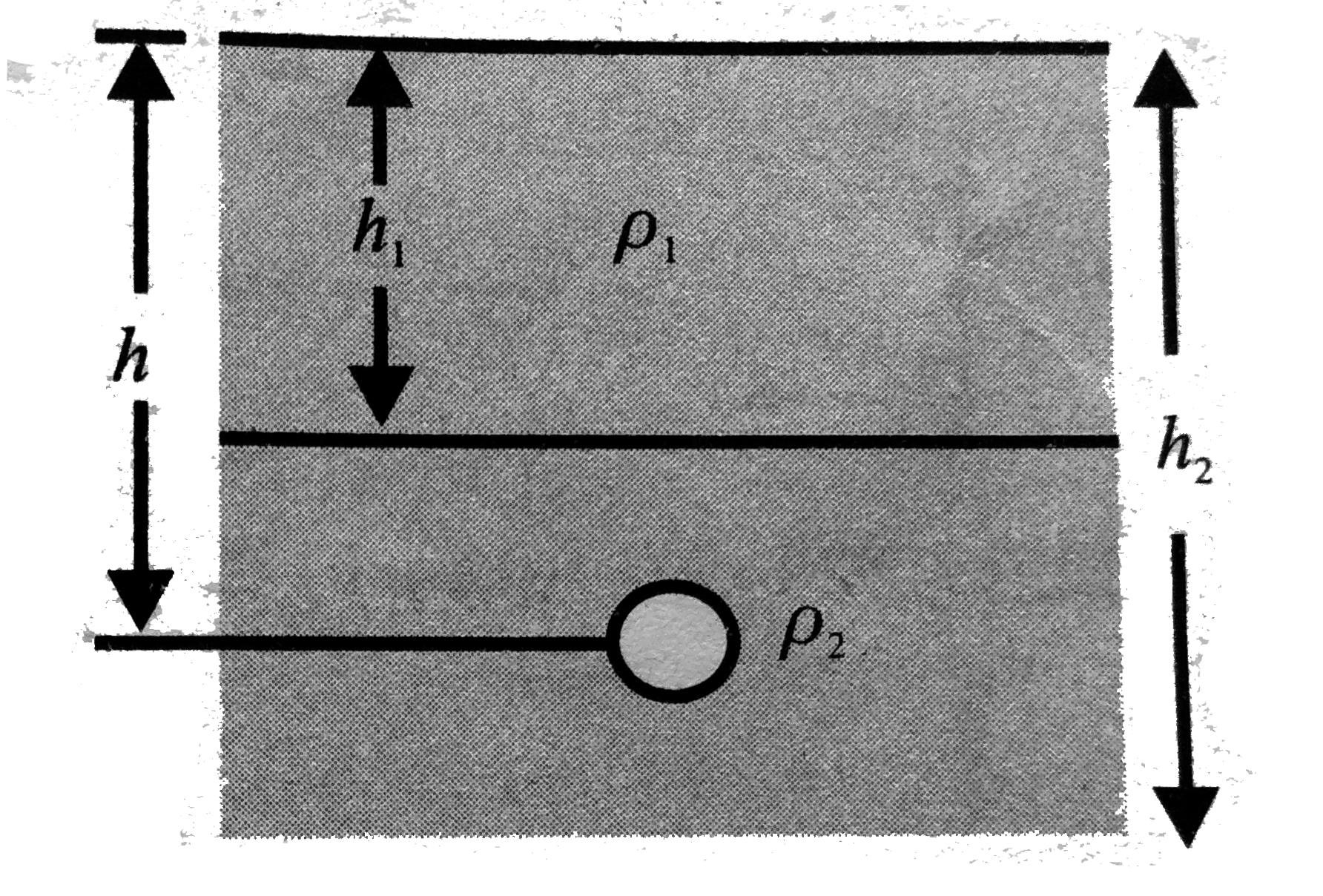 View Solution
View Solution

