A particle moves with a constant acceleration a=2ms−2 along a straight line. If it moves with an initial velocity of 5ms−1, then obtain an expression for its instantaneous velocity.
We know that acceleration is the time rate of change of velocity, i.e. a=dv/dt and differentiation is the inverse operation of integration. So by integrating acceleration, we can obtain the expression of velocity.
So, v=∫adt=2∫dt=2t+c
where c is the constant of integration and its value can be obtained from the initial conditions.
At t=0, v=5ms−1. Putting these in Eq. (i), we have
5=2×0+c
⇒c=5ms−1
Therefore, v=2t+5 is the required expression for the instantaneous velocity.
Topper's Solved these Questions
- BASIC MATHEMATICSBOOK - CENGAGE PHYSICSCHAPTER - BASIC MATHEMATICSEXERCISE - Solved Examples
9 Videos
- BASIC MATHEMATICSBOOK - CENGAGE PHYSICSCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.1
2 Videos
- ARCHIVES 2 VOLUME 6BOOK - CENGAGE PHYSICSCHAPTER - ARCHIVES 2 VOLUME 6EXERCISE - Integer
4 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
Similar Questions
Knowledge Check
A particle starting from a point A and moving with a positive constant acceleration along a straight line reaches another point B in time T. Suppose that the initial velocity of the paticle is u > 0 and P is the midpoint of the line AB. If the velocity of the particle at point P is ν1, and if the velocity at time T/2 is ν2, then
Aν1=ν2Bν1>ν2Cν1<ν2Dν1=12ν2Velocity of a particle at time t=0 is 2ms−1. A constant acceleration of 2ms−2 acts on the particle for 1second at an angle of 60∘ with its initial velocity. Find the magnitude of velocity at the end of 1second.
A√3m/sB2√3m/sC4m/sD8m/sA particle is moving in a straight line and passes through a point O with a velocity of 6ms−1 The particle moves with a constant retardation of 2ms−2 for 4 s and there after moves with constant velocity. How long after leaving O does the particle return to O
A√2tB(2+√2)tCt√2DCan not perdicted unless accelerationis given
A particle starts moving with initial velocity u=25ms−1 and retardation a=−2ms−2. Draw the velocity-time graph.
View SolutionMark the statement true (T) or false (F). For a particle moving with a constant acceleration along a straight line, the velocity- time graph is a straight line.
View SolutionA particle moves in straight line. Acceleration of particle changes with velocity as showns in graph:
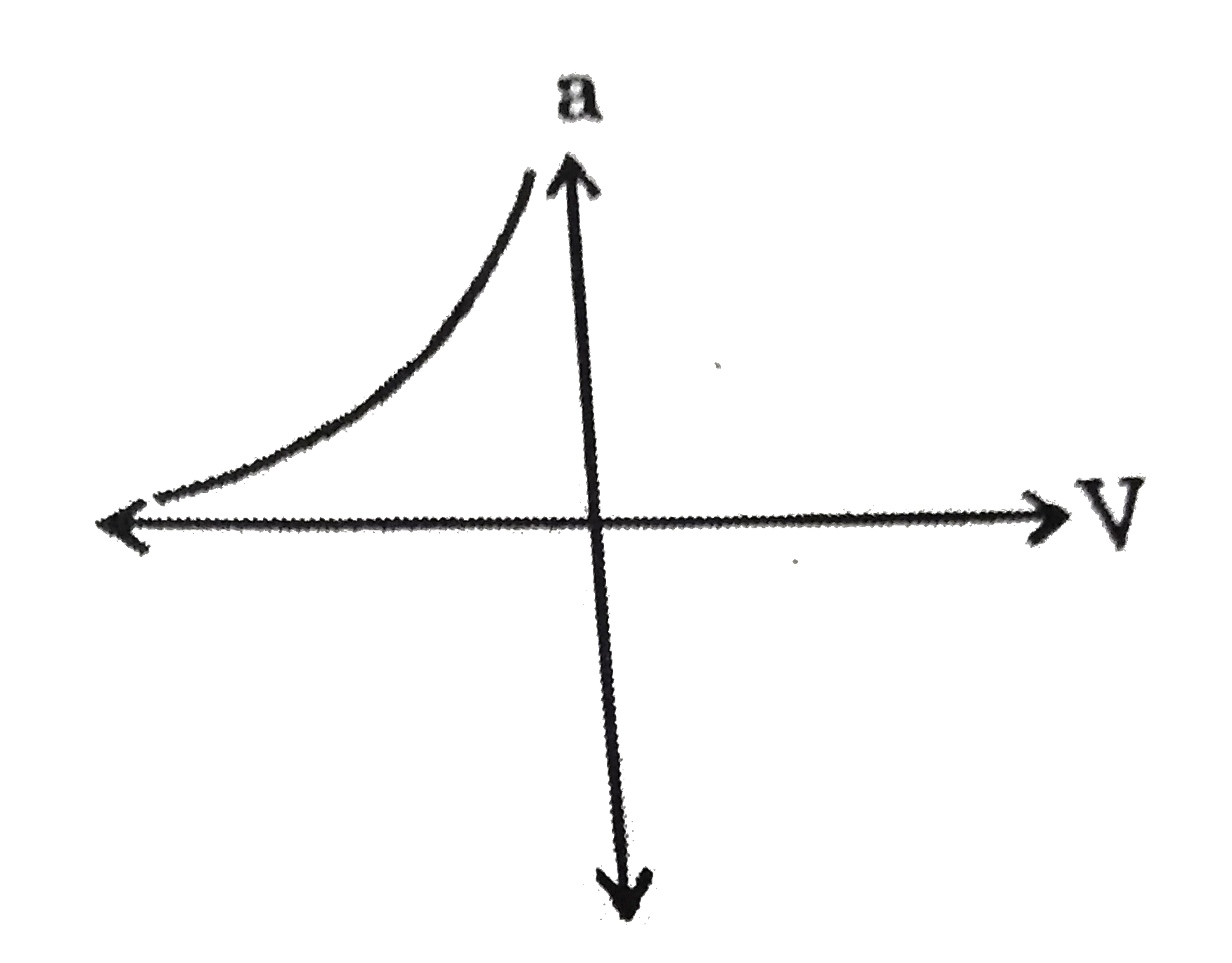
View SolutionA particle is moving on a straight line with constant retardation of 1m/s2. Its initial velocity is 10m/s.
View SolutionA particle is moving in a straight line and passes through a point O with a velocity of 10ms−1. The particle moves with a constannt retardation of 5ms−2 for 3 s and there after moves with constant velocity. How long after leaving O does the particle return to O:-
View Solution
