A particle slides along a track with elevated ends and a flat central part as shown in figure. The flat part has a length l=3m. The curved portions of the track are frictionless. For the flat part, the coefficient of kinetic friction is μk=0.2. The particle is released at point A which is at height h=1.5m above the flat part of the track. Where does the particle finally come to rest?

The particle will finally come to rest on the flat part. Hence, displacement of the particle along vertical is h. If W8 be the work done on the particle by the gravity, then
Wg=mgh(i)
where m is the mass of the particle.
If distance travelled by the particle on the flat part is x, the work done on the particle by the friction is
Wf=−μmgx (ii)
Since, initially, particle was at rest and finally it comes to rest again. Hence, change in its KE is zero.
From work-energy theorem, Wg+Wf=ΔKE
⇒mgh−μmgx=0
⇒x=hμ=1.50.2m⇒x=7.5m
Since x>l, the particle will reach C and then will rise up till the remaining KE at C is converted into potential energy. It will then again descent to C and will have the same kinetic energy as it had when ascending but now will move from C to B. At B, same thing will be repeated (because 7.5<2l), and finally, the particle will stop at E such that
BC+CB+BE=7.5
BE=7.5−6=1.5m
Topper's Solved these Questions
Similar Questions
A particle slides along a track with elevated ends and a flat central part as shown in figure. The flat part has a length l=3m. The curved portions of the track are frictionless. For the flat part, the coefficient of kinetic friction is μk=0.2. The particle is released at point A which is at height h=1.5m above the flat part of the track. Where does the particle finally come to rest?
 View Solution
View SolutionA block slides down a curved frictionless track and then up an inclined plane as in figure. The coefficient of kinetic friction between the block and incline is μk. Find the maximum height reached by the clock.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A block slides down a curved frictionless track and then up an inclined plane as in figure. The coefficient of kinetic friction between the block and incline is μk. Find the maximum height reached by the clock.
 Ah1+μcosθBh1+μtanθCh1+μcotθDhμtanθ
Ah1+μcosθBh1+μtanθCh1+μcotθDhμtanθ - Question 2 - Select One
A particle of mass m slides along a curved-flat-curved track. The curved portions of the track are smooth. If the particle is released at the top of one of the curved portions, the particle comes to rest at flat portion of length l and of μ=μkinetic after covering a distance of
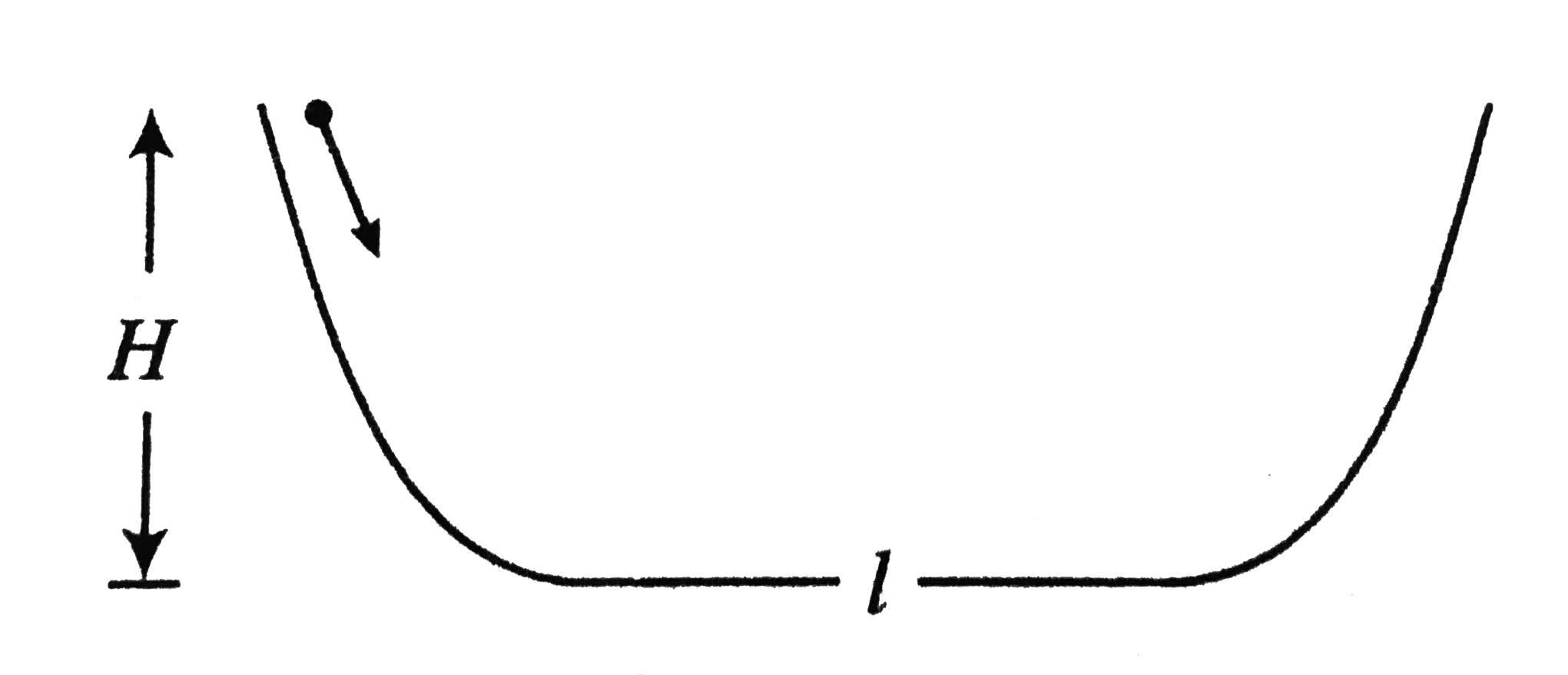 A(a) l3μB(b) H2μkineticC(c) l6D(d) Hμkinetic
A(a) l3μB(b) H2μkineticC(c) l6D(d) Hμkinetic - Question 3 - Select One
A track has two inclined surface AB and DC each of length 3 m and angle of inclination of 30∘ with the horizontal and a central horizontal part of length 4m shown in figure. A block of mass 0.2 kg slides from rest from point A. The inclined surfaces are frictionless. If the coefficient of friction between the block and the horizontal flat surface is 0.2, where will the block finally come to rest ? [in 10−1m]
 A0.5 m away from point BB3.5 m away from point BC0.5 m away from point CD1.5 m away from point C
A0.5 m away from point BB3.5 m away from point BC0.5 m away from point CD1.5 m away from point C
A 0.5kg block slides from the point A on a horizontal track with an initial speed 3m/s towards a weightless horizontal spring of length 1m and force constant 2N/m. The part AB of the track is frictionless and the part BC has the confficient of static and kinetic friction as 0.20 respectively. If the distancences AB and BD are 2m and 2.14m respectively, find total distance through which the block moves before it comes to rest completely. (g=10 m//s^(2) ).
View SolutionA small block slide along a path that is without friction until the block reaches the section l=3m, which begains at height h=3m on a flat incline of angle 37° , as shown. In that section, the coefficient of kinetic friction is 0.50. The block passes through point A with a speed of √136m/s. Find the speed of the block as it passes through point B where the friction ends, in m/s .(take g=10m/s2)
View SolutionA 0.5kg block slides from the point A on a horizontal track with an initial speed 3m/s towards a weightless horizontal spring of length 1m and force constant 2N/m. The part AB of the track is frictionless and the part BC has the coefficient of static and kinetic friction as '0.22' and 0.20 respectively. If the distances AB and BD are 2m and 2.14m respectively, find total distance through which the block moves before it comes to rest completely. (g=10 m//s^(2) ).
View SolutionA 0.5kg block slides from the point A on a horizontal track with an initial speed 3m/s towards a weightless horizontal spring of length 1m and force constant 2N/m. The part AB of the track is frictionless and the part BC has the coefficient of static and kinetic friction as '0.22' and 0.20 respectively. If the distances AB and BD are 2m and 2.14m respectively, find total distance through which the block moves before it comes to rest completely. (g=10 m//s^(2) ).
View SolutionA particle of mass m slides on a frictionaless surface ABCD, starting from rest as shown in figure. The part BCD is a circular arc. If it looses contact at point P, the maximum height attained by the particle from point C is
 View Solution
View Solution

